Alternate Angles
Alternate angles are formed between parallel lines after they are cut by a transversal. They don't have the same vertices and fall on the alternate side of the transversal. In this section, we will discuss the alternate angles, alternate interior angle theorem, and alternate exterior angle theorem along with a few solved examples.
| 1. | Alternate Angles Definition |
| 2. | Types of Alternate Angles |
| 3. | Alternate Angles Theorem |
| 4. | Solved Examples on Alternate Angles |
| 5. | Practice Questions on Alternate Angles |
| 6. | FAQs on Alternate Angles |
Alternate Angles Definition
When two straight lines are cut by a transversal, then the angles formed on the opposite side of the transversal with respect to both the lines are called alternate angles. Hence, it is those angles that have different vertices and lie on the alternate sides of the transversal.

The pairs of alternate angles in the above figure are:
- ∠3 and ∠5
- ∠4 and ∠6
- ∠1 and ∠7
- ∠2 and ∠8
Types of Alternate Angles
There are two types of alternate angles- alternate interior angles and alternate exterior angles. Alternate angles that lie in the interior region of both the lines are called alternate interior angles. Alternate angles that lie in the exterior region of both the lines are called alternate exterior angles.
Example:

In the above image, two pairs of alternate interior angles are ∠3 & ∠5 and ∠4 & ∠6. Two pairs of alternate exterior angles are ∠1 & ∠7, and ∠2 & ∠8.
Alternate Angles Theorem
Now, let us learn about the two theorems related to Alternate Angles.
- Alternate Interior Angle Theorem
- Alternate Exterior Angle Theorem
Alternate Interior Angle Theorem
Suppose two parallel lines are intersected by a transversal, as shown below:
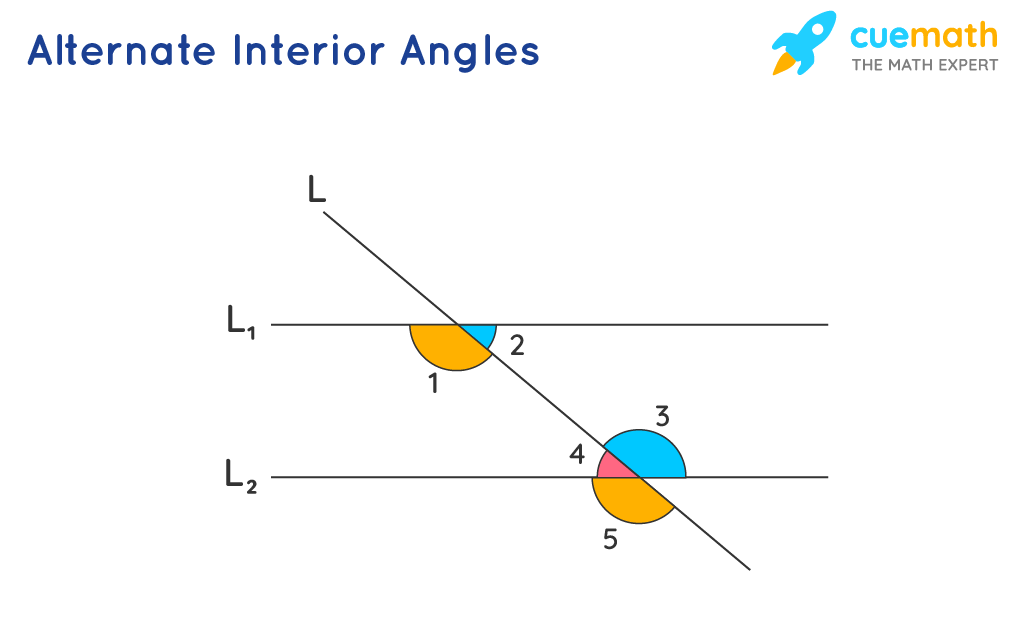
What is the relation between any pair of alternate interior angles? This relation is determined by the "Alternate Interior Angle Theorem." When a transversal intersects two parallel lines, each pair of alternate interior angles are equal. By alternate interior angle theorem converse, if a transversal intersects two lines such that a pair of interior angles are equal, then the two lines are parallel.
Alternate Interior Angle Theorem Proof
Refer to the figure above. We have:
∠1= ∠5 (corresponding angles)
∠3= ∠5 (vertically opposite angles)
Thus, ∠1= ∠3
Similarly, we can prove that ∠2 = ∠4
Alternate Exterior Angle Theorem
If two lines are parallel, then the pair alternate exterior angles formed are congruent.
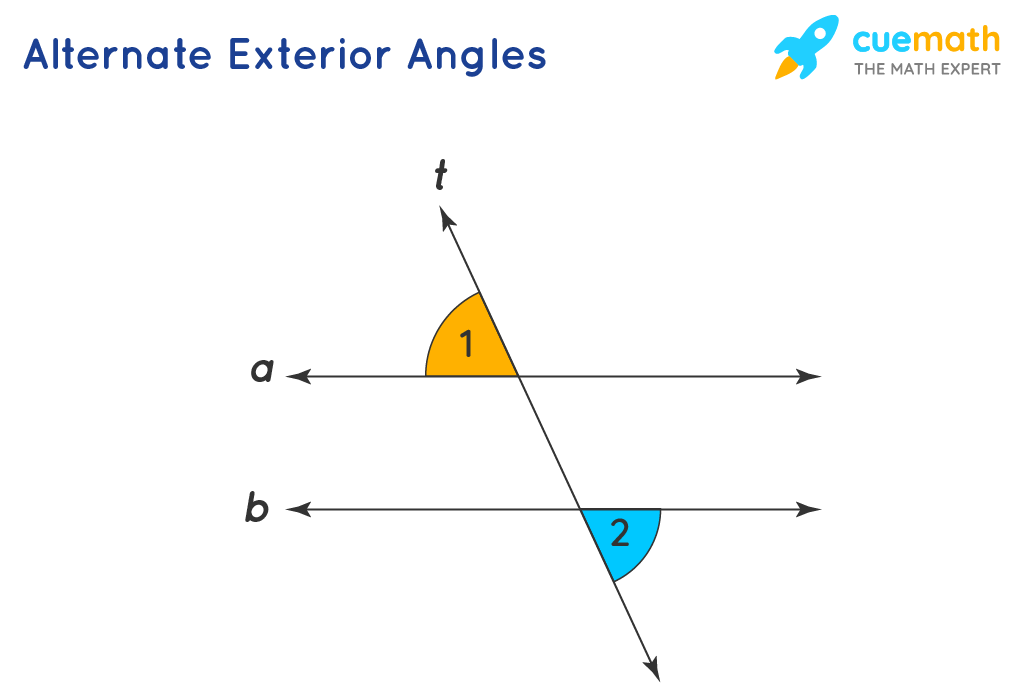
In the figure above, we can observe that angles 1 and 2 is a pair of alternate exterior angle. We will now prove that they are congruent (i.e. they have equal measure).
Alternate Exterior Angle Theorem Proof
To prove this result, we will consider the vertically opposite angle of ∠1. Let's denote it by ∠3.

Now,
∠1=∠3 as they are vertically opposite angles.

Since line a || line b
∠3 = ∠2 (corresponding angles axiom)
∴ ∠1 = ∠2 (transitivity)
- Alternate Angles can be formed with two non-parallel lines also, but there is no relation between the angles so formed.
- Alternate Interior Angles are popularly known as Z-angles because we can easily identify a pair of alternate interior angles in a Z-shaped figure.
Related Topics:
Alternate Angles Examples
-
Example 1: Two roads are running parallel to each other as shown below.

If a = (2x)∘ and b = (30−4x)∘, then what will be the value of x?
Solution:
To solve this problem, we will be using the alternate exterior angle theorem.
The pair of angles in this figure forms a pair of alternate exterior angles.
So, we have:
(2x)º = (30−4x)º
2x+4x = 30
6x = 30
x = 5
Therefore, the value of x is 5.
-
Example 2: In the given figure, find the value of p.

Solution:
In the given figure, ∠ADE and ∠FGJ form a pair of alternate exterior angles.
By using alternate exterior angle theorem, we have, ∠ADE=∠FGJ
So, pº +55° = 135º
pº =135º−55º
p° = 80°Therefore, the value of p is 80º.
-
Example 3: Find x, if line p || line q.

Solution:
Given that, p||q. So by alternate exterior angle theorem we get,
(2x+26)∘ = (3x−33)∘
2x−3x = −33−26
−x = −59
x = 59
Therefore, the value of x is 59.

FAQs on Alternate Angles
What are Alternate Interior Angles?
Alternate interior angles lie on the interior side of the parallel lines and are on the opposite side of the transversal. While the angles that lie on the exterior of the parallel lines are not considered as the alternate interior angles.
What are Alternate Exterior Angles?
Alternate exterior angles lie on the exterior side of the parallel lines and are on the opposite side of the transversal. While the angles that lie on the interior of the parallel lines are not considered as the alternate exterior angles.
Do Alternate Angles Add up to 180?
Alternate angles generally don't add up to 180 degrees because they are not supplementary angles, but they can add up to 180 degrees if the transversal is perpendicular to the parallel lines. They become supplementary angles if the transversal is perpendicular to the parallel lines, hence it will make every angle equal to 90 degrees, so any two angles become supplementary angles.
How Many Types of Alternate Angles are There?
There are two types of alternate angles:
- Alternate interior angles
- Alternate exterior angles
Do Alternate Interior Angles Equal Each Other?
Yes, when a transversal meets two or more parallel lines, alternate interior angles formed are equal to each other. The angles are formed on the opposite sides of the transversal and in the interior of two lines. When a transversal meets two or more parallel lines, alternate interior angles formed are not supplementary angles. The measure of all pairs of alternate angles are equal.
What is the Converse of Alternate Interior Angles Theorem?
The converse of alternate interior angles is that "if a transversal intersects two lines such that a pair of interior angles are equal, then the two lines are parallel."
What is the Converse of Alternate Exterior Angles Theorem?
The converse of alternate exterior angles is that "if a transversal intersects two lines such that a pair of exterior angles are equal, then the two lines are parallel."
What is the Alternate Angle of Angle?
Alternate angles are obtained on the opposite sides of a transversal line that have the same size. These angles are equal and are of two different types, namely alternate interior angles and alternate exterior angles. Usually, interior alternate angles can be identified when a Z-like shape is drawn.
visual curriculum
