Acute Triangle

An acute triangle is a trigon with three sides and three angles each less than 90º. The measurement of all the three interior angles of the acute triangle lies within 0° to 90°, but the sum of all the interior angles is always 180 degrees. Triangle can be classified on the basis of angles and sides. An acute triangle is one that is classified on the basis of the measurement of angles.

As we know that triangles can be classified on the basis of sides and angles. The acute triangle can also be further classified as:

• Equilateral Acute Triangle: It is also known as an equiangular triangle because all the three interior angles of an equilateral acute triangle measure 60°.
• Isosceles Acute Triangle: In this triangle, two sides and two angles always measure the same.
• Scalene Acute Triangle: In this triangle, all three sides and internal angles are unequal. All the interior angles measure less than 90 degrees.

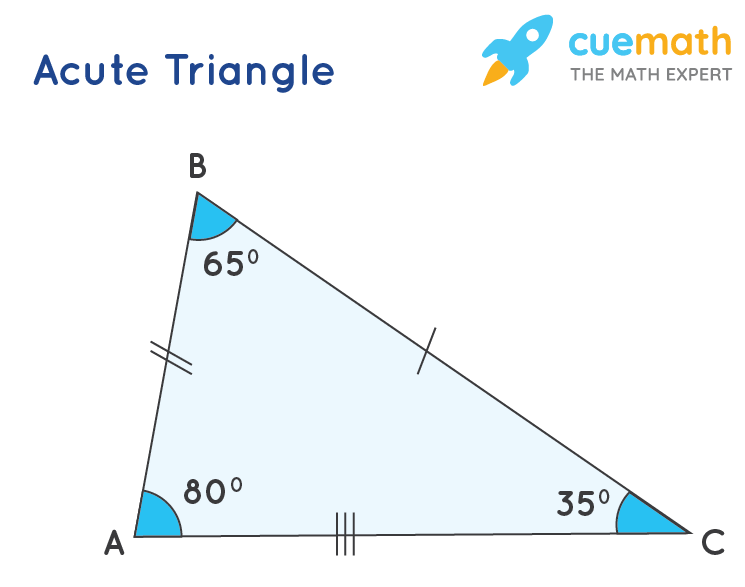
The above image is an example of the scalene acute triangle representing 3 unequal sides and unequal angles. But the value of all three angles is less than 90 degrees and they add up to 180 degrees.

There are few important properties that make the acute triangle different from other types of triangles.

• According to the angle sum property, all the three interior angles of an acute triangle add up to 180°.
• A triangle cannot be a right-angled triangle and an acute-angled triangle at the same time.
• A triangle cannot be an acute-angled triangle and an obtuse-angled triangle at the same time.
• The angle property of the acute triangle says the interior angles of an acute triangle are always less than 90° or lies between (0° to 90°).

There are two basic formulas of an acute triangle, which are given below;

• Area of an acute triangle
• The perimeter of the acute triangle

Let us learn about these two formulas of an acute triangle in detail.

Area of Acute Triangle

The area of an acute triangle is given as Area = (1/2) × b × h square units. Here, "b" denotes the base, and "h" denotes the height of an acute triangle.

Note: If all the sides of the acute triangle are given then the area of an acute triangle can be easily calculated using Heron's formula given below.

Area of an acute triangle using heron's formula = \(\sqrt{S(S-a)(S-b)(S-c)}\) square units. Here, S denotes the semi perimeter which can be calculated as S = (a + b + c)/2, and a, b, and c are the sides of the given triangle.

Perimeter of Acute Triangle

The perimeter of an acute triangle is defined as the sum of the three sides and it is given as, P = (a + b + c) units. Here, a, b, and c are the sides of the acute triangle. It gives the total length required to form an acute-angled triangle. We use the perimeter to draw or make an acute triangle with a rope, thread, pencil, etc.

Related Articles on Acute Triangle

Check out the interesting articles linked below to learn more about terminologies related to the acute triangle.

• Types of Triangles
• Triangles
• Acute Angles

Are Isosceles Triangles Always Acute Triangle?

No, an isosceles triangle can be acute, right, or obtuse-angled depending upon the measure of the angles it has. To be an acute triangle, all three interior angles should measure less than 90 degrees.

How do you know if a Triangle is Acute Triangle?

A triangle can be acute if all the angles inside it are measuring less than 90°. All angles should be measured between 0 degrees to 90 degrees.

What are the Types of an Acute Triangle?

There are three types of acute triangles mentioned below:

• Equilateral Acute Triangle: All three angles are equal to 60° and all sides should be equal.
• Isosceles Acute Triangle: Two sides and two angles equal, and all angles less than 90 degrees.
• Scalene Acute Triangle: Three unequal sides and three unequal interior angles. All the interior angles measure less than 90 degrees.

Do Angles of Acute Triangles Add up to 180?

The angles of any triangle add up to 180 degrees. An acute triangle is a type of triangle hence sum of its interior angle sum up to 180 degrees, where each individual angle measures less than 90 degrees.

Can a Triangle be Right and Acute?

No, a triangle can either be acute or be right-angled. It cannot be both at the same time. If the value of any one angle of triangle crosses 90 degrees then it is no more considered an acute triangle.

How to Find the Area of an Acute Triangle?

To find the area of an acute triangle we will follow the given steps:

• Note down the given dimensions of an acute triangle, i.e., base length (in units) and altitude (in units).
• Now substitute the dimensions in the area of an acute triangle formula which is given as Area = (1/2) × b × h square units.
• Write the answer in terms of square units (units²).
• In the case where all sides are given, we can use heron's formula for calculating the acute triangle's area (A = \(\sqrt{S(S-a)(S-b)(S-c)}\) square units).

What does an Acute Triangle Look Like?

An acute triangle has a measure of its angle always between 0 degrees to 90 degrees. The measure of its three sides can be equal, unequal, or any two sides equal. When an acute triangle has three angles measure less than 90 degrees with all three equal sides it is termed as an equilateral acute triangle. If an acute triangle has three angles less than 90 degrees with two equal sides it is known as an isosceles acute triangle. If an acute triangle has three angles less than 90 degrees with all three sides unequal it is known as a scalene acute triangle. All the vertices of the acute triangle are pointed outwards, so it is a convex 2D shape.