Trigonometric Table
A trigonometric table is a table that lists the values of the trigonometric functions for various standard angles such as 0°, 30°, 45°, 60°, and 90°. Trigonometric table comprises trigonometric ratios – sine, cosine, tangent, cosecant, secant, cotangent. These ratios, in short, are written as sin, cos, tan, cosec, sec, and cot. The values of trigonometric ratios of standard angles in a trig table are essential in solving trigonometry problems.
Trigonometry table is useful for solving a variety of problems in mathematics and physics, including finding the solutions of triangles. Also, it helps in determining the values of periodic functions and solving differential equations.
| 1. | What is Trigonometric Table? |
| 2. | Trigonometric Values |
| 3. | Trigonometry Table for Standard Angles |
| 4. | How to Learn Trigonometric Table? |
| 5. | Trigonometry Table in Radians |
| 6. | FAQs on Trigonometric Table |
What is Trigonometric Table?
The trigonometric table is simply a collection of the values of trigonometric ratios for various standard angles including 0°, 30°, 45°, 60°, 90°, sometimes with other angles like 180°, 270°, and 360° included, in a tabular format. Because of patterns existing within trigonometric ratios and even between angles, it is easy to both predict the values of the trigonometry table and use the table as a reference to calculate trigonometric values for various other angles. The trigonometric functions are namely the sine function, cosine function, tan function, cot function, sec function, and cosec function.
Here is the trigonometry table for standard angles along with some non-standard angles:
| Trigonometry Table | ||||||||
|---|---|---|---|---|---|---|---|---|
| θ | 0° (0 radians) |
30° (π/6) |
45° (π/4) |
60° (π/3) |
90° (π/2) |
180° (π) |
270° (3π/2) |
360° (2π) |
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
| csc | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
| sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1 |
| cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
Note: Here, 1/√2 can also be written as √2/2 and 1/√3 can also be written as √3/3 (by rationalizing the denominators).
Trigonometric Values
Trigonometry deals with the relationship between the sides of a triangle (right-angled triangle) and its angles. The trigonometric value is a collective term for values of different ratios, such as sine, cosine, tangent, secant, cotangent, and cosecant in a trigonometric table. All the trigonometric ratios are related to the sides of a right-angle triangle and their values are found using the following ratios.

To remember this easily remember the word "SOHCAHTOA"!
- SOH
Sine = Opposite / Hypotenuse
- CAH
Cosine = Adjacent / Hypotenuse
- TOA
Tangent = Opposite / Adjacent
Standard Angles in a Trigonometric Table
The values of trigonometric ratios for the angles 0°, 30°, 45°, 60°, and 90° in a trigonometry table are commonly used to solve trigonometry problems. These values are associated with the measurement of the lengths and the angles of a right-angle triangle. Thus, 0°, 30°, 45°, 60°, and 90° are called the standard angles in trigonometry.
Trigonometry Table for Standard Angles
The trigonometric table is simply a collection of values of trigonometric functions of various standard angles including 0°, 30°, 45°, 60°, 90°, along with with other angles like 180°, 270°, and 360° included, in a tabular format. It is easy to predict the values of the trig ratios in a trigonometric table and to use the table as a reference to calculate trigonometric values for various other angles, due to the patterns existing within trigonometric ratios and even between angles.
The table consists of trigonometric ratios – sine, cosine, tangent, cosecant, secant, and cotangent. In short, these ratios are written as sin, cos, tan, cosec, sec, and cot. It is best to remember the values of the trigonometric ratios of these standard angles.
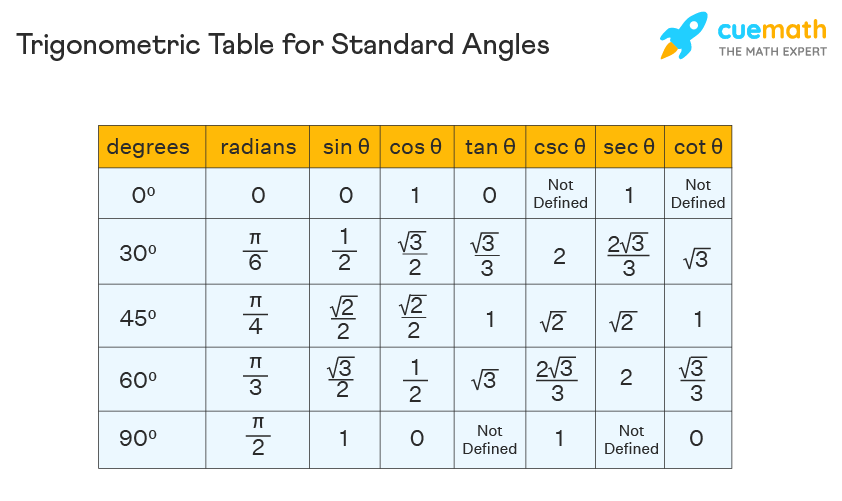
A few key points that can be noted in the trigonometric table are,
- The values for complementary angles present like 30° and 60° in a trigonometric values table can be computed using complementary formulas for the various trigonometric ratios.
- The value for some ratios in a trig table is given as ∞ or "not defined". The reason for this is that while computing the values, a "0" appears in their denominator, so the value becomes undefined and is said to be equivalent to infinity.
- There is a sign change in the values in various places under 180°, 270°, etc for values of some trig ratios in a trigonometric table. This is due to the change in the quadrant.
How to Learn Trigonometric Table?
The trigonometric table might seem complex at first, but it can be learned easily by only the values of sine for the 8 standard angles. Before generating the table, there are few formulas that must be followed as given below:
- tan x = sin x/cos x
- cosec x = 1/sin x
- sec x = 1/cos x
- cot x = 1/tan x
Given below are the steps to create and remember a trigonometric table.
- Step 1: Create a table with the top row listing the angles such as 0°, 30°, 45°, 60°, 90°, 180°, 270° and 360°and write all trigonometric functions in the first column such as sin, cos, tan, cosec, sec, cot.
- Step 2: Determining the value of sin: Write the angles 0°, 30°, 45°, 60°, and 90° in ascending order. The values of sin for these angles are 0, 1/2, 1/√2, √3/2, and 1 respectively. This gives the values of sine for these 5 angles. Now for the remaining three use:
sin (180° − x) = sin x
sin (180° + x) = -sin x
sin (360° − x) = -sin x
This means,
sin 180° = sin (180° − 0º) = sin 0º = 0
sin 270° = sin (180° + 90º) = -sin 90º = -1
sin 360° = sin (360° − 0º) = -sin 0º = 0
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
|---|---|---|---|---|---|---|---|---|
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
- Step 3: Determining the value of cos: sin (90° – x) = cos x. Use this formula to compute values for cos x. For example, cos 45° = sin (90° – 45°) = sin 45°. Similarly, cos 30° = sin (90° - 30°) = sin 60°. Using this, you can easily find out the value of cos function as,
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
|---|---|---|---|---|---|---|---|---|
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
- Step 4: Determining the value of tan: (tan x = sin x/cos x). Hence, the value of tan function can be generated as,
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
|---|---|---|---|---|---|---|---|---|
| tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
- Step 5: Determining the value of cot: (cot x = 1/tan x). Use the relation to generate the cot function as,
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
|---|---|---|---|---|---|---|---|---|
| cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
- Step 6: Determining the value of cosec: (cosec x = 1/sin x)
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
| cosec | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
- Step 7: Determining the value of sec: (sec x = 1/cos x)
| Angles(in Degrees) | 0° | 30° | 45° | 60° | 90° | 180° | 270° | 360° |
|---|---|---|---|---|---|---|---|---|
| sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1 |
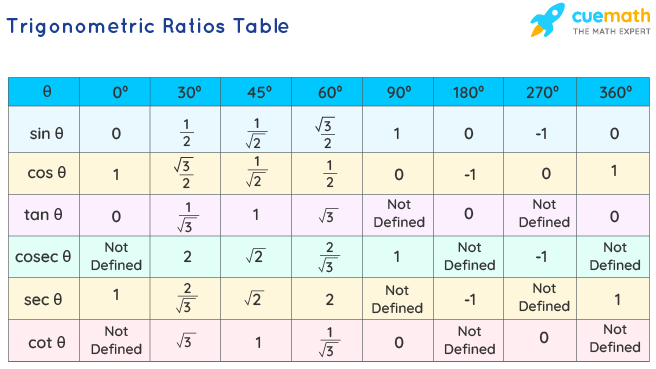
The following trigonometric table covers the value of trigonometric ratios for all basic angles ranging from 0º to 360º.
Trick to Remember Trigonometric Table
Let us learn the one-hand trick for remembering the trigonometric table easily! Designate each finger the standard angles as shown in the image. For filling the sine values in the trigonometry table, we will include counting of the fingers, while for the cos table we will simply fill the values in reverse order.

- Step 1: For the sine table, count the fingers on the left side for the standard angle.
- Step 2: Divide the result of above step (number of fingers on the left side) by 4
- Step 3: Take the square root of the ratio.
Example 1: For sin 0°, there are no fingers on the left-hand side, therefore we will take 0. Dividing the zero by 4 we get 0. Taking the square root of the ratio we would get the value of sin 0° = 0
Example 2: For sin 60°, there are 3 fingers on the left-hand side. Dividing 3 by 4 we get (3/4). Taking the square root of the ratio √(3/4) we would get the value of sin 60° = √3/2.
Similarly, we can find out values for sin 30°, 45°, and 90° to fill the table.
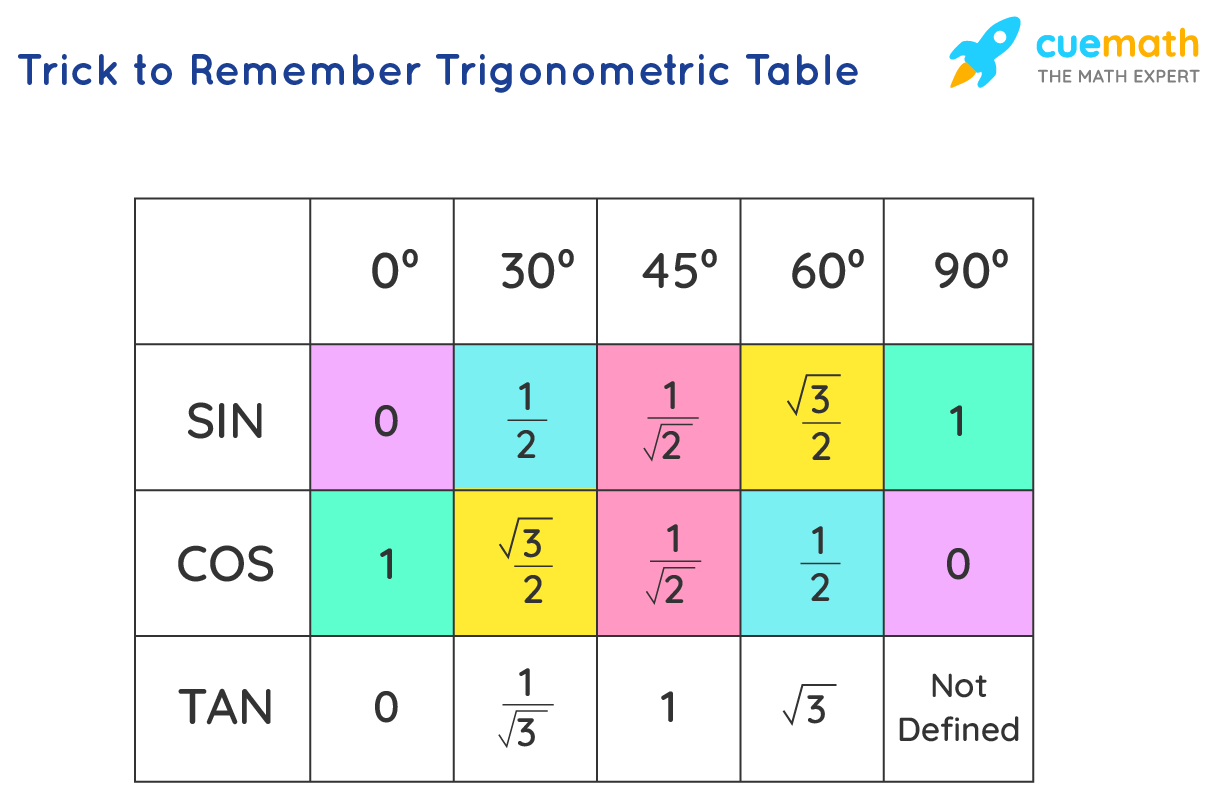
In order to complete the cos table, simply fill in the values of sine in a reverse manner. For tan values, create the ratios by taking numerators of sine and cos values of the corresponding angle.
Given below is a list of trigonometric formulas that would help you memorize the trigonometric table, based on the relationship between different trigonometric ratios.
sin x = cos (90° – x)
- cos x = sin (90° – x)
- tan x = cot (90° – x)
- cot x = tan (90° – x)
- sec x = cosec (90° – x)
- cosec x = sec (90° – x)
- 1/sin x = cosec x
- 1/cos x = sec x
- tan x = sin x/cos x
- 1/tan x = cot x
Trigonometry Table in Radians
The trigonometric table for standard angles below presents the values of trigonometric ratios for the measure of angles given in radians.

To know how each of these is derived, check out the following links:
- sin 0, cos 0, tan 0
- sin π/6, cos π/6, tan π/6
- sin π/4, cos π/4, tan π/4
- sin π/3, cos π/3, tan π/3
- sin π/2, cos π/2, tan π/2
Important Notes on Trigonometry Table:
- Trigonometric values are based on the three major trigonometric ratios: Sine, Cosine, and Tangent.
- Sine or sin θ = Side opposite to θ / Hypotenuse
- Cosines or cos θ = Adjacent side to θ / Hypotenuse
- Tangent or tan θ = Side opposite to θ / Adjacent side to θ
- 0°, 30°, 45°, 60°, and 90° in a trigonometric table are the standard angles in trigonometry.
- The angle values of trigonometric functions cotangent, secant, and cosecant in a trigonometric table, can also be calculated using these standard angles values of sine, cosecant, tangent.
- All the higher angle values of trigonometric functions such as 120°, 390°, .... can be easily calculated from the standard angle values in a trigonometric values table.
☛ Related Topics:
Examples using Trigonometric Table
-
Example 1: Find out the exact value of sin15º using the trig value for standard angles from a trigonometry table.
Solution:
Using the trigonometric values table, we know that sin 45º = 1/√2, cos 30º = (√3/2), cos 45º = 1/√2, and sin 30º = 1/2
sin 15º = sin(45º - 30º) = sin45º cos 30º - cos 45ºsin 30º = (√2/2) • (√3/2) - (√2/2) • (1/2) = (√6 - √2)/4 = (√3 - 1)/2√2
Answer: The value of sin15º = (√3 - 1)/2√2
-
Example 2: Can you determine the height of the glacier given below using a trigonometric table? Use of calculators is permitted.

Solution:
The length of the hypotenuse is known to be 20 units. We want to find out the opposite side to the angle. Sine ratio uses the opposite side and hypotenuse. Remember the word "SOHCAHTOA"! SOH: Sine = Opposite / Hypotenuse.
Thus, sin45º = Opposite/Hypotenuse, which gives 1/√2 = Opposite/20.
Hence, Opposite = 20/√2, Opposite ≈ 14.14.
Answer: Hence, the height of the glacier is approx. 14.14 units.

FAQs on Trigonometric Table
What is the Trigonometry Table?
Trigonometric table gives the values of trigonometric functions for the standard angles such as 0°, 30°, 45°, 60°, and 90°, in a tabulated manner.
| θ | 0° | 30° | 45° | 60° | 90° |
|---|---|---|---|---|---|
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 |
| tan | 0 | 1/√3 | 1 | √3 | ∞ |
| csc | ∞ | 2 | √2 | 2/√3 | 1 |
| sec | 1 | 2/√3 | √2 | 2 | ∞ |
| cot | ∞ | √3 | 1 | 1/√3 | 0 |
This table is also known as sin cos tan table.
What is Trigonometry Table Formula?
In trig table, if the first row (of sine) is written, writing the other rows is pretty simple. For the angles 0°, 30°, 45°, 60°, and 90°, write the row of sine using the following formula/trick: √0/2, √1/2 √2/2, √3/2, and √4/2. These would correspond to the values 0, 1/2, 1/√2, √3/2, and 1. Then we can write the cos row by reversing these values. The values of other trig ratios can be written using trigonometric identities.
What are the Standard Angles in a Trigonometric Ratio Table?
The angles 0°, 30°, 45°, 60°, and 90° in a trigonometry ratio table are called standard angles, and the trigonometric values for these standard angles are commonly used to solve the trigonometry problems. These values are associated with the measurement of lengths and angles of the right-angle triangle.
What Does the Infinity Value of the Trigonometric Ratio in the Trigonometry Table Mean?
Undefined values in a trigonometric table are often given an infinity (∞) value because the value is such large that there is no definite value to assign at this point.
How to Find the Value of Trigonometric Functions Given in Trigonometric Table?
All the trigonometric functions in a trigonometric ratio table are related to the ratios of sides of the triangle and their values can be easily found by using the following relations:
- sinθ = Opposite/Hypotenuse
- cosθ = Adjacent/Hypotenuse
- tanθ = Opposite/Adjacent
- cotθ = 1/tanθ = Adjacent/Opposite
- cosecθ = 1/sinθ = Hypotenuse/Opposite
- secθ = 1/cosθ = Hypotenuse/Adjacent
What are the Six Basic Trigonometric Functions in Trigonometric Table?
The six basic trigonometric functions in the trigonometric table are Sine, Cosine, Tangent, Secant, Cotangent, and Cosecant function.
How Does a Trigonometric Table Work?
The trigonometric ratios table helps us to find the values of trig ratios for standard angles such as 0°, 30°, 45°, 60°, and 90°. It comprises trigonometric ratios of sine, cosine, tangent, cosecant, secant, cotangent. These ratios are written in short as sin, cos, tan, cosec, sec, and cot.
Who Invented Trigonometry Table?
The Greek astronomer Hipparchus (127 BC) is believed to be the first to make a trigonometry value chart (based on the chords in a circle).
What is the Easiest Way to Learn Trigonometric Tables?
To learn the one-hand trick for remembering the trigonometric table easily, designate each finger the standard angles.
- For filling the sine row write the values 0, 1/2, 1/√2, √3/2, and 1 in order.
- For the cos row, we will simply fill the values in reverse order.
- For the row of tan, divide the corresponding values of sin by cos.
- For other trig ratios, just apply reciprocal identities.
What is the Use of a Trigonometry Table?
A trigonometry ratio table is used to find the value of any trigonometric ratio for a given angle. The basic values of trig ratios for standard angles given in a trigonometry table can be used in the calculation of trigonometric ratios of other angles. These values find application in fields like engineering, architecture, etc.
visual curriculum
