Difference of Cubes Formula

The difference of cubes formula is used to find the difference of cubes of two numbers without actually calculating the cubes. It is one of the algebraic identities. The difference of cubes formula is used to factorize the binomials of cubes. The difference of cubes formula is also known as a³ - b³ formula. The difference of cubes formula is explained below along with solved examples in the following section.

What Is the Difference of Cubes Formula? 

The difference of cubes formula or a³ - b³ formula can be verified, by multiplying (a - b) (a² + ab + b²) and see whether you get a³ - b³.The difference of cubes formula is given as,

Difference of Cubes Formula

a³ - b³ = (a - b) (a² + ab + b²)

You can remember these signs using the following trick.

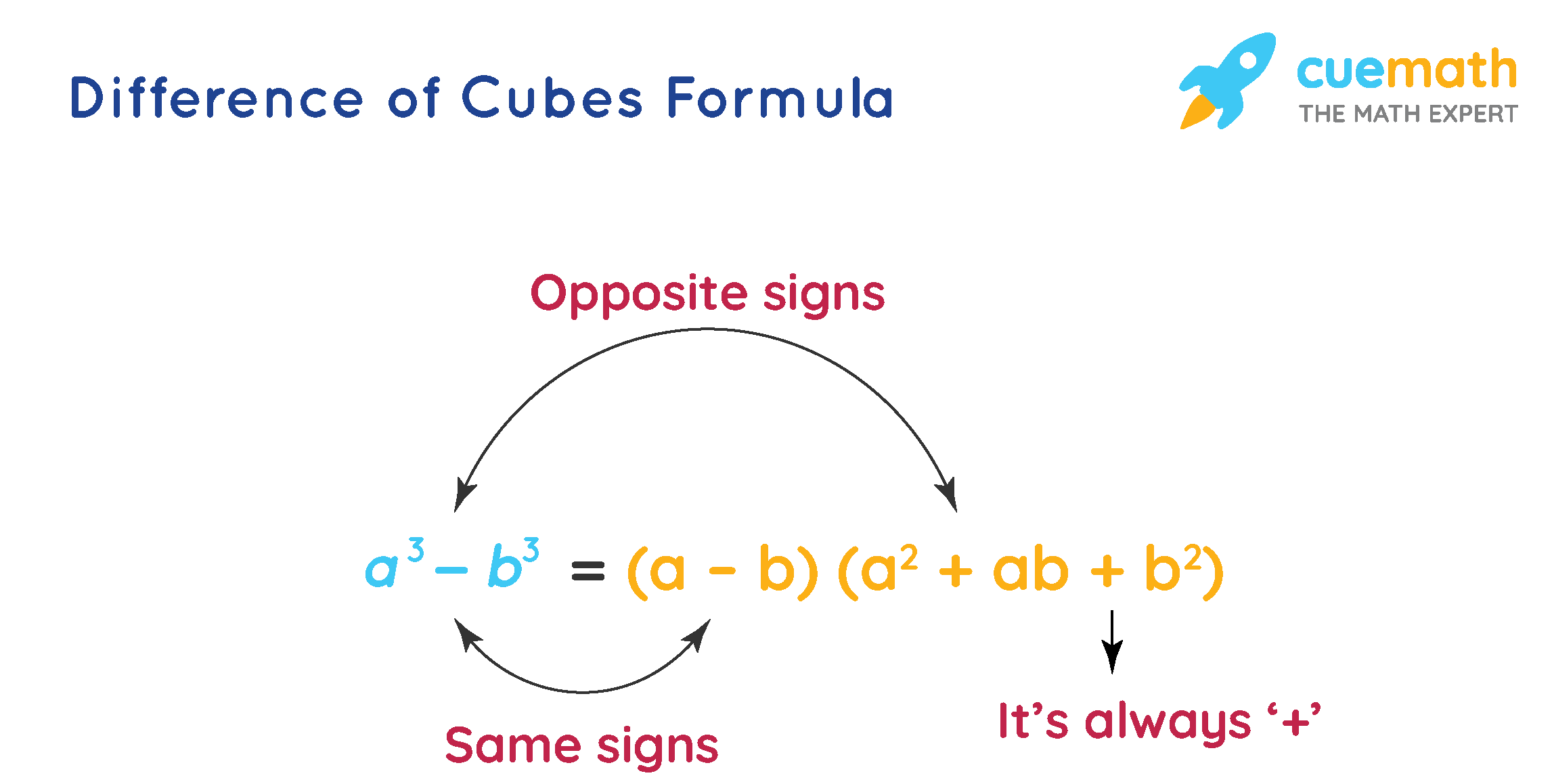
Examples Using Difference of Cubes Formula

Example 1: Find the value of 108³ - 8³ by using the difference of cubes formula.

Solution:

To find: 108³ - 8³.

Let us assume that a = 108 and b = 8.

We will substitute these in the formula of difference of cubes. 

a³ - b³ = (a - b) (a² + ab + b²)

108³−8³ = (108 − 8)(108² + (108)(8) + 8²) = (100)(11664 + 864 + 64) = (100)(12592) = 1259200

Answer: 108³ - 8³ = 1,259,200.

Example 2: Factorize the expression 27x³ - 125 by using the difference of cubes formula.

Solution:

To factorize: 27x³ - 125.

We will use the difference of cubes formula to factorize this.

We can write the given expression as

27x³ - 125 = (3x)³ - 5³

We will substitute a = 3x and b = 5 in the formula of the difference of cubes. 

a³ - b³ = (a - b) (a² + ab + b²)

(3x)³ − 5³ = (3x − 5)((3x)² + (3x)(5) + 5²) = (3x − 5)(9x² + 15x + 25)

Answer: 27x³ - 125 = (3x - 5)(9x² + 15x + 25).