A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
LearnPracticeDownload

Quartile Deviation

Quartile deviation is a statistic that measures the deviation in the middle of the data. Quartile deviation is also referred to as the semi interquartile range and is half of the difference between the third quartile and the first quartile value. The formula for quartile deviation of the data is Q.D = (Q3 - Q1)/2.

Let us learn more about quartile deviation, steps to find quartile deviation for grouped and ungrouped data, and also check the solved examples, FAQs.

1. What Is Quartile Deviation?
2. How to Find Quartile Deviation?
3. Examples on Quartile Deviation
4. Practice Questions
5. FAQs on Quartile Deviation

What Is Quartile Deviation?

Quartile deviation is a statistic that measures the deviation. It measures the deviation of the data from the average value. Here quartile deviation gives the spread of the data, which helps to understand the distribution of the data. Before understanding more about quartile deviation let us understand more about quartiles. Here we have three quartiles Q1, Q2, Q3 which divide the data into three quarters. The median of the data has been referred as the second quartile Q2. Also, the first quartile Q1 is the median of the first half of the data, and the third quartile Q3 is the median of the second half of the data.

Quartile deviation is the dispersion in the middle of the data. The difference between the first quartile Q1 and the third quartile Q3 is called the interquartile range, and half of this interquartile range is called the quartile deviation. This quartile deviation is also referred to as a semi-interquartile range.

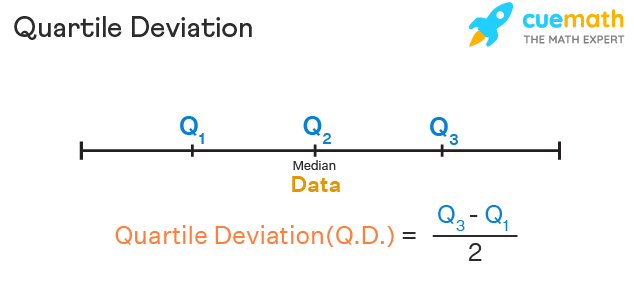
Quartile Deviation

Quartile Deviation = (Third Quartile – First Quartile) / 2

Quartile Deviation =(Q3 – Q1) / 2

Quartile deviation can be calculated for both the grouped data and the ungrouped data. Quartile deviation measures the absolute level of dispersion and is not affected by the extreme values. And the relative measure with reference to quartile deviation is known as the coefficient of quartile deviation.

Coefficient of Quartile Deviation = (Q3 – Q1) / (Q3 + Q1)

How to Find Quartile Deviation?

The quartile deviation can be calculated in two different methods, based on the type of given data. The quartile deviation is calculated differently for ungrouped data and for the grouped data. The quartile deviation is

  • Arrange the available data in ascending or both the grouped and ungrouped data.
  • Find the first quartile value using one of these formulas. For ungrouped data use the formula Q1 = (n + 1)/4, and for ungrouped data use the formula \(Q_1 =l_1 + \dfrac{(N/4) - c}{f}(l_2 - l_1)\). Here n is for the particular quartile, N is the total frequency, f is the frequency of the particular class, c is the cumulative frequency of the preceding class, and l1, l2 are the lower and upper boundaries of the class interval.

  • Find the third quartile the formula for ungrouped data is Q3= 3(n + 1)/4, and for ungrouped data the formula is \(Q_3 =l_1 + \dfrac{3(N/4) - c}{f}(l_2 - l_1)\).

  • Finally, calculate the quartile deviation using the formula Q.D = (Q3 - Q1)/2.

Related Topics

The following topics help in a better understanding of quartile deviation.

 

Examples on Quartile Deviation

  1. Example 1: Find the quartile deviation and the coefficient of quartile deviation for the following given data.

    23, 8, 5, 16, 33, 7, 24, 5, 30, 33, 37, 30, 9, 11, 26, 32

    Solution:

    The given data points are 23, 8, 5, 16, 33, 7, 24, 5, 30, 33, 37, 30, 9, 11, 26, 32

    Let us arrange this data in the following ascending order.

    5, 5, 7, 8, 9, 11, 16, 23, 24, 26, 30, 30, 32, 33, 33, 37

    From the above data we have Q1 = ( 8 + 9)/2 = 17/2 = 8.5, and Q3 = (30 + 32)/2 = 62/2 = 31

    Quartile Deviation = \(\dfrac{Q_3 - Q_1}{2} = \dfrac{31 - 8.5}{2} = \dfrac{22.5}{2} = 11.25\)

    Coefficient of Quartile Deviation = \(\dfrac{Q_3 - Q_1}{Q_3 + Q_1} = \dfrac{31 - 8.5}{31 + 8.5} = \dfrac{22.5}{39.5} =0.57 \)

    Therefore, the quartile deviation is 11.25, and the coefficient of quartile deviation is 0.57.

  2. Example 2: Find the quartile deviation of the marks scored by 50 students of a class.

    Class Interval of Marks 45 - 50 50-55 55 - 60 60-65 65-70 70-75
    Number of Students (Frequency) 7 5 12 11 9 6

    Solution:

    The given information is presented as follows.

    Class Interval Frequency Cumulative Frequency
    45 - 50 7 7
    50 - 55 5 7 + 5 = 12
    55 - 60 12 12 + 12 = 24
    60 - 65 11 24 + 11 = 35
    65 - 70 9 35 + 9 = 44
    70 - 75 6 44 + 6 = 50

    N = 50

    N/4 = 50/4 = 12.5

    3N/4 = 3(50/4) = 2(12.5) = 37.5

    Hence the class containing Q1 is 55 - 60, and the class containing Q3 is 65 - 70.

    The formula to find the quartile is \(Q_n =l_1 + \dfrac{n(N/4) - c}{f}(l_2 - l_1)\).

    Here we have the following notations.

    n = Quartile

    N = Total Frequency

    f = Frequency of the particular class

    c = Cumulative Frequency of the preceding class,

    l1 = Lower Boundary of the Class Interval l2 = Upper Boundary of the Class Interval.

    The calculation for the first quartile Q1 is as follows.

    n = 1, N = 50, f = 12, c = 12, l1 = 55, and l2 = 60

    \(Q_1 =l_1 + \dfrac{(N/4) - c}{f}(l_2 - l_1)\)

    \(Q_1 = 55 + \dfrac{(50/4) - 12}{12}(60 - 55)\)

    \(Q_1 =55 + \dfrac{(12.5 - 12}{12}(5)\)

    Q1 = 55 +(0.5)5/12 = 55 + 2.5/12 = 55 + 0.2083

    Q1 = 55.21

    And the calculation for the third quartile Q3 is as follows.

    n = 3, N = 50, f = 9, c = 35, l1 = 65, and l2 = 70

    \(Q_3 = l_1 + \dfrac{3(N/4) - c}{f}(l_2 - l_1)\)

    \(Q_3 = 65 + \dfrac{3(50/4) - 35}{9}(70 - 65)\)

    \(Q_3 = 65 + \dfrac{(37.5 - 35}{9}(5)\)

    Q1 = 65 +(2.5)5/9 = 55 + 7.5/9 = 55 + 0.83

    Q3 = 65.83

    Further, we need to find the quartile deviation of this data.

    Quartile Deviation = \(\dfrac{Q_3 - Q_1}{2} = \dfrac{65.83 - 55.21}{2} = \dfrac{10.62}{2} = 5.31\)

    Therefore, the quartile deviation of the marks of the students is 5.31.

View Answer >

go to slidego to slide

Great learning in high school using simple cues
Indulging in rote learning, you are likely to forget concepts. With Cuemath, you will learn visually and be surprised by the outcomes.

Book a Free Trial Class

FAQs on Quartile Deviation

What Is Quartile Deviation?

Quartile deviation is a dispersion in the middle of the data and is a statistic that defines the spread of the data. Quartile Deviation is half of the difference between the first quartile Q1 and the third quartile Q3 of the given data which has been arranged in ascending order. The quartile deviation is also referred to as a semi-interquartile range.

What Is the Formula For Quartile Deviation?

The formula for quartile deviation is Q.D = \(\dfrac{Q_3 - Q_1}{2}\), and Q3 is the third quartile or the median of the second half of the data, and Q1 is the first quartile or the median of the first half of the data. The values of Q1 and Q3 can be easily calculated using the for ungrouped data by arranging the data in ascending order. But for grouped data the formula to find the quartiles is \(Q_n =l_1 + \dfrac{n(N/4) - c}{f}(l_2 - l_1)\). Here n is for the particular quartile, N is the total frequency, f is the frequency of the particular class, c is the cumulative frequency of the preceding class, and l1, l2 are the lower and upper boundaries of the class interval.

How Do We Calculate Quartile Deviation?

To calculate quartile deviation we first need to find the first quartile Q1, and the third quartile Q3 of the given data. For ungrouped data, the data is arranged in ascending order and the median of the first half of the data is Q1, and the median for the second half of the data is Q3. And for grouped data the quartiles are calculated using the formula \(Q_n =l_1 + \dfrac{n(N/4) - c}{f}(l_2 - l_1)\). Finally the quartile deviation is calculated using the formula Q.D = \(\dfrac{Q_3 - Q_1}{2}\).

What Is Coefficient of Quartile Deviation?

The relative measure with reference to quartile deviation is known as the coefficient of quartile deviation. The coefficient of quartile deviation is the difference of the third quartile and the first quartile, divided by the sum of the third quartile and the first quartile. Coefficient of Quartile Deviation = (Q3 – Q1) / (Q3 + Q1).

How Do We Calculate Q1, Q3 for Quartile Deviation?

The Q1, and Q3 values for ungrouped data is calculated by arranging the data in ascending order and taking the median of the first half of the data as Q1, and the median of the second half of the data as Q3. And for grouped data the quartiles are calculated using the formula \(Q_n =l_1 + \dfrac{n(N/4) - c}{f}(l_2 - l_1)\). Here n is for the particular quartile, N is the total frequency, f is the frequency of the particular class, c is the cumulative frequency of the preceding class, and l1, l2 are the lower and upper boundaries of the class interval.

What Is the Difference Between Standard Deviation and Quartile Deviation?

The standard deviation is the square root of the average of the deviation of each of the data wth reference to the mean of the data. And the quartile deviation is the half of the interquartile range of the given data.

What Are The Uses of Quartile Deviation?

The quartile deviation helps to measure the spread of the data with reference to the central medin value. It helps to avoid the extreme value of the data or the outliers of the data, and it only measures the real range of the data from the first quartile to the third quartile. Unlike range, the quartile deviation includes only the real part of the data.

Math worksheets and
visual curriculum