Paired T-Test
In Statistics, a t-test can be represented as a statistical hypothesis test where the test statistic supports a student’s t distribution if the null hypothesis is established. In Paired T-Test, they compare the means of two groups of observations. The observations must be randomly assigned to each of the two groups so that the difference in response seen is due to the treatment and not because of any other factors. If two samples are given, then the observation of one sample can be paired with the observation of the other sample, by using the paired t-test.
Further, this test can be used in making observations on the same sample before and after an event. Now, let us discuss what is paired t-test, its formula, table, and the procedure to perform the paired t-test in detail.
Paired T-Test Definition
The paired t-test gives a hypothesis examination of the difference between population means for a set of random samples whose variations are almost normally distributed. Subjects are often tested in a before-after situation or with subjects as alike as possible. The paired t-test is a test that the differences between the two observations are zero.
Let us assume two paired sets, such as Xi and Yi for i = 1, 2, …, n such that their paired difference is independent which is identically and normally distributed. Then the paired t-test concludes whether they notably vary from each other.
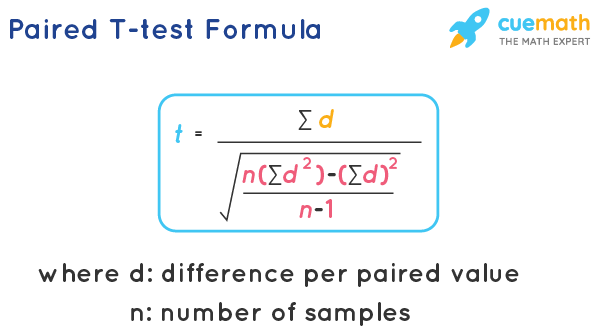
Paired T-Test Formula
Paired T-test is a test that is based on the differences between the values of a single pair, that is one deducted from the other. In the formula for a paired t-test, this difference is notated as d. The formula of the paired t-test is defined as the sum of the differences of each pair divided by the square root of n times the sum of the differences squared minus the sum of the squared differences, overall n-1.
The formula for the paired t-test is given by
\(t = \frac{\sum d}{\sqrt{\frac{n(\sum d^{2})-(\sum d)^{2}}{n-1}}}\)
Where, Σd is the sum of the differences.
Paired T-Test Table
Paired T-test table enables the t-value from a t-test to be converted to a statement about significance. The table is given below:
\(\begin{array}{l}
\text { Paired T Test Table }\\
\begin{array}{|c|c|c|c|c|c|c|}
\hline {\text { Two Tailed Significance }} \\
\hline \begin{array}{c}
\text { Degrees of } \\
\text { fredom }(n-1)
\end{array} & \alpha=0.20 & 0.10 & 0.05 & 0.02 & 0.01 & 0.002 \\
\hline & & & & & & \\
\hline 1 & 3.078 & 6.314 & 12.706 & 31.821 & 63.657 & 318.300 \\
\hline 2 & 1.886 & 2.920 & 4.303 & 6.965 & 9.925 & 22.327 \\
\hline 3 & 1.638 & 2.353 & 3.182 & 4.541 & 5.841 & 10.214 \\
\hline 4 & 1.533 & 2.132 & 2.776 & 3.747 & 4.604 & 7.173 \\
\hline 5 & 1.476 & 2.015 & 2.571 & 3.305 & 4.032 & 5.893 \\
\hline 6 & 1.440 & 1.943 & 2.447 & 3.143 & 3.707 & 5.208 \\
\hline 7 & 1.415 & 1.895 & 2.365 & 2.998 & 3.499 & 4.785 \\
\hline 8 & 1.397 & 1.860 & 2.306 & 2.896 & 3.355 & 4.501 \\
\hline 9 & 1.383 & 1.833 & 2.262 & 2.821 & 3.250 & 4.297 \\
\hline 10 & 1.372 & 1.812 & 2.228 & 2.764 & 3.169 & 4.144 \\
\hline 11 & 1.363 & 1.796 & 2.201 & 2.718 & 3.106 & 4.025 \\
\hline 12 & 1.356 & 1.782 & 2.179 & 2.681 & 3.055 & 3.930 \\
\hline 13 & 1.350 & 1.771 & 2.160 & 2.650 & 3.012 & 3.852 \\
\hline 14 & 1.345 & 1.761 & 2.145 & 2.624 & 2.977 & 3.787 \\
\hline 15 & 1.341 & 1.753 & 2.131 & 2.602 & 2.947 & 3.733 \\
\hline
\end{array}
\end{array}\)
Paired Vs Unpaired T-Test
The similarity between paired and unpaired t-test is that both assume data from the normal distribution.
Characteristics of Unpaired T-Test:
- The two groups taken should be independent.
- The sample size of the two groups need not be equal.
- It compares the mean of the data of the two groups.
- 95% confidence interval for the mean difference is calculated.
Characteristics of Paired T-Test:
- The data is taken from subjects who have been measured twice.
- 95% confidence interval is obtained from the difference between the two sets of joined observations.
How to Find the Paired T-Test?
Let us take two sets of data that are related to each other, say X and Y with xi ∈ X, yi ∈ Y. where i = 1, 2,……., n. Follow the steps given below to find the paired t-test.
- Assume the null hypothesis that the actual mean difference is zero.
- Determine the difference di = yi – xi between the set of observations.
- Compute the mean difference.
- Calculate the standard error of the mean difference, which is equal to Sd /√n, where n is the total number, and Sd is the standard deviation of the difference.
- Determine the t-statistic value.
- Refer to the T-distribution table and compare it with the tn-1 distribution. It gives the p-value.
Important Notes on Paired T-Test
Here are a few important notes on the Paired T-Test:
- The data is taken from subjects who have been measured twice.
- 95% confidence interval is obtained from the difference between the two sets of joined observations.
Related Topics
FAQs on Paired T-Test
How Do You Know if Paired or Unpaired T-Test?
A paired t-test is designed to compare the means of the same group or item under two separate scenarios. An unpaired t-test compares the means of two independent or unrelated groups. In an unpaired t-test, the variance between groups is assumed to be equal. In a paired t-test, the variance is not assumed to be equal.
Should I Use Paired or Unpaired T-Test?
If the data are paired or matched, then you should choose a paired t-test instead. If the pairing is effective in controlling for experimental variability, the paired t-test will be more powerful than the unpaired test.
What Is the Difference Between a Paired T-Test and a 2 Sample T-Test?
A two-sample t-test is used when the data of two samples are statistically independent, while the paired t-test is used when data is in the form of matched pairs. ... To use the two-sample t-test, we need to assume that the data from both samples are normally distributed and have the same variances.
Why Would You Use a Paired T-Test?
A paired t-test is used when we are interested in the difference between two variables for the same subject. Often the two variables are separated by time. ... Since we are ultimately concerned with the difference between two measures in one sample, the paired t-test reduces to the one-sample t-test.
How Do You Interpret a Paired T-Test?
Complete the following steps to interpret a paired t-test.
...
- Step 1: Determine a confidence interval for the population mean difference. First, consider the mean difference, and then examine the confidence interval. ...
- Step 2: Determine whether the difference is statistically significant. ...
- Step 3: Check your data for problems.
How Do You Know if Data Is Paired?
Two data sets are "paired" when the following one-to-one relationship exists between values in the two data sets.
- Each data set has the same number of data points.
- Each data point in one data set is related to one, and only one, data point in the other data set.