Confidence Interval Formula

The confidence interval formula is used in statistics for describing the amount of uncertainty associated with a sample estimate of a population parameter. It is used to describe the uncertainty associated with a sampling method. 

To recall, the confidence interval is a range within which most possible values would occur. To calculate the confidence interval, one needs to set the confidence level as 90%, 95%, or 99%, etc. A 90% confidence level means that we would expect 90% of the interval estimates to include the population parameter; 95% of the intervals would include the parameter and so on. 

Let us understand the confidence interval formula using solved examples.

What is Confidence Interval Formula?

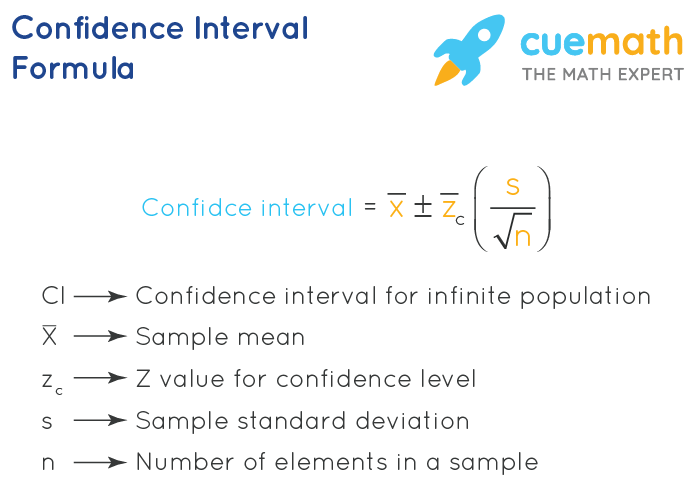
A confidence interval gives the probability within which the true value of the parameter will lie. The confidence level (in percentage) is selected by the investigator. The higher the confidence level is the wider is the confidence interval (less precise). Before learning the confidence interval, one must understand the basic statistics formulas and z-score formula. The formula for the confidence interval is given below:

Confidence Interval Formulas:

If n ≥ 30, Confidence Interval = x̄ ± z_c(σ/√n)

If n<30, Confidence Interval = x̄ ± t_c(S/√n)

Where,

• n = Number of terms
• x̄ = Sample Mean
• σ = Standard Deviation 
• z_c = Value corresponding to confidence interval in z table
• t_c = Value corresponding to confidence interval in t table

Examples Using Confidence Interval Formula

Example 1: A random sample of 30 apples was taken from a large population. On measuring their diameter the mean diameter of the sample was 91 millimeters with a standard deviation of 8 mm. Calculate the 85% confidence limits for the mean diameter of the whole population of apples. 

Solution:

For 85% confidence, Z = 1.440 We have: \(\bar{x}=91, s=8, Z=1.440\) and \(n=30\)
Substitute into confidence interval formula:

\(\bar{x} \pm Z \frac{s}{\sqrt{n}}\)
Therefore, the 85% confidence limits are:
\(=91 \pm 1.440 \times \frac{8}{\sqrt{30}}\)
\(=91 \pm 1.440 \times \frac{8}{5.477 \ldots}\)
\(=91 \pm 2.1\)
Answer: The 85% confidence limits are \(=91 \pm 2.1\)

Example 2: A random sample of ten scores obtained by the students in a Math test are as follows: 2, 16, 3, 10, 11, 4, 6, 7, 9, 12. What will be the 90% confidence limits for the mean of the whole sample?

Solution:

First, calculate the sample mean:
\(\bar{x}=\frac{2+3+4+6+7+9+10+11+12+16}{10}=\frac{80}{10}=8\)
Now the sample standard deviation:
Because this is a sample, we use the formula \(s=\sqrt{\frac{1}{n-1} \sum_{i=1}^{n}\left(x_{i}-\bar{x}\right)^{2}}\)
with \(\bar{x}=8\) and \(n-1=9\)
Therefore \(s=\)
\(\begin{array}{l}
\sqrt{\frac{1}{9}\left((2-8)^{2}+(3-8)^{2}+(4-8)^{2}+(6-8)^{2}+(7-8)^{2}+(9-8)^{2}+(10-8)^{2}+(11-8)^{2}+(12-8)^{2}+(16-8)^{2}\right)} \\
=\sqrt{\frac{1}{9}(36+25+16+4+1+1+4+9+16+64)} \\
=\sqrt{\frac{1}{9} \times 176}
\end{array}\)
\(=\sqrt{19.555 \ldots}\)
\(=4.4221 \ldots\)
For \(90 \%\) confidence, \(\mathrm{Z}=1.645\)

Therefore, the \(90 \%\) confidence limits are \(\bar{x} \pm Z \frac{s}{\sqrt{n}}\) \(=8 \pm 1.645 \times \frac{4.4221 \ldots}{\sqrt{10}}\)
\(=8 \pm 1.645 \times \frac{4.4221 \ldots}{3.1622 \ldots} =8 \pm 2.3\)

Answer: The 90% confidence limits are \(8 \pm 2.3\)

Example 3: Out of hundreds of people. You randomly chose 46 men with a mean of 86 inches (height) with a standard deviation of 6.2 inches. Determine that the selected men are tall enough.

Solution: 

Given: Mean, X̄ = 86

Standard deviation, σ = 6.2

Total observations, n = 46

Taking the confidence level as 95%, the value of z = 1.960

The formula to find the confidence interval is

X̄ ± Zα/2 × [ σ / √n ]

Substitute the given values in the formula, we get

86 ± 1.960 × [ 6.2 / √46 ]

86 ± 1.960 × [ 6.2 / 6.78]

86 ± 1.960 × 0.914

86 ± 1.79

So, the margin of error is 1.79

Answer: Therefore, all the hundreds of people are likely to be between in the range of 84. 21 and 87.79 inches.