Common Ratio Formula

Before learning the common ratio formula, let us recall what is the common ratio. The number multiplied (or divided) at each stage of a geometric sequence is called the "common ratio", because if you divide (that is, if you find the ratio of) successive terms, you'll always get this value. The common ratio formula helps in calculating the common ratio for a given geometric progression. 

What Is the Common Ratio Formula?

The general form of representing a geometric progression is a₁, (a₁r), (a₁r²), (a₁r³), (a₁r⁴) ,... where a₁ is the first term of GP, a₁r is the second term of GP, and r is the common ratio. Thus, the common ratio formula of a geometric progression is given as,

Common Ratio Formula:

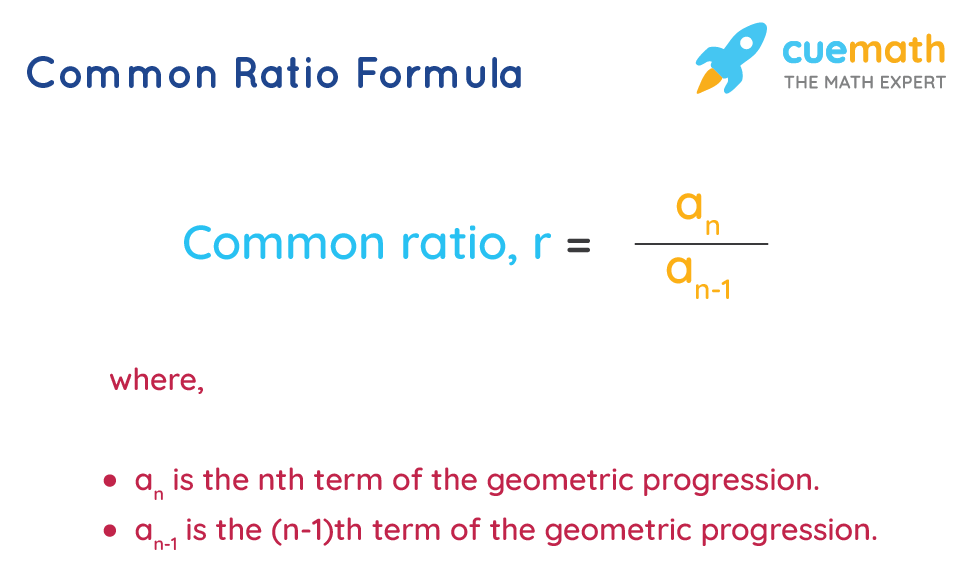
Common ratio, \(r = \frac{a_n}{a_{n-1}}\) 

where,

• a\(_n\) is the n^th term of the geometric progression.
• a\(_{n-1}\) is the (n - 1)^th term of the geometric progression.

Let us see the applications of the common ratio formula in the following section.

Examples Using Common Ratio Formula

Example 1: Find the common ratio for the geometric sequence 1, 2, 4, 8, 16,... using the common ratio formula.

Solution:     

To find: Common ratio

Divide each term by the previous term to determine whether a common ratio exists.

\(\frac{2}{1} = \frac{4}{2} = \frac{8}{4} = \frac{16}{8} = 2 \)

The sequence is geometric because there is a common multiple, 2, which is called the common ratio.

Answer:  Common ratio, r = 2

Example 2: What is the common ratio for a geometric sequence whose formula for the n^th term is given by: a\(_n\) = 4(3)^n-1?

Solution:       

To find: Common ratio

Given: Formula of geometric sequence = 4(3)^n-1

A listing of the terms will show what is happening in the sequence (start with n = 1). 

4, 12, 36,108,...

Using the common ratio formula,

r = 12/4 = 3

Answer:  Common ratio, r = 3