Quotient Rule
Quotient rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two differentiable functions. That means, we can apply the quotient rule when we have to find the derivative of a function of the form: f(x)/g(x), such that both f(x) and g(x) are differentiable, and g(x) ≠ 0. The quotient rule follows the product rule and the concept of limits of derivation in differentiation directly. Let us understand the formula for quotient rule, its proof using solved examples in detail in the following sections.
| 1. | What is Quotient Rule? |
| 2. | Quotient Rule Formula |
| 3. | Derivation of Quotient Rule Formula |
| 4. | How to Apply the Quotient Rule in Differentiation? |
| 5. | FAQs on Quotient Rule |
What is the Quotient Rule?
Quotient rule in calculus is a method used to find the derivative of any function given in the form of a quotient obtained from the result of the division of two differentiable functions. The quotient rule in words states that the derivative of a quotient is equal to the ratio of the result obtained on the subtraction of the numerator times the derivative of the denominator from the denominator times the derivative of the numerator to the square of the denominator. That means if we are given a function of the form: f(x) = u(x)/v(x), we can find the derivative of this function using the quotient rule derivative as,
f'(x) = [u(x)/v(x)]' = [v(x) × u'(x) - u(x) × v'(x)]/[v(x)]2
Quotient Rule Formula
We can calculate the derivative or evaluate the differentiation of the quotient of two functions using the quotient rule derivative formula. The quotient rule derivative formula is given as,
f'(x) = [u(x)/v(x)]' = [v(x) × u'(x) - u(x) × v'(x)]/[v(x)]2
where,
- f(x) = The function of the form u(x)/v(x) for which the derivative is to be calculated.
- u(x) = A differentiable function that makes numerator of the function f(x).
- u'(x) = Derivative of function u(x).
- v(x) = A differentiable function that makes denominator of the given function f(x).
- v'(x) = Derivative of the function v(x).
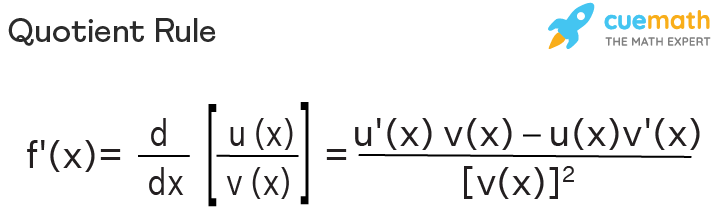
Derivation of Quotient Rule Formula
In the previous section, we learned about the quotient formula to find derivatives of the quotient of two differentiable functions. Let us see the proof of the quotient rule formula here. There are different methods to prove the quotient rule formula, given as,
- Using derivative and limit properties
- Using implicit differentiation
- Using chain rule
Quotient Rule Formula Proof Using Derivative and Limit Properties
To prove quotient rule formula using the definition of derivative or limits, let the function f(x) = u(x)/v(x).
⇒ f'(x) = \(\mathop {\lim }\limits_{h \to 0}\) [f(x + h) - f(x)]/h
= \(\mathop {\lim }\limits_{h \to 0}\) \(\frac{\frac{u(x+h)}{v(x+h)} - \frac{u(x)}{v(x)}}{h}\)
= \(\mathop {\lim }\limits_{h \to 0}\) \(\frac{u(x+h)v(x) - u(x)v(x+h)}{h \cdot v(x) \cdot v(x+h)}\)
= \( \left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x) - u(x)v(x+h)}{h}\right) \left(\mathop {\lim }\limits_{h \to 0} \frac{1}{v(x) \cdot v(x+h)}\right)\)
= \(\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x) - u(x)v(x) + u(x)v(x) - u(x)v(x + h)}{h}\right) \) [1/v(x)2]
= \(\left[\!\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x) - u(x)v(x)}{h}\right)\!\!\! -\!\! \left(\mathop {\lim }\limits_{h \to 0} \frac{u(x)v(x + h) - u(x)v(x)}{h}\right)\!\right]\) [1/v(x)2]
= \(\left[\!v(x)\!\left(\!\mathop {\lim }\limits_{h \to 0} \frac{u(x+h) - u(x)}{h}\right)\!\!\!-\!\!u(x)\!\!\left( \mathop {\lim }\limits_{h \to 0} \!\!\frac{v(x + h) - v(x)}{h} \right)\!\!\right]\) [1/v(x)2]
= \(\frac{v(x)u'(x) - u(x)v'(x)}{[v(x)]^2}\)
Quotient Rule Formula Proof Using Implicit Differentiation
To prove the quotient rule formula using implicit differentiation formula, let us take a differentiable function f(x) = u(x)/v(x), so u(x) = f(x)⋅v(x). Using the product rule, we have, u'(x) = f'(x)⋅v(x) + f(x)v'(x). Solving for f'(x), we get,
f'(x) = \(\frac{u'(x) - f(x)v'(x)}{v(x)}\)
Substitute f(x),
⇒ f'(x) = \(\frac{u'(x) - \frac{u(x)}{v(x)}v'(x)}{v(x)}\)
= \(\frac{u'(x)v(x) - u(x)v'(x)}{[v(x)]^2}\)
Quotient Rule Formula Proof Using Chain Rule
We can derive the quotient rule formula in calculus using the chain rule formula. Let f(x) be a differentiable function such that f(x) = u(x)/v(x).
⇒ f(x) = u(x)v-1(x)
Using the product rule, f'(x) = u'(x)v-1(x) + u(x)⋅\(\left(\frac{d}{dx}(v^{-1}(x)\right)\)
Applying the power rule to solve the derivative in the second term, we have,
f'(x) = u'(x)v-1(x) + u(x)⋅(-1)(v(x)-2)v'(x)
f'(x) = \(\frac{u'(x)}{v(x)} - \frac{u(x)v'(x)}{v(x)^2}\)
f'(x) = \(\frac{d}{dx} \left[ \frac{u(x)}{v(x)} \right] \) = \(\frac{u'(x)v(x) - u(x)v'(x)}{v(x)^2}\)
How to Apply Quotient Rule in Differentiation?
In order to find the derivative of the function of the form f(x) = u(x)/v(x), both u(x) and v(x) should be differentiable functions. We can apply the following given steps to find the derivation of a differentiable function f(x) = u(x)/v(x) using the quotient rule.
- Step 1: Note down the values of u(x) and v(x).
- Step 2: Find the values of u'(x) and v'(x) and apply the quotient rule formula, given as: f'(x) = [u(x)/v(x)]' = [u'(x) × v(x) - u(x) × v'(x)]/[v(x)]2
Let us have a look at the following example given below to understand the quotient rule better.
Example: Find f'(x) for the following function f(x) using the quotient rule: f(x) = x2/(x+1).
Solution:
Here, f(x) = x2/(x + 1)
u(x) = x2
v(x) = (x + 1)
⇒u'(x) = 2x
⇒v'(x) = 1
⇒f'(x) = [v(x)u'(x) - u(x)v'(x)]/[v(x)]2
⇒f'(x) = [(x+1)•2x - x2•1]/(x + 1)2
⇒f'(x) = (2x2 + 2x - x2)/(x + 1)2
⇒f'(x) = (x2 + 2x)/(x + 1)2
Answer: The derivative of x2/(x + 1) is (x2 + 2x)/(x + 1)2.
☛ Topics Related to Quotient Rule:
Examples on Quotient Rule
-
Example 1: Find the derivative of \(\frac{\cos{x}}{x}\) using the quotient rule formula.
Solution:
Let f(x) = cos x and g(x) = x.
\(\frac{d}{{dx}}\left\{ {\frac{{f\left( x \right)}}{{g\left( x \right)}}} \right\} = \frac{{g\left( x \right)f'\left( x \right) - f\left( x \right)g'\left( x \right)}}{{{{\left( {g\left( x \right)} \right)}^2}}}\\=\frac{x(-\sin{x})-\cos{x}(1)}{x^2}\\=-\frac{x\sin{x}+\cos{x}}{x^2}\)
Answer: The derivative of \(\frac{\cos{x}}{x}\) is \(-\frac{x\sin{x}+\cos{x}}{x^2}\). -
Example 2: Differentiate \(\frac{\log{x}}{x}\) using the quotient rule formula.
Solution:
Let f(x) = log x and g(x) = x.
\(\frac{d}{{dx}}\left\{ {\frac{{f\left( x \right)}}{{g\left( x \right)}}} \right\}= \frac{{g\left( x \right)f'\left( x \right) - f\left( x \right)g'\left( x \right)}}{{{{\left( {g\left( x \right)} \right)}^2}}}\\=\frac{x(1/x)-\log{x}}{x^2}\\=\frac{1-\log{x}}{x^2}\)
Answer: The derivative of \(\frac{\log{x}}{x}\) is \(\frac{1-\log{x}}{x^2}\). -
Example 3: Apply the quotient rule to differentiate \(\frac{1-2x}{x}\).
Solution:
Let f(x) = (1-2x)/x
f'(x) = \(\frac{d}{dx}\) \(\frac{(1-2x)}{x}\) = [x \(\frac{d}{dx}\) (1-2x) - (1-2x) \(\frac{d}{dx}\) x]/x2f'(x) = [x(-2) - (1-2x) (x)]/x2 = (-2x - 1 + 2x)/x2 = -1/x2
Answer: The derivative of \(\frac{(1-2x)}{x}\) is -1/x2.

FAQs on Quotient Rule
What is Quotient Rule of Differentiation in Calculus?
The quotient rule is one of the derivative rules that we use to find the derivative of functions of the form P(x) = f(x)/g(x). The derivative of a function P(x) is denoted by P'(x). If the derivative of the function P(x) exists, we say P(x) is differentiable. So, differentiable functions are those functions whose derivatives exist. A function P(x) is differentiable at a point x = a if the following limit exists.
\(P'(x) = \mathop {\lim }\limits_{h \to 0} \frac{P(a+h)-P(a)}{h}\)
How to Find Derivative Using Quotient Rule?
The derivatives of the quotient for the ratio of two differentiable functions can be calculated in calculus using the quotient rule. We need to apply the quotient rule formula for differentiation of function f(x) = u(x)/v(x). The quotient rule formula is given as,
f'(x) = [u(x)/v(x)]' = [u'(x) × v(x) - u(x) × v'(x)]/[v(x)]2
where, f'(x), u'(x) and v'(x) are derivatives of functions f(x), v(x) and u(x).
What is Quotient Rule Formula?
Quotient rule derivative formula is a rule in differential calculus that we use to find the derivative of rational functions. Suppose two functions, u(x) and v(x) are differentiable, then the quotient rule can be applied to find (d/dx)[u(x)/v(x)] as,
f'(x) = [u(x)/v(x)]' = [u'(x) × v(x) - u(x) × v'(x)]/[v(x)]2
How to Derive the Formula for Quotient Rule?
Quotient rule formula can be derived using different methods. They are given as,
- Using derivative and limit properties
- Using implicit differentiation
- Using chain rule
Using chain rule, we can derive the quotient rule formula by taking f(x) as a differentiable function such that f(x) = u(x)/v(x). ⇒ f(x) = u(x)v-1(x)
Using the product rule, f'(x) = u'(x)v-1(x) + u(x)⋅\(\left(\frac{d}{dx}(v^{-1}(x)\right)\)
⇒f'(x) = u'(x)v-1(x) + u(x)⋅(-1)(v(x)-2)v'(x)
⇒f'(x) = \(\frac{d}{dx} \left[ \frac{u(x)}{v(x)} \right] \) = \(\frac{u'(x)v(x) - u(x)v'(x)}{[v(x)]^2}\)
How to Use Quotient Rule in Differentiation?
Quotient rule can be used in finding the differentiation of a function f(x) of the form u(x)/v(x). Derivative of this function using quotient rule can be given as, f'(x) = \(\frac{d}{dx} \left[ \frac{u(x)}{v(x)} \right] \) = \(\frac{u'(x)v(x) - u(x)v'(x)}{v(x)^2}\)
How to Derive Quotient Rule Using Definition of Limits and Derivatives?
The proof of the quotient rule can be given using the definition and properties of limits and derivatives. For a function f(x) = u(x)/v(x), the derivative f'(x) can be given as,
⇒ f'(x) = \(\mathop {\lim }\limits_{h \to 0}\) [f(x + h) - f(x)]/h
= \(\mathop {\lim }\limits_{h \to 0}\) \(\frac{\frac{u(x+h)}{v(x+h)} - \frac{u(x)}{v(x)}}{h}\)
= \(\mathop {\lim }\limits_{h \to 0}\) \(\frac{u(x+h)v(x)\) - \(u(x)v(x+h)}{h \cdot v(x) \cdot v(x+h)}\)
= \( \left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x)\) - \(u(x)v(x+h)}{h}\right) \left(\mathop {\lim }\limits_{h \to 0} \frac{1}{v(x) \cdot v(x+h)}\right)\)
= \(\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x) - u(x)v(x) + u(x)v(x) - u(x)v(x + h)}{h}\right) \) [1/v(x)2]
= \(\left[\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h)v(x) - u(x)v(x)}{h}\right)\) - \(\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x)v(x + h) - u(x)v(x)}{h}\right)\right]\) [1/v(x)2]
= \(\left[v(x)\left(\mathop {\lim }\limits_{h \to 0} \frac{u(x+h) - u(x)}{h}\right)\)-\(u(x)\left( \mathop {\lim }\limits_{h \to 0} \frac{v(x + h) - v(x)}{h} \right)\right]\) [1/v(x)2]
What are Applications of Quotient Rule Derivative Formula? Give Examples.
We can apply the quotient rule to find the differentiation of the function of the form u(x)/v(x). For example, for a function f(x) = sin x/x, we can find the derivative as, f'(x) = [x \(\frac{d}{dx}\) sin x - sin x \(\frac{d}{dx}\) x]/x2, f'(x) = (x•cos x - sin x)/x2.
How do we Prove Quotient Rule Using Implicit Differentiation?
We can use the implicit differentiation method to derive the quotient rule, for a differentiable function f(x) = u(x)/v(x), so u(x) = f(x)⋅v(x). Using the product rule, we have, u'(x) = f'(x)⋅v(x) + f(x)v'(x). Solving for f'(x), we get,
f'(x) = \(\frac{u'(x) - f(x)v'(x)}{v(x)}\)
Substitute f(x),
⇒ f'(x) = \(\frac{u'(x) - \frac{u(x)}{v(x)}v'(x)}{v(x)}\)
= \(\frac{u'(x)v(x) - u(x)v'(x)}{[v(x)]^2}\)
How Can we Prove Quotient Rule Using the Product Rule in Calculus?
To prove the quotient rule using the product rule and chain rule, we can express the function f(x) = u(x)/v(x) as f(x) = u(x)•1/v(x) and further apply product rule formula to find f'(x) = (1/v(x))u'(x) - u(x)•(1/v(x))2•v'(x) = \(\frac{u'(x)v(x) - u(x)v'(x)}{[v(x)]^2}\).
visual curriculum