Inverse Trig Integrals
The inverse trig integrals are the integrals (or antiderivatives) of the inverse trigonometric functions. There are 6 inverse trig functions and they can be integrated using the method of integration by parts.
Let us learn more about the inverse trig integrals along with their proofs.
| 1. | What are Inverse Trig Integrals? |
| 2. | How to Find Integrals of Inverse Trig Functions? |
| 3. | Integrals that Produce in Inverse Trig Functions |
| 4. | FAQs on Inverse Trig Integrals |
What are Inverse Trig Integrals?
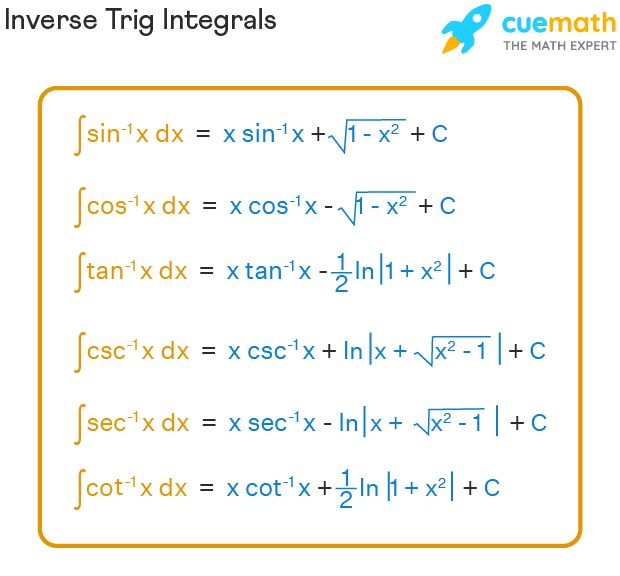
The inverse trig integrals are the integrals of the 6 inverse trig functions sin-1x (arcsin), cos-1x (arccos), tan-1x (arctan), csc-1x (arccsc), sec-1x (arcsec), and cot-1x (arccot). The integration by parts technique (and the substitution method along the way) is used for the integration of inverse trigonometric functions. The integrals of inverse trig functions are tabulated below:
| Inverse Trig Function | Integral |
|---|---|
| ∫ sin-1x dx | x sin-1x + √(1 - x²) + C |
| ∫ cos-1x dx | x cos-1x - √(1 - x²) + C |
| ∫ tan-1x dx | x tan-1x - (1/2) ln |1 + x²| + C |
| ∫ csc-1x dx | x csc-1x + ln |x + √(x² - 1)| + C |
| ∫ sec-1x dx | x sec-1x - ln |x + √(x² - 1)| + C |
| ∫ cot-1x dx | x cot-1x + (1/2) ln |1 + x²| + C |
How to Find Integrals of Inverse Trig Functions?
As mentioned earlier, we use the integration by parts to evaluate the integrals of inverse trig functions. To evaluate each of the above integrals, we assume the first function as the respective inverse trig function and the second function as 1. Here are the proofs of inverse trig integrals.
Proof of Integral of Inverse Sine
Let us prove that ∫ sin⁻¹x dx = x sin-1x + √(1 - x²) + C. For this, we write ∫ sin-1x dx as ∫ sin-1x · 1 dx. By ILATE rule, the first function = sin-1x and the second function = 1. Then by using the formula of integration by parts:
∫ sin-1x · 1 dx = sin-1x ∫ dx - ∫ [d/dx (sin-1x) ∫ 1 dx] dx
= sin-1x (x) - ∫ (1 / √(1 - x²)) x dx (Using the formula of derivative of sin inverse x)
= x sin-1x - ∫ (x / √(1 - x²)) dx
We solve this integral using the substitution method. By substituting 1 - x2 = u, we get -2x dx = du (or) x dx = -(1/2) du. Using this,
= x sin-1x - (-1/2) ∫ 1 / √u du
= x sin-1x + (1/2) ∫ u-1/2 du
= x sin-1x + (1/2) [(u1/2) / (1/2)] + C (by power rule of integration)
= x sin-1x + √u + C
= x sin-1x + √(1 - x²) + C
Therefore, ∫ sin-1x dx = x sin-1x + √(1 - x²) + C. For more detailed proof, click here.
Proof of Integral of Inverse Cosine
Let us prove that ∫ cos⁻¹x dx = x cos-1x - √(1 - x²) + C. We integrate this also just like earlier. Then by using integration by parts:
∫ cos-1x · 1 dx = cos-1x ∫ dx - ∫ [d/dx (cos-1x) ∫ 1 dx] dx
= cos-1x (x) - ∫ (-1 / √(1 - x²)) x dx (Using the formula of derivative of cos inverse x)
= x cos-1x + ∫ (x / √(1 - x²)) dx
Substituting 1 - x2 = u, we get -2x dx = du (or) x dx = -(1/2) du. Using this,
= x cos-1x + (-1/2) ∫ 1 / √u du
= x cos-1x - (1/2) ∫ u-1/2 du
= x cos-1x - (1/2) [(u1/2) / (1/2)] + C
= x cos-1x - √u + C
= x cos-1x - √(1 - x²) + C
Therefore, ∫ cos-1x dx = x cos-1x - √(1 - x²) + C.
Proof of Integral of Inverse Tan
Let us prove that ∫ tan⁻¹x dx = x tan-1x - (1/2) ln |1 + x²| + C. By integration by parts formula:
∫ tan-1x · 1 dx = tan-1x ∫ dx - ∫ [d/dx (tan-1x) ∫ 1 dx] dx
= tan-1x (x) - ∫ (1 / (1 + x²)) x dx (Using the formula of derivative of tan inverse x)
= x tan-1x - ∫ (x / (1 + x²)) dx
Substituting 1 + x2 = u, we get 2x dx = du (or) x dx = (1/2) du. Using this,
= x tan-1x - (1/2) ∫ 1 / u du
= x tan-1x - (1/2) ln |u| + C
= x tan-1x - (1/2) ln |1 + x²| + C
Therefore, ∫ tan-1x dx = x tan-1x - (1/2) ln |1 + x²| + C. For more detailed proof, click here.
We have so far found the inverse trig integrals of 3 inverse trig functions. We will see the proofs of the rest of the 3 functions in the "Examples" section below.
Integrals that Produce in Inverse Trig Functions
We have already seen how to find the integrals of inverse trig functions. On the contrary, the result of some functions would be inverse trig functions. Such integrals occur very frequently when we are solving the problems of integrals. All such integrals are tabulated below.
| Integral that Results in Inverse Trig Function | Result |
|---|---|
| ∫1/√(1 - x2).dx | sin-1x + C |
| ∫-1/√(1 - x2).dx | cos-1x + C |
| ∫1/(1 + x2).dx | tan-1x + C |
| ∫-1/(1 + x2).dx | cot-1x + C |
| ∫ 1/x√(x2 - 1).dx | sec-1x + C |
| ∫ -1/x√(x2 - 1).dx | csc-1x + C |
These formulas directly follow from derivatives of inverse trig functions. For example, the derivative of inverse sine is, d/dx (sin-1x) = 1/√(1 - x2).dx; integration is the reverse process of differentiation and so ∫1/√(1 - x2).dx = sin-1x + C. Similarly, one can verify all the other formulas.
Important Notes on Inverse Trig Integrals:
- The integral of an inverse trig function is very different from the integral that results in an inverse trig function.
- For example:
∫ sin⁻¹x dx = x sin-1x + √(1 - x²) + C whereas
∫1/√(1 - x2).dx = sin-1x + C
☛ Related Topics:
Inverse Trig Integrals Examples
-
Example 1: Derive the inverse trig formula of csc-1x.
Solution:
By using integration by parts:
∫ csc-1x · 1 dx = csc-1x ∫ dx - ∫ [d/dx (csc-1x) ∫ 1 dx] dx
= csc-1x (x) - ∫ ( -1/(x√x²-1)) x dx
= x csc-1x + ∫ (1 / √√(x²-1)) dx
Substituting x = sec u. Then dx = sec u tan u du. Also, √(x2 - 1) = √(sec2u - 1) = √(tan2u) = tan u.
= x csc-1x + ∫ (1 / tan u) (sec u tan u) du
= x csc-1x + ∫ sec u du
= x csc-1x + ln |sec u + tan u| + C (from the integral of sec u formula)
= x csc-1x + ln |sec u + √(sec2u - 1)| + C (by trig identities)
= x csc-1x + ln |x + √(x2 - 1)| + C
Therefore, ∫ csc⁻¹x dx = x csc-1x + ln |x + √(x2 - 1)| + C
Answer: x csc-1x + ln |x + √(x2 - 1)| + C.
-
Example 2: Evaluate the inverse trig integral ∫ sec-1x dx.
Solution:
By using integration by parts:
∫ sec-1x · 1 dx = sec-1x ∫ dx - ∫ [d/dx (sec-1x) ∫ 1 dx] dx
= sec-1x (x) - ∫ ( 1/(x√x²-1)) x dx
= x sec-1x - ∫ (1 / √√(x²-1)) dx
Substituting x = sec u. Then dx = sec u tan u du. Also, √(x2 - 1) = √(sec2u - 1) = √(tan2u) = tan u.
= x sec-1x - ∫ (1 / tan u) (sec u tan u) du
= x sec-1x - ∫ sec u du
= x sec-1x - ln |sec u + tan u| + C
= x sec-1x - ln |sec u + √(sec2u - 1)| + C (by trig formulas)
= x sec-1x - ln |x + √(x2 - 1)| + C
Therefore, ∫ sec⁻¹x dx = x sec-1x - ln |x + √(x2 - 1)| + C
Answer: x sec-1x - ln |x + √(x2 - 1)| + C.
-
Example 3: What is the inverse trig integral of cot-1x?
Solution:
By integration by parts formula:
∫ cot-1x · 1 dx = cot-1x ∫ dx - ∫ [d/dx (cot-1x) ∫ 1 dx] dx
= cot-1x (x) - ∫ (-1 / (1 + x²)) x dx (Using the formula of derivative of tan inverse x)
= x cot-1x + ∫ (x / (1 + x²)) dx
Substituting 1 + x2 = u, we get 2x dx = du (or) x dx = (1/2) du. Using this,
= x cot-1x + (1/2) ∫ 1 / u du
= x cot-1x + (1/2) ln |u| + C
= x cot-1x + (1/2) ln |1 + x²| + C.
Answer: x cot-1x + (1/2) ln |1 + x²| + C.

FAQs on Inverse Trig Integrals
What are the Formulas of Inverse Trig Integrals?
The formulas of inverse trig integrals are as follows:
- ∫ sin-1x dx = x sin-1x + √(1 - x2) + C
- ∫ cos-1x dx = x cos-1x - √(1 - x²) + C
- ∫ tan-1x dx = x tan-1x - (1/2) ln |1 + x2| + C
- ∫ csc-1x dx = x csc-1x + ln |x + √(x2 - 1)| + C
- ∫ sec-1x dx = x sec-1x - ln |x + √(x2 - 1)| + C
- ∫ cot-1x dx = x cot-1x + (1/2) ln |1+x2| + C
How to do the Integration of Inverse Trigonometric Functions?
To integrate the inverse trigonometric functions, say to integrate sin-1x, write "times 1" after it. i.e., sin-1x × 1. Then assume sin-1x as the first function and 1 as the second function and finally apply the UV formula. We need to use the substitution method of integration also in this process.
Can you Integrate Inverse Trig?
Yes, we can integrate the inverse trig functions by using the process of integration by parts. But since we have only one function in the integrand, we assume the second function to be 1.
What are the Integrals that Result in Inverse Trig?
There are some integrals that frequently occur when we solve the problems of integration and that result in inverse trig functions. They are as follows:
- ∫1/√(1 - x2).dx = sin-1x + C
- ∫ -1/(1 - x2).dx = cos-1x + C
- ∫1/(1 + x2).dx = tan-1x + C
- ∫ -1/x√(x2 - 1).dx = cosec-1 x + C
- ∫ 1/x√(x2 - 1).dx = sec-1x + C
- ∫ -1/(1 +x2 ).dx = cot-1x + C
How to Remember Integrals of Inverse Trig Functions?
Here are some tricks to remember the inverse trig integrals.
- All integrals of inverse trig functions have the first term to be " x times the given function". For example, ∫ sin-1x dx = x sin-1x + ...
- The integrals of tan-1x, cot-1x, csc-1x and sec-1x all have "ln" in it.
- The integrals of sin-1x and cos-1x differ only in sign of the √(1 - x2). i.e.,
∫ sin-1x dx = x sin-1x + √(1 - x2) + C
∫ cos-1x dx = x cos-1x - √(1 - x²) + C - The integrals of tan-1x and cot-1x differ only in sign of the (1/2) ln |1 + x2|. i.e.,
∫ tan-1x dx = x tan-1x - (1/2) ln |1 + x2| + C
∫ cot-1x dx = x cot-1x + (1/2) ln |1+x2| + C - The integrals of csc-1x and sec-1x differ only in sign of the ln |x + √(x2 - 1)|. i.e.,
∫ csc-1x dx = x csc-1x + ln |x + √(x2 - 1)| + C
∫ sec-1x dx = x sec-1x - ln |x + √(x2 - 1)| + C
The best idea is NOT to memorize these formulas, but to learn the method of deriving them.
visual curriculum