Inverse Trig Derivatives
Inverse trig derivatives are the derivatives of inverse trigonometric functions. We have 6 inverse trigonometric functions that are the inverses of the 6 basic trigonometric functions. The inverse trig derivatives are defined only in the domain of the inverse trigonometric functions which are stated as follows:
| Inverse Trigonometric Function | Domain |
|---|---|
| arcsin x | [-1, 1] |
| arccos x | [-1, 1] |
| arctan x | R |
| arccsc x | (-∞, -1] U [1, ∞) |
| arcsec x | (-∞, -1] U [1, ∞) |
| arccot x | R |
Let us learn the derivatives of inverse trigonometric functions with detailed proof. Also, let us solve a few problems related to the inverse trig derivatives.
| 1. | What are Inverse Trig Derivatives? |
| 2. | Derivatives of Inverse Trig Functions |
| 3. | Inverse Trig Derivatives Proofs |
| 4. | Inverse Trig Derivatives and Integrals |
| 5. | FAQs on Inverse Trig Derivatives |
What are Inverse Trig Derivatives?
The inverse trig derivatives are the derivatives of the inverse trigonometric functions arcsin (or sin-1), arccos (or cos-1), arctan (or tan-1), etc. We use implicit differentiation to find the derivatives of the inverse trig function which we we explore in detail in the upcoming section. Here are the inverse trig derivatives:
- The derivative of arcsin x is d/dx(arcsin x) = 1/√1-x², when -1 < x < 1
- The derivative of arccos x is d/dx(arccos x) = -1/√1-x², when -1 < x < 1
- The derivative of arctan x is d/dx(arctan x) = 1/(1+x²), for all x in R
- The derivative of arccsc x is d/dx(arccsc x) = -1/(|x|√x²-1), when x < -1 or x > 1
- The derivative of arcsec x is d/dx(arcsec x) = 1/(|x|√x²-1), when x < -1 or x > 1
- The derivative of arccot x is d/dx(arccot x) = -1/(1+x²), for all x in R
Derivatives of Inverse Trig Functions
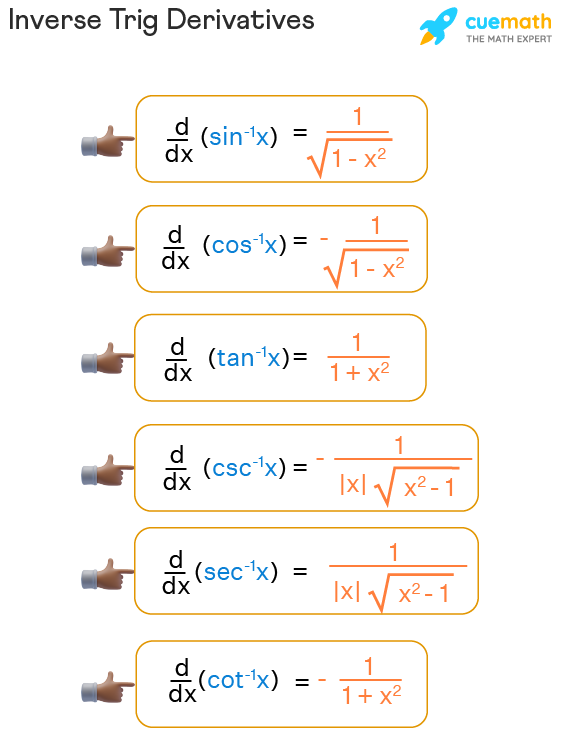
We know that another form of writing an inverse trig function, say arcsin x, is sin-1x. The derivatives of inverse trig functions can be written in alternative notation as follows:
- d/dx (sin-1x) = 1/√1-x²
- d/dx(cos-1x) = -1/√1-x²
- d/dx(tan-1x) = 1/(1+x²)
- d/dx(csc-1x) = -1/(|x|√x²-1)
- d/dx(sec-1x) = 1/(|x|√x²-1)
- d/dx(cot-1x) = -1/(1+x²)
Let us derive each of these derivative formulas.
Inverse Trig Derivatives Proofs
In the previous section, we have already seen the formulas of derivatives of inverse trigonometric functions. If we observe them carefully, the derivatives neither include trigonometric functions nor include inverse trigonometric functions. Rather they include squares and square roots. Thus, it is difficult to memorize them unless we know how these formulas are derived. We use the process of implicit differentiation (which is the process of using the chain rule when the functions are implicitly defined) to derive the inverse trig derivatives.
Derivative of Arcsin
To find the derivative of arcsin x, let us assume that y = arcsin x. Then by the definition of inverse sine, sin y = x. Differentiating both sides with respect to x,
cos y (dy/dx) = 1
dy/dx = 1/cos y ... (1)
By one of the trigonometric identities, sin2y + cos2y = 1. From this, cos y = √1-sin²y = √1-x².
Substituting this in (1),
dy/dx = 1/√1-x² (or)
d (arcsin x) / dx = 1/√1-x²
Thus, the derivative of arcsin x (or) sin-1x (or) inverse sin x is 1/√1-x².
Derivative of Arccos
To find the derivative of arccos x, let us assume that y = arccos x. Then by the definition of inverse cos, cos y = x. Differentiating both sides with respect to x,
-sin y (dy/dx) = 1
dy/dx = 1/(-sin y) = -1/sin y ... (1)
By one of the trigonometric identities, sin2y + cos2y = 1. From this, sin y = √1-cos²y = √1-x².
Substituting this in (1),
dy/dx = -1/√1-x² (or)
d (arccos x) / dx = 1/√1-x²
Thus, the derivative of arccos x (or) cos-1x (or) inverse cos x is 1/√1-x².
Derivative of Arctan
To find the derivative of arctan x, let us assume that y = arctan x. Then by the definition of inverse tan, tan y = x. Differentiating both sides with respect to x,
sec2y (dy/dx) = 1
dy/dx = 1/(sec2y) ... (1)
By one of the trigonometric identities, sec2y - tan2y = 1. From this, sec2y = 1 + tan2y = 1 + x2.
Substituting this in (1),
dy/dx = 1 / (1 + x2) (or)
d (arctan x) / dx = 1 / (1 + x2)
Thus, the derivative of arctan x (or) tan-1x (or) inverse tan x is 1 / (1 + x2).
Derivative of Arccsc
To find the derivative of arccsc x, let us assume that y = arccsc x. Then by the definition of inverse cosecant, csc y = x. Differentiating both sides with respect to x,
-csc y cot y (dy/dx) = 1
dy/dx = -1/(csc y cot y) ... (1)
By one of the trigonometric identities, csc2y - cot2y = 1. From this, cot2y = csc2y - 1 = x2 - 1. Then cot y = √x²-1.
Also, we have csc y = x.
Substituting this in (1),
dy/dx = -1/(|x|√x²-1)(or)
d (arccsc x) / dx = -1/(|x|√x²-1).
Here, we have written the absolute value sign around x instead of just writing x because if we observe the graph of csc-1x, the slope of the tangent of this curve is always negative. So the derivative of csc-1x must be always negative irrespective of the sign of x. That is why we always write the absolute value sign around x here.
Thus, the derivative of arccsc x (or) csc-1x (or) inverse csc x is -1/(|x|√x²-1).
Derivative of Arcsec
To find the derivative of arcsec x, let us assume that y = arcsec x. Then by the definition of inverse cosecant, sec y = x. Differentiating both sides with respect to x,
sec y tan y (dy/dx) = 1
dy/dx = 1/(sec y tan y) ... (1)
By one of the trigonometric identities, sec2y - tan2y = 1. From this, tan2y = sec2y - 1 = x2 - 1. Then tan y = √x²-1.
Also, we have sec y = x.
Substituting this in (1),
dy/dx = 1/(|x|√x²-1) (or)
d (arcsec x) / dx = 1/(|x|√x²-1).
Here also we have used the absolute value sign as the graph of sec-1x always has tangents with positive slopes and hence the derivative shouldn't be affected by the sign of x.
Thus, the derivative of arcsec x (or) sec-1x (or) inverse sec x is 1/(|x|√x²-1).
Derivative of Arccot
To find the derivative of arccot x, let us assume that y = arccot x. Then by the definition of inverse cot, cot y = x. Differentiating both sides with respect to x,
-csc2y (dy/dx) = 1
dy/dx = -1/(csc2y) ... (1)
By one of the trigonometric identities, csc2y - cot2y = 1. From this, csc2y = 1 + cot2y = 1 + x2.
Substituting this in (1),
dy/dx = -1 / (1 + x2) (or)
d (arccot x) / dx = -1 / (1 + x2)
Thus, the derivative of arccot x (or) cot-1x (or) inverse cot x is -1 / (1 + x2).
Inverse Trig Derivatives and Integrals
Here is a table with derivatives and integrals of inverse trigonometric functions. This will help you to summarize and memorize the difference between the derivatives and integrals of inverse trig functions.
| Inverse Trig Function | Derivative | Integral |
|---|---|---|
| arcsin x | 1/√1-x² | x arcsin x + √1-x² + C |
| arccos x | -1/√1-x² | x arccos x - √1-x² + C |
| arctan x | 1/(1+x²) | x arctan x - (1/2) ln |x2+1| + C |
| arccsc x | -1/(|x|√x²-1) | x arccsc x + ln |x + √x²-1| + C |
| arcsec x | 1/(|x|√x²-1) | x arcsec x - ln |x + √x²-1| + C |
| arccot x | -1/(1+x²) | x arccot x + (1/2) ln |x2+1| + C |
☛Related Topics:
FAQs on Inverse Trig Derivatives
What are the Formulas of Inverse Trig Derivatives?
The inverse trig derivatives are the derivatives of the inverse trigonometric functions. They can be derived using the formulas of inverse trig functions and differentiation techniques. The most used formulas are:
- d/dx(sin-1x) = 1/√1-x²
- d/dx(cos-1x) = -1/√1-x²
- d/dx(tan-1x) = 1/(1+x²)
How to Memorize Inverse Trig Derivatives?
The derivatives of inverse trig functions are:
- d/dx(sin-1x) = 1/√1-x²
- d/dx(cos-1x) = -1/√1-x²
- d/dx(tan-1x) = 1/(1+x²)
- d/dx(csc-1x) = -1/(|x|√(x²-1))
- d/dx(sec-1x) = 1/(|x|√(x²-1))
- d/dx(cot-1x) = -1/(1+x²)
Here, the derivatives of sin-1x and cos-1x are negatives of each other; the derivatives of tan-1x and cot-1x are negatives of each other; and the derivatives of csc-1x and sec-1x are negatives of each other.
How to Find the Derivatives of Inverse Trigonometric Functions?
To find the derivatives of inverse trigonometric functions, we use implicit differentiation. For example, to find the derivative of sin-1x, we assume that y = sin-1x from which we get sin y = x. Differentiating both sides with respect to x, we get cos y dy/dx = 1. From this, dy/dx = 1/cos y = 1/√1-sin²y = 1/√1-x². Like this, we can derive the derivatives of other inverse trigonometric functions.
What is the Derivative of Sin-14x Using Inverse Trig Derivatives?
We know that d/dx(sin-1x) = 1/√1-x². Using this and also applying the chain rule, d/dx(sin-1x) = 4/√1-16x².
Where Can I Find Inverse Trig Derivatives Calculator?
We can find the derivative of any inverse trig function using this calculator. Click here to get it.
How to Prove Inverse Trig Derivatives?
To prove any inverse trig derivative, we use the chain rule. For example, to find the derivative of cos-1x, we assume that y = cos-1x from which we get cos y = x. Differentiating both sides with respect to x, we get -sin y dy/dx = 1. From this, dy/dx = -1/sin y = -1/√1-cos²y = -1/√1-x².
Why is the Absolute Value Sign in Inverse Trig Derivatives?
We have the absolute value sign in the derivatives of csc-1x and sec-1x. This is because the graph of csc-1x is always decreasing and the graph of csc is always increasing in their domains and hence their derivatives must be always negative and positive respectively. So the absolute value sign is kept around x to keep their derivatives irrespective of x.
- d/dx(csc-1x) = -1/(|x|√(x²-1))
- d/dx(sec-1x) = 1/(|x|√(x²-1))