A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
LearnPracticeDownload

Integration by Partial Fractions

Integration by partial fractions is a method used to decompose and then integrate a rational fraction integrand that has complex terms in the denominator. By using partial fraction, we calculate and decompose the expression into simpler terms so that we can easily calculate or integrate the expression thus obtained.

The basic idea in the integration by partial fractions is to factor the denominator and then decompose them into two different fractions where the denominators are the factors respectively and the numerator is calculated suitably. Let us learn more about the different forms used in integration by partial fractions and also the different methods.

1. What Is Integration by Partial Fractions?
2.
3. Method of Integration by Partial Fractions
4. Solved Examples on Integration by Partial Fractions
5. Practice Questions
6. FAQs on Integration by Partial Fractions

What is Integration by Partial Fractions?

Integration by partial fractions is one of the three methods of integration. In this method, we decompose the proper rational fraction into a sum of simpler rational fractions. It is always possible to decompose the rational fraction into simpler rational fractions and this is done by a process called partial fraction decomposition. Let us understand this with the help of an example. Suppose we have 5/6, we can decompose it as 5/6 = 1/2 + 1/3, similarly, we do this by decomposing two partial fractions algebrically. Suppose we have:

2/(x+1) - 1/x

on adding we will get

2/(x+1) - 1/x = (x-1)/(x2+x).

Now if we have

(x-1)/(x2+x)

so we can decompose it into

(x-1)/(x2+x) = 2/(x+1) - 1/x

Thus the partial fractions have been decomposed into simpler terms. So now integrating the resultant terms would be a relatively easy task. Integration by partial fractions would be:

∫[f(x)/g(x)]dx = ∫[p(x)/q(x)]dx + ∫[r(x)/s(x)]dx

where

  • f(x)/g(x) = p(x)/q(x) + r(x)/s(x) and
  • g(x) = q(x).s(x)

Forms Used in Integration by Partial Fractions

In integration by partial fraction, we can use some particular type of partial fractions to decompose specific forms of proper rational fractions. Using these forms we can easily integrate fractions that are in similar forms as given in the following table.

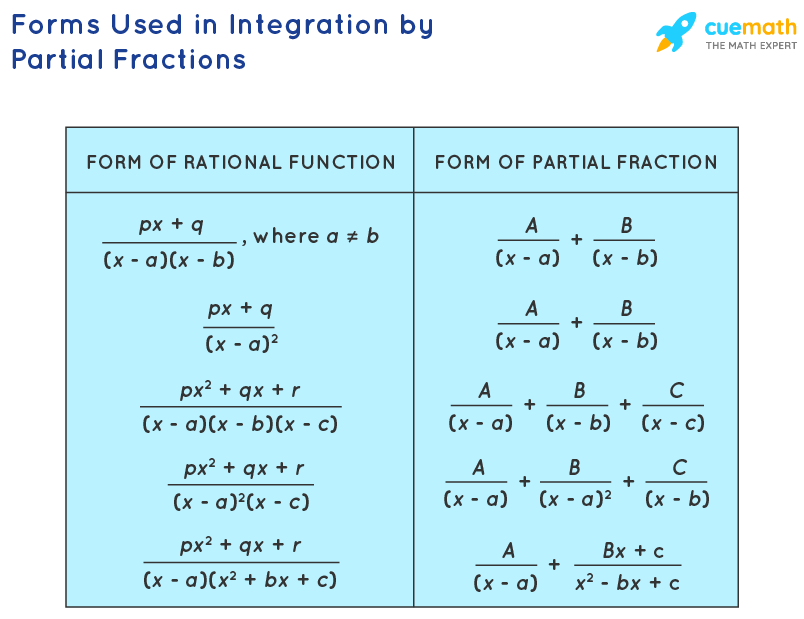
integration by partial fractions

 

Method of Integration by Partial Fractions

The method of integration by partial fractions is a simple process. Let us understand the method of integration by partial fractions with an example. We have:

∫[6/(x2-1)]dx

Since we know: x2-1 = (x+1)(x-1)

Hence we can write:

∫[6/(x2-1)]dx = ∫[6/(x+1)(x-1)]dx

Now by using the form of partial fraction for this kind of rational form, we get:

6/(x+1)(x-1) = A/(x-1) + B/(x+1)

Now, we have to find the value of A and B, making a common denominator on both sides.

6/(x+1)(x-1) = [A/(x-1)][(x+1)/(x+1)] + [B/(x+1)][(x-1)/(x-1)]

6/(x+1)(x-1)= [A(x+1) + B (x-1)]/(x-1)(x+1)

Further we have the denominators on both the sides as equal, and hence the numerators will also be equal.

6 = [A(x+1) + B (x-1)]

On solving we get,

A = 3, and B = -3

Hence, we can write

6/(x+1)(x-1) = 3/(x-1) + (-3)/(x+1)

Now, we can write:

∫[6/(x2-1)]dx = ∫[3/(x-1) - 3/(x+1)]dx

On solving, we will get:

∫[6/(x2-1)]dx = −3ln(|x+1|)+3ln(|x−1|)+C

 

Integration by Partial Fractions Examples

  1. Example 1:

    Integrate using integration by partial fractions: ∫[x+1]/x(1+xex)2dx

    Solution: Observe that the derivative of xex is (x+1)ex. Thus, we could substitute xex for a new variable t if we multiply the numerator and denominator of the expression above by ex:

    I = ∫[x+1]/x(1+xex)2dx.

    =∫(x+1)ex/xex(1+xex)2dx.

    The substitution xex = t now reduces I to:

    I = ∫dt/(t(1+t)2).dt

    We can now expand this expression in t using partial fractions:

    1/(t(1+t)2) = A/t+B/(1+t) + C/(1+t)2

    ⇒1 = A(1+t)2 + B(1+t)t + Ct

    Put t = 0 ⇒ A=1

    Put t = −1 ⇒C = −1

    Compare the coefficient of t2 ⇒ 0 = A+B

    ⇒ B = −1

    The partial fraction expansion is:

    1/t−1/(1+t) − 1/(1+t)2

    Therefore, I is

    I= ln|t| − ln|1+t| + 1/(1+t) + C

    Answer: ∫[x+1]/x(1+xex)2dx = ln|t| − ln|1+ xex| + 1/(1+ xex) + C.

  2. Example 2:

    Integrate using integration by partial fractions: ∫[3x2 + x + 3]/[(x−1)3(x2+1)]dx

    Solution:

    We again find out the partial fraction expansion of the given expression:

    [3x2+x+3]/[(x−1)3(x2+1)] = A/(x−1) + B/ (x−1)2 + C/(x−1)3 + (Dx+E)/(x2+1)

    We cross multiply to obtain

    3x2+x+3 = A(x−1)2(x2+1) + B(x−1)(x2+1) + C(x2+1) + (Dx+E)(x−1)3

    Put x = 1 ⇒ C = 72 ... (1)

    Compare the coefficient of x4 ⇒ 0 = A + D. ..(2)

    Compare the coefficient of x3 ⇒ 0 = −2A + B −3D + E ...(3)

    Compare the coeff of x2 ⇒ 3 = 2A−B+C+3D−3E ...(4)

    Compare the coeff of x ⇒ 1 = −2A + B − D + 3E ...(5)

    Adding (4) and (5), we obtain

    4 = C + 2D

    ⇒ D = 1/4 (from (1))

    ⇒ A = −1/4 (from (2))

    Adding (3) and (4), we obtain

    3 = C − 2E

    ⇒ E = 1/4 (again, using (1))

    Finally, from (5), B = 0

    The partial fraction expansion is therefore,

    (−1/4)/(x−1) + (7/2)/(x−1)3 + [(1/4)x+(1/4)]/(x2+1)

    The integral is

    I = (−1/4)∫[1/(x−1)]dx + 7/2 ∫[1/(x−1)3]dx + 1/4∫[x/(x2+1)]dx + 1/4∫[1/(x2+1)]dx

    = −1/4 ln|x−1| − 7/4(x−1)2 + 1/8 ln(x2+1) + 1/4 tan−1x + C

    = (1/4) {tan−1x − 7/(x−1)2 + ln((√(x2+1))/(|x−1|))} + C

    Answer: ∫[3x2 + x + 3]/[(x−1)3(x2+1)]dx = (1/4){tan−1x − 7/(x−1)2 + ln((√(x2+1))/(|x−1|))} + C

go to slidego to slide

Breakdown tough concepts through simple visuals.
Math will no longer be a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

FAQs on Integration by Partial Fractions

How to do Integration by Partial Fractions?

Integration by partial fractions is a method used to decompose and then integrate a rational fraction integrand that has complex terms in the denominator. By using partial fraction, we can calculate and decompose the expression into simpler terms so that we can easily calculate or integrate the expression thus obtained.

When to Use Integration by Partial Fractions?

The basic idea in the integration by partial fractions is to factor the denominator and then decompose them into two different fractions where the denominators are the factors respectively and the numerator is calculated suitably. Hence we use this method when the degree of the denominator is more than the numerator and the denominator is a complicated expression.

What are the Applications of Integration by Partial Fraction?

Major applications of the method integration by partial fractions include: Integrating rational fraction in Calculus. Finding the Inverse Laplace Transform in the theory of differential equations. It is mostly used to decompose the fraction into two or more different fractions

What is the Difference between Integration by Partial Fraction and Integration by Substitution?

If you observe that a fraction in which substitution will lead to a derivative and will make your question in an integrable form with ease then go for integration by substitution. On the other hand, if it is observed that the given fraction is either a complicated fraction, which can be split into two or more fractions, then we go for Integration by a partial fractions.

Math worksheets and
visual curriculum