Derivative Rules
Derivative rules are used to differentiate different types of functions. All these rules are basically derived from the "derivative using first principle" (limit definition of the derivative). Since using the limit definition is difficult, the derivative rules that are derived from the limit definition are very helpful in making the process of differentiation very easier.
Let us see the derivative rules along with their proofs and many more examples.
| 1. | What are Derivative Rules? |
| 2. | Differentiation Rules of Different Functions |
| 3. | Important Derivative Rules |
| 4. | Derivatives Rules Table |
| 5. | FAQs on Derivative Rules |
What are Derivative Rules?
Derivative rules in Calculus are used to find the derivatives of different operations and different types of functions such as power functions, logarithmic functions, exponential functions, etc. Some important derivative rules are:
- Power Rule
- Sum/Difference Rule
- Product Rule
- Quotient Rule
- Chain Rule
All these rules are obtained from the limit definition of the derivative by which the derivative of a function f(x) (denoted by f '(x)) is given by the limit: f '(x) = limₕ→₀ [f(x + h) - f(x)] / h. The derivative of a function y = f(x) is written as f'(x) (or) dy/dx (or) d/dx (f(x)) and it gives the slope of the curve at a fixed point. It also gives the rate of change of a function with respect to a variable. Let us study each of the differentiation rules in detail in the upcoming sections.
Differentiation Rules of Different Functions
Let us see the differentiation rules of different types of functions. If you want to see the proof of each derivative rule, click on the respective link.
Derivative Rules of Exponential Functions
The exponential function is a function whose base is a constant and whose exponent is a variable. There are mainly two types of exponential functions: ex and ax, where 'e' is Euler's number and 'a' is any constant. We will see the rules for the derivatives of exponential functions.
- The derivative of ex is, d/dx (ex) = ex.
- The derivative of ax is, d/dx (ax) = ax ln a.
Derivative Rules of Logarithmic Functions
A logarithmic function involves a logarithm (either common or natural logarithm). i.e., it is of the form loga x (or) ln x. The rules for finding the derivatives of these two logarithmic functions are:
- The derivative of loga x is, d/dx (loga x) = 1 / (x ln a)
- The derivative of ln x is, d/dx (ln x) = 1/x.
Derivative Rules of Trigonometric Functions
We have six trigonometric functions: sin, cos, tan, csc, sec, and cot. Here are the rules to find the derivatives of trigonometric functions.
- The derivative of sin x is, d/dx (sin x) = cos x
- The derivative of cos x is, d/dx (cos x) = - sin x
- The derivative of tan x is, d/dx (tan x) = sec2x
- The derivative of csc x is, d/dx (csc x) = - csc x cot x
- The derivative of sec x is, d/dx (sec x) = sec x tan x
- The derivative of cot x is, d/dx (cot x) = - csc2x
Derivative Rules of Inverse Trigonometric Functions
There are 6 inverse trigonometric functions corresponding to the above trigonometric functions. Remember that the derivatives of inverse trigonometric functions do NOT involve any trigonometric/inverse trigonometric functions. Here are the rules:
- The derivative of inverse sine is, d/dx (sin-1x) = 1/√(1-x2)
- The derivative of inverse cosine is, d/dx (cos-1x) = -1/√(1-x2)
- The derivative of inverse tan is, d/dx (tan-1x) = 1/(1 + x2)
- The derivative of inverse cosec is, d/dx (csc-1x) = -1/ [x √(x2 - 1) ], x ≠ 1, -1, 0
- The derivative of inverse sec is, d/dx (sec-1x) = 1/ [x √(x2 - 1) ], x ≠ 1, -1, 0
- The derivative of inverse cot is, d/dx (cot-1x) = -1/(1 + x2)
Derivative Rules of Hyperbolic Functions
There are 6 hyperbolic functions corresponding to 6 trigonometric functions. Each trigonometric function followed by a "h" is its corresponding hyperbolic function. Here are the derivatives of hyperbolic functions.
- The derivative of sinh x is, d/dx (sinh x) = cosh x
- The derivative of cosh x is, d/dx (cosh x) = sinh x
- The derivative of tanh x is, d/dx (tanh x) = sech2 x
- The derivative of csch x is, d/dx (csch x) = - csch x coth x
- The derivative of sech x is, d/dx (sech x) = - sech x tanh x
- The derivative of coth x is, d/dx (coth x) = - csch2 x
Derivative Rules of Inverse Hyperbolic Functions
There are again 6 inverse hyperbolic functions that correspond to 6 hyperbolic functions. Here are the rules to find their derivatives.
- The derivative of sinh-1x is, d/dx (sinh-1x) = 1/√(1+x2)
- The derivative of cosh-1x is, d/dx (cosh-1x) = 1/√(x2-1), x>1
- The derivative of tanh-1x is, d/dx (tanh-1x) = 1/(1-x2), |x|<1
- The derivative of csch-1x is, d/dx (csch-1x) = -1/ [ |x| √(1-x2) ], x ≠ 0
- The derivative of sech-1x is, d/dx (sech-1x) = -1/ [ x√(1-x2) ], 0 < x < 1
- The derivative of coth-1x is, d/dx (coth-1x) = 1/(1-x2), |x|>1
Important Derivative Rules
Here are very important rules of differentiation such as the product rule, quotient rule, sum rule, difference rule, etc, and these rules are used for different combinations of the above-mentioned functions. Let us also see some examples of each rule.
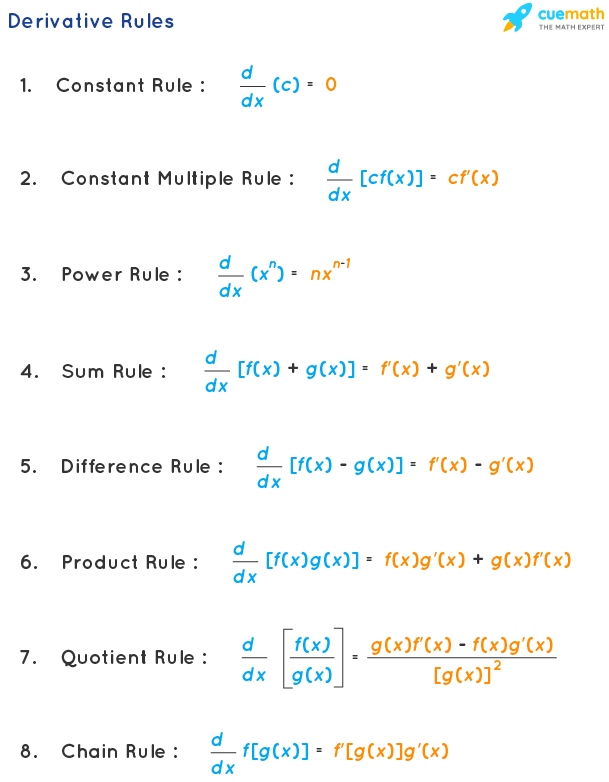
Power Rule of Derivatives
The power rule of derivatives says d/dx (xn) = n · xn - 1. Here are some examples for the application of this rule.
- d/dx (x2) = 2x2 - 1 = 2x
- d/dy (y5) = 5y5 - 1 = 5y4
Using this rule, we derive two things:
- The derivative of x with respect to itself is 1. i.e., d/dx (x) = 1.
This is because
d/dx (x) = d/dx (x1) = 1 x1-1 = 1x0 = 1. - The derivative of a constant function is 0. i.e., d/dx (c) = 0, where 'c' is a constant (This rule is said to be constant rule).
This is because
d/dx (c) = d/dx (c x0) = c d/dx (x0) = c (0 x0-1) = 0
Why did we write 'c' out of differentiation here? This is because of the following rule.
Constant Multiple Rule of Derivatives
The constant multiple rule of derivatives says that d/dx (c f(x)) = c d/dx (f(x)). It means that if a constant is getting multiplied by a function, then that constant doesn't participate in the differentiation process and it comes out. For example:
- d/dx (2x3) = 2 d/dx(x3) = 2(3x2) = 6x2
- d/dx (4x) = 4 d/dx (x) = 4 d/dx (x) = 4(1) = 4.
Here are two examples to avoid common confusion when a constant is involved in differentiation.
- d/dx (4 + x) = d/dx (4) + d/dx (x) = 0 + 1 = 0
- d/dx (4x) = 4 d/dx (x) = 4(1) = 4
Why did we split d/dx for 4 and x in d/dx (4 + x) here? Let's see the rule behind it.
Sum/Difference Rule of Derivatives
This rule says, the differentiation process can be distributed to the functions in case of sum/difference. i.e., d/dx (f(x) ± g(x)) = d/dx (f(x)) ± d/dx (g(x)). Here are some examples for the application of this rule.
- d/dx (x3 + x2) = d/dx (x3) + d/dx (x2) = 3x2 + 2x
- d/dx (x3 - x2) = d/dx (x3) - d/dx (x2) = 3x2 - 2x
Product Rule of Derivatives
The product rule is used to find the derivative of product of two functions. It says d/dx (f(x)) · g(x)) = f(x) d/dx (g(x)) + g(x) d/dx (f(x)). In other words, it can be written as (uv)' = u v' + v u'. We can understand this rule by the following examples.
- d/dx (x sin x) = x d/dx (sin x) + sin x d/dx (x) = x cos x + sin x (1) = x cos x + sin x
- d/dx (x2 ln x) = x2 d/dx (ln x) + ln x d/dx (x2) = x2 (1/x) + ln x (2x) = x + 2x ln x
Quotient Rule of Derivatives
The quotient rule of derivatives is to find the derivative of a fraction where both numerator and denominator involve variable. The quotient rule says \(\frac{d}{d x}\left[\frac{f(x)}{g(x)}\right]=\frac{f^{\prime}(x) g(x)-f(x) g^{\prime}(x)}{[g(x)]^{2}}\). In other words, the quotient rule says d/dx (u/v) = (v u' - u v') / v2. Here are some examples.
- d/dx (x / sin x) = [ sin x d/dx (x) - x d/dx (sin x) ] / (sin x)2 = (sin x - x cos x) / sin2x.
- d/dx (x / ln x) = [ ln x d/dx (x) - x d/dx (ln x) ] / (ln x)2 = (ln x - x(1/x)) / (ln x)2 = (ln x - 1) / (ln x)2.
Chain Rule of Derivatives
The chain rule of derivatives is used to differentiate a composite function, or in other words, chain rule is used to find the derivative of a function that is inside the other function. For example, it can be used to differentiate functions such as sin (x2), ln (2x + 1), tan (ln x), etc. The chain rule says d/dx (f(g(x)) = f ' (g(x)) · g'(x). This rule just means that we find the derivative found by using derivative rules by the derivative of the inside function. For example:
- d/dx (sin (x2)) = cos (x2) · d/dx (x2) = 2x cos (x2)
- d/dx (tan (ln x)) = sec2 (ln x) · d/dx (ln x) = sec2 (ln x)/x
Derivative Rule of Inverse Functions
The inverse of a function f(x) is denoted by f-1(x). Here is the rule to find the derivative of the inverse function which says : \(\left[f^{-1}\right]^{\prime}(a)=\frac{1}{f^{\prime}\left[f^{-1}(a)\right]}\). Here is an example: Find (f-1)(1) if f(x) = x3 - 7. Let us apply the rule to find this derivative. Then we get:
\(\left[f^{-1}\right]^{\prime}(1)=\frac{1}{f^{\prime}\left[f^{-1}(1)\right]}\) ... (1)
To find f-1(1), assume that f-1(1) = x ⇒ 1 = f(x) ⇒ 1 = x3 - 7 ⇒ x3 = 8 ⇒ x = 2.
So f-1(1) = 2 ... (2)
Now, f '(x) = 3x2 - 0 = 3x2.
Then f '(f-1(1)) = f ' (2) (from (2))
= 3(2)2
= 12
Substituting this in (1):
(f-1)(1) = 1 / 12.
Derivative Rules of Tangent and Normal
A tangent line of a curve, y = f(x) is a line that touches the curve at one point. A normal of a tangent is a line perpendicular to the tangent. The slopes of tangent and normal can be found using the derivative.
- The slope of a tangent line is f ' (x)
- The slope of a normal line is - 1/ f ' (x)
Derivatives Rules Table
The following table includes the important derivatives rules.
| Function in x | Derivative |
|---|---|
| c (constant) | 0 |
| x | 1 |
| xn | n xn-1 |
| ex | ex |
| ax | ax ln a |
| loga x | 1 / (x ln a) |
| ln x | 1/x |
| sin x | cos x |
| cos x | - sin x |
| tan x | sec2x |
| csc x | - csc x cot x |
| sec x | sec x tan x |
| cot x | - csc2x |
| sin-1x | 1/√(1-x2) |
| cos-1x | -1/√(1-x2) |
| tan-1x | 1/(1 + x2) |
| csc-1x | -1/ [x √(x2 - 1) ] |
| sec-1x | 1/ [x √(x2 - 1) ] |
| cot-1x | -1/(1 + x2) |
Here is the table with important differentiation rules that are helpful in finding the derivatives of complex functions.
| Name of the Rule | Differentiation Rule |
|---|---|
| Constant Multiple Rule | d/dx (c f(x)) = c d/dx (f(x)) |
| Sum/Difference Rule | d/dx (f(x) ± g(x)) = d/dx (f(x)) ± d/dx (g(x)) |
| Product Rule | d/dx (f(x)) · g(x)) = f(x) d/dx (g(x)) + g(x) d/dx (f(x)) |
| Quotient Rule | \(\frac{d}{d x}\left[\frac{f(x)}{g(x)}\right]=\frac{f^{\prime}(x) g(x)-f(x) g^{\prime}(x)}{[g(x)]^{2}}\) |
| Chain Rule | d/dx (f(g(x)) = f ' (g(x)) · g'(x) |
| Derivative of Inverse Function | \(\left[f^{-1}\right]^{\prime}(a)=\frac{1}{f^{\prime}\left[f^{-1}(a)\right]}\) |
☛Related Links:
Examples on Derivative Rules
-
Example 1: Find the derivative of the function: f(x) = (x + a) / (x + b).
Solution:
Let f(x) = (x + a) / (x + b) = u/v
By quotient rule of derivatives,
(u/v)' = (vu' - uv')/v2
Using this,
f'(x) = [ (x + b) d/dx (x + a) - (x + a) d/dx (x + b)] / (x + b)2
= [ (x + b) (1) - (x + a) (1)] / (x + b)2
= (x + b - x - a) / (x + b)2
= (b - a) / (x + b)2Answer: The derivative of the given function is (b - a) / (x + b)2.
-
Example 2: By using the derivative rules, find the derivative of f(x) = (ax2 + b)2.
Solution:
The given function is, f(x) = (ax2 + b)2. Here, one function is inside the other (composite function). So we will apply the chain rule.
f'(x) = 2(ax2 + b) d/dx (ax2 + b)
= 2(ax2 + b) (2ax)
= 4ax(ax2 + b)Answer: The derivative is 4ax(ax2 + b).
-
Example 3: Find the derivative of f(x) = x sin-1x using the differentiation rules.
Solution:
The given function is f(x) = x sin-1x = u v.
Since this is a product of two different functions, we can apply the product rule here.
(uv)' = uv' + vu'
f'(x) = x d/dx (sin-1x) + sin-1x d/dx (x)
= x (1/√(1-x2) ) + sin-1x (1)
= x/√(1-x2) + sin-1xAnswer: The derivative is x/√(1-x2) + sin-1x.

FAQs on Derivative Rules
What are the Four Basic Derivative Rules?
The four basic derivative rules are:
- Derivative rule of sum: (u + v) ' = u' + v'
- Derivative rule of difference: (u - v) ' = u' - v'
- Product rule derivative: (uv)' = u v' + v u'
- Quotient rule derivative: (u/v)' = (vu' - uv')/v2
How to Use Derivative Rules to Find the Derivative of Square Root?
We know that a square root can be replaced with the exponent 1/2. i.e., √x can be written as x1/2 and hence we can apply the power rule to differentiate this. Using this, d/dx (√x) = d/dx (x1/2) = (1/2) x-1/2 = 1/(2√x).
What are Differentiation Rules?
The differentiation rules (also known as derivative rules) in Calculus are the rules that are used for finding derivatives. There are different differentiation rules such as product rule, quotient rule, power rule, etc. Also, we have different differentiation rules to find the derivatives of logarithmic functions, exponential functions, trigonometric functions, etc.
What are Derivative Rules of e?
The derivative rules that involve "e" are:
- d/dx (ex) = ex
- d/dx (eax) = a eax
What are Differentiation Rules Trig?
The differentiation rules in trigonometry are the formulas to find the derivatives of sin x, cos x, tan x, csc x, sec x, and cot x. They are:
- (sin x)' = cos x
- (cos x)' = - sin x
- (tan x)' = sec2x
- (csc x)' = - csc x cot x
- (sec x)' = sec x tan x
- (cot x)' = - csc2x
What are the Applications of Derivative Rules?
Here are the applications of differentiation rules.
- Finding the maxima and minima.
- Finding critical points.
- Finding the inflection points.
- Finding the instantaneous rate of change.
- Finding the intervals of increase/decrease of a function.
What are ln Derivative Rules?
The derivative rules that involve logarithms are:
- d/dx (log x) = 1 / (x ln 10)
- d/dx (ln x) = 1/x
visual curriculum