Derivative of Hyperbolic Functions

Before getting into the details of the derivative of hyperbolic functions, let us recall the concept of the hyperbolic functions. Hyperbolic functions are functions in calculus that are expressed as combinations of the exponential functions e^x and e^-x. We have six main hyperbolic functions given by, sinhx, coshx, tanhx, sechx, cothx, and cschx. The derivative of hyperbolic functions is calculated using the derivatives of exponential functions formula and other hyperbolic functions formulas and identities.

In this article, we will evaluate the derivatives of hyperbolic functions using different hyperbolic trig identities and derive their formulas. We will also explore the graphs of the derivative of hyperbolic functions and solve examples and find derivatives of functions using these derivatives for a better understanding of the concept.

1.	What is Derivative of Hyperbolic Functions?
2.	Derivative of Hyperbolic Functions Formula
3.	Derivatives of Hyperbolic Functions Proof
4.	Derivative of Inverse Hyperbolic Functions
5.	Derivatives of Hyperbolic Functions and Inverse Hyperbolic Functions Table
6.	FAQs on Derivative of Hyperbolic Functions

The derivative of hyperbolic functions gives the rate of change in the hyperbolic functions as differentiation of a function determines the rate of change in function with respect to the variable. We can evaluate these derivatives using the derivative of exponential functions e^x and e^-x along with other hyperbolic functions formulas and identities. We have six main hyperbolic functions namely,

• Sinhx
• Coshx
• Tanhx
• Cothx
• Sechx
• Cschx

The derivative of hyperbolic functions is used in describing the shape of electrical wires hanging freely between two poles. They are also used to describe any freely hanging cable between two ends. Among other applications, the derivative of hyperbolic functions is used to describe the formation of satellite rings and planets. In the next section, we will explore the formulas of the derivatives of hyperbolic functions.

Now, we will go through the formulas of the derivatives of hyperbolic functions. The hyperbolic functions are combinations of exponential functions e^x and e^-x. Given below are the formulas for the derivative of hyperbolic functions:

• Derivative of Hyperbolic Sine Function: d(sinhx)/dx = coshx
• Derivative of Hyperbolic Cosine Function: d(coshx)/dx = sinhx
• Derivative of Hyperbolic Tangent Function: d(tanhx)/dx = sech²x
• Derivative of Hyperbolic Cotangent Function: d(cothx)/dx = -csch²x (x ≠ 0)
• Derivative of Hyperbolic Secant Function: d(sechx)/dx = -sechx tanhx
• Derivative of Hyperbolic Cosecant Function: d(cschx)/dx = -cschx cothx (x ≠ 0)

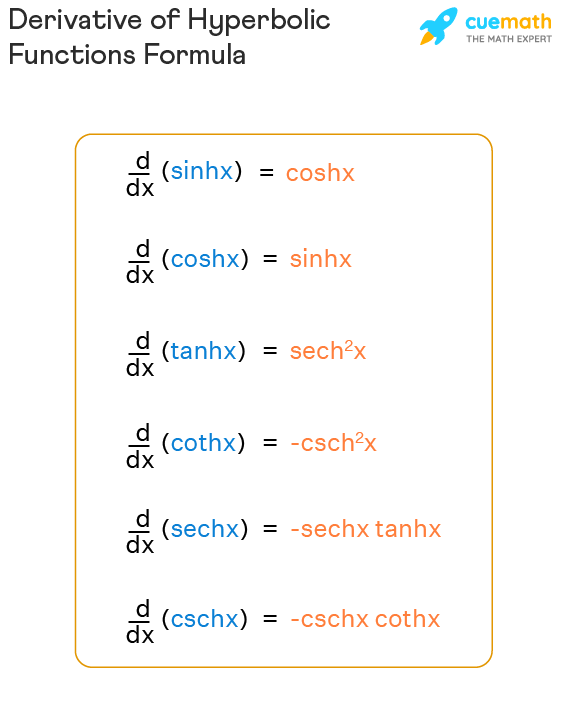
Let us now prove these derivatives using different mathematical formulas and identities.

Now that we know the formulas for the derivatives of hyperbolic functions, let us now prove them using various formulas and identities of hyperbolic functions. We will use the following formulas to prove the derivative of hyperbolic functions:

• Derivative of e to the power x: d(e^x)/dx = e^x
• Derivative of e to the power negative x: d(e^-x)/dx = -e^-x

Derivative of Sinhx

We know that the formula for sinhx is given by, sinhx = (e^x - e^-x)/2. To find the derivative of hyperbolic function sinhx, we will write as a combination of exponential function and differentiate it using the quotient rule of differentiation. Also, we know that we can write the hyperbolic function cosh x as cosh x = (e^x + e^-x)/2. So, using these formulas, we have

d(sinhx)/dx = d[(e^x - e^-x)/2] / dx

= [ (e^x - e^-x)' 2 - (e^x - e^-x) (2)' ] / 2²

= [e^x - (-e^-x)] 2 / 2² --- [Using d(e^x)/dx = e^x and d(e^-x)/dx = -e^-x]

= (e^x + e^-x)/2

= cosh x

Therefore, the derivative of sinh x is equal to cosh x.

Derivative of Coshx

To prove the derivative of coshx, we will use the following formulas:

• sinhx = (e^x - e^-x)/2
• cosh x = (e^x + e^-x)/2
• d(e^x)/dx = e^x
• d(e^-x)/dx = -e^-x

Using the above formulas, we have

d(coshx)/dx = d[(e^x + e^-x)/2] / dx

= d(e^x/2) / dx + d(e^-x/2) / dx

= e^x/2 - e^-x/2

= (e^x - e^-x)/2

= sinhx

Hence, we have proved that the derivative of coshx is equal to sinhx.

Derivative of Tanhx

Using hyperbolic functions formulas, we know that tanhx can be written as the ratio of sinhx and coshx. So, we will use the quotient rule and the following formulas to find the derivative of tanhx:

• tanhx = sinhx / coshx
• d(sinhx)/dx = coshx
• d(coshx)/dx = sinhx
• cosh²x - sinh²x = 1
• 1/coshx = sechx

Using the above formulas, we have

d(tanhx)/dx = d(sinhx / coshx)/dx

= [(sinhx)² coshx - (coshx)' sinhx] / cosh²x --- [Using quotient rule of derivatives]

= (cosh²x - sinh²x) / cosh²x

= 1 / cosh²x

= sech²x

Hence, the derivative of hyperbolic function tanhx is equal to sech²x.

Derivative of Cothx

Just like we derived the derivative of tanhx, we will evaluate the derivative of hyperbolic function cothx using the quotient rule. Also, we can express cothx as the ratio of coshx and sinhx. We will use the following formulas to calculate the derivative of tanhx:

• cothx = coshx/sinhx
• d(sinhx)/dx = coshx
• d(coshx)/dx = sinhx
• cosh²x - sinh²x = 1
• 1/sinhx = cschx

So, we have

d(cothx)/dx = d(coshx/sinhx)/dx

= [(coshx)' sinhx - (sinhx)' coshx] / sinh²x

= (sinh²x - cosh²x) / sinh²x

= -(cosh²x - sinh²x) / sinh²x

= -1 / sinh²x

= - csch²x

Therefore, the derivative of cothx is equal to - csch²x.

Derivative of Sechx

In this section, we will derive the formula for the derivative of sechx using the quotient rule. We will use the formula for the derivative of coshx along with other formulas given by,

• d(coshx)/dx = sinhx
• sechx = 1/coshx
• tanhx = sinhx/coshx

Using the above formulas, we have

d(sechx)/dx = d(1/coshx)/dx

= [(1)' coshx - (coshx)' (1)] / cosh²x

= (0 × coshx - sinhx) / cosh²x

= -sinhx / cosh²x

= - (sinhx / coshx) × (1/coshx)

= - tanhx sechx

Hence, the derivative of hyperbolic function sechx is equal to - tanhx sechx.

Derivative of Cschx

To find the derivative of cschx, we will use a similar method as we used to find the derivative of sechx. We will use the following formulas to find the derivative of cschx:

• d(sinhx)/dx = coshx
• 1/sinhx = cschx
• cothx = coshx/sinhx

So, we have

d(cschx)/dx = d(1/sinhx)/dx

= [(1)' sinhx - (sinhx)' (1)] / sinh²x

= (0 × sinhx - coshx) / sinh²x

= -coshx / sinh²x

= - (coshx / sinhx) × (1/sinhx)

= - cothx cschx (x ≠ 0)

Hence, we have proved that the derivative of cschx is equal to - cothx cschx.

Now that we have derived the derivative of hyperbolic functions, we will derive the formulas of the derivatives of inverse hyperbolic functions. We can find the derivatives of inverse hyperbolic functions using the implicit differentiation method. We have six main inverse hyperbolic functions, given by arcsinhx, arccoshx, arctanhx, arccothx, arcsechx, and arccschx. Their derivatives are given by:

• Derivative of arcsinhx: d(arcsinhx)/dx = 1/√(x² + 1), -∞ < x < ∞
• Derivative of arccoshx: d(arccoshx)/dx = 1/√(x² - 1), x > 1
• Derivative of arctanhx: d(arctanhx)/dx = 1/(1 - x²), |x| < 1
• Derivative of arccothx: d(arccothx)/dx = 1/(1 - x²), |x| > 1
• Derivative of arcsechx: d(arcsechx)/dx = -1/x√(1 - x²), 0 < x < 1
• Derivative of arccschx: d(arccschx)/dx = -1/|x|√(1 + x²), x ≠ 0

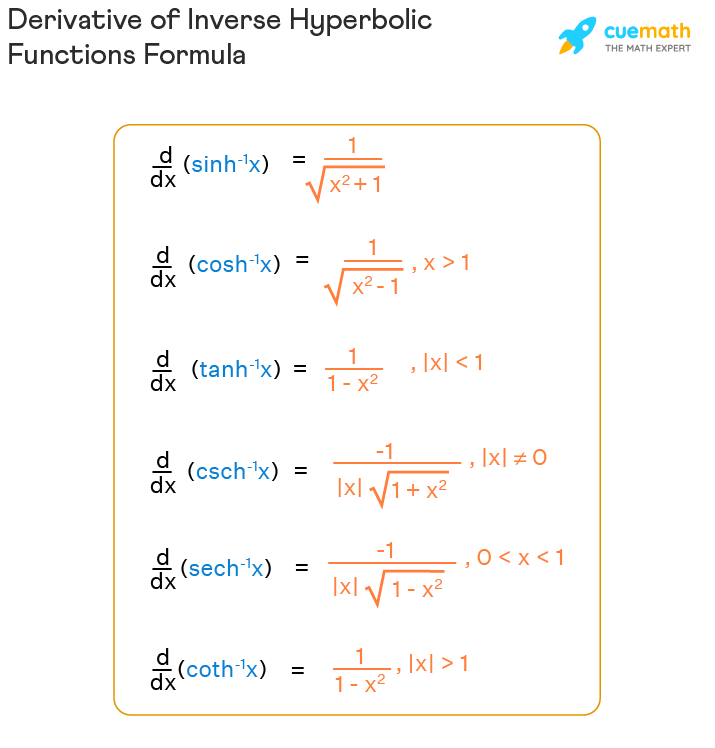
Now, let use derive the above formulas of derivatives of inverse hyperbolic functions using implicit differentiation method.

Derivative of Arcsinhx

Assume arcsinhx = y, then we have x = sinh y. Now, differentiating both sides of x = sinh y with respect to x, we have:

dx/dx = d(sinh y)/dx

⇒ 1 = cosh y × dy/dx --- [Because derivative of sinh y is cosh y]

⇒ dy/dx = 1/coshy

= 1/√(1 + sinh²y) --- [Because cosh²A - sinh²A = 1 which implies coshA = √(1 + sinh²A)]

= 1/√(1 + x²) --- [Because x = sinh y]

⇒ d(arcsinhx)/dx = 1/√(1 + x²)

Derivative of Arccoshx

To find the derivative of arccoshx, we assume arccoshx = y. This implies we have x = cosh y. Now, differentiating both sides of x = cosh y, we have

dx/dx = d(cosh y)/dx

⇒ 1 = sinh y × dy/dx --- [Because derivative of cosh y is sinh y]

⇒ dy/dx = 1/sinhy

= 1/√(cosh²y - 1) --- [Because cosh²A - sinh²A = 1 which implies sinhA = √(cosh²A - 1)]

= 1/√(x² - 1) --- [Because x = cosh y]

⇒ d(arccoshx)/dx = 1/√(x² - 1), x > 1

Derivative of Arctanhx

Next, we will calculate the derivative of tanhx. Assume arctanhx = y, then we have x = tanh y. Now, differentiating both sides of x = tanh y with respect to x, we have

dx/dx = d(tanh y)/dx

⇒ 1 = sech²y × dy/dx --- [Because derivative of tanh y is sech²y]

⇒ dy/dx = 1/sech²y

= 1/(1 - tanh²y) --- [Using hyperbolic trig identity 1 - tanh²A = sech²A]

= 1/(1 - x²) --- [Because x = tanhy]

⇒ d(arctanhx)/dx = 1/(1 - x²), |x| < 1

Derivative of Arccothx

We will find the derivative of arccothx using a similar way as we did for the derivative of arctanhx. Assume arccothx = y, then we have x = coth y. Now, differentiating both sides of x = coth y with respect to x, we have

dx/dx = d(coth y)/dx

⇒ 1 = -csch²y × dy/dx --- [Because derivative of coth y is -csch²y]

⇒ dy/dx = -1/csch²y

= -1/(coth²y - 1) --- [Using hyperbolic trig identity coth²A - 1 = csch²A]

= -1/(x² - 1) --- [Because x = cothy]

⇒ d(arccothx)/dx = -1/(x² - 1) , |x| > 1

Derivative of Arcsechx

To find the derivative of arcsechx, we will use the formula for the derivative of sechx. Assume arcsechx = y, this implies we have x = sech y. Now, differentiating both sides of x = sech y with respect to x, we have

dx/dx = d(sech y)/dx

⇒ 1 = -sech y tanh y × dy/dx --- [Because derivative of sech y is -sech y tanh y]

⇒ dy/dx = -1/sech y tanh y

= -1/sech y √(1 - sech²y) --- [Using hyperbolic trig identity 1 - tanh²A = sech²A which implies tanh A = √(1 - sech²A)]

= -1/x √(1 - x²)

⇒ d(arcsechx)/dx = -1/x √(1 - x²) , 0 < x < 1

Derivative of Arccschx

To find the derivative of arccschx, we will use the formula for the derivative of cschx. Assume arccschx = y, this implies we have x = csch y. Now, differentiating both sides of x = csch y with respect to x, we have

dx/dx = d(csch y)/dx

⇒ 1 = -csch y coth y × dy/dx --- [Because derivative of sech y is -csch y coth y]

⇒ dy/dx = -1/csch y coth y

= -1/csch y √(csch²y + 1)--- [Using hyperbolic trig identity coth²A - 1 = csch²A which implies coth A = ±√(csch²A + 1)]

= -1/|x| √(x² + 1)

⇒ d(arccschx)/dx = -1/|x| √(x² + 1) , x ≠ 0

In the above sections, we have derived the formulas for the derivatives of hyperbolic functions and inverse hyperbolic functions. Let us now summarize all the derivatives in a table below along with their domains (restrictions):

Function	Derivative	Domain
sinhx	coshx	-∞ < x < ∞
coshx	sinhx	-∞ < x < ∞
tanhx	sech2x	-∞ < x < ∞
cothx	-csch2x	x ≠ 0
sechx	-sechx tanhx	-∞ < x < ∞
cschx	-cschx cothx	x ≠ 0
arcsinhx	1/√(x2 + 1)	-∞ < x < ∞
arccoshx	1/√(x2 - 1)	x > 1
arctanhx	1/(1 - x2)	|x| < 1
arccothx	1/(1 - x2)	|x| > 1
arcsechx	-1/x√(1 - x2)	0 < x < 1
arccschx	-1/|x|√(1 - x2)	x ≠ 0

Important Notes on Derivative of Hyperbolic Functions

• d(sinhx)/dx = coshx
• d(coshx)/dx = sinhx
• d(tanhx)/dx = sech²x
• d(cothx)/dx = -csch²x (x ≠ 0)
• d(sechx)/dx = -sechx tanhx
• d(cschx)/dx = -cschx cothx (x ≠ 0)

☛ Related Topics:

• Eccentricity of Hyperbola
• Hyperbola

 

What are Derivatives of Hyperbolic Functions?

The derivative of hyperbolic functions gives the rate of change in the hyperbolic functions as differentiation of a function determines the rate of change in function with respect to the variable. We have six main hyperbolic functions given by, sinhx, coshx, tanhx, sechx, cothx, and cschx.

What is the Derivative of Hyperbolic Functions Formula?

Given below are the formulas for the derivative of hyperbolic functions:

• Derivative of Sinhx: d(sinhx)/dx = coshx
• Derivative of Coshx: d(coshx)/dx = sinhx
• Derivative of Tanhx: d(tanhx)/dx = sech²x
• Derivative of Cothx: d(cothx)/dx = -csch²x (x ≠ 0)
• Derivative of Sechx: d(sechx)/dx = -sechx tanhx
• Derivative of Cschx: d(cschx)/dx = -cschx cothx (x ≠ 0)

How to Prove Derivatives of Hyperbolic Functions?

We can prove the derivative of hyperbolic functions by using the derivative of exponential function along with other hyperbolic formulas and identities. We know that hyperbolic functions are expressed as combinations of e^x and e^-x.

What are Derivatives of Inverse Hyperbolic Functions?

The derivatives of inverse hyperbolic functions are given by:

• Derivative of arcsinhx: d(arcsinhx)/dx = 1/√(x² + 1), -∞ < x < ∞
• Derivative of arccoshx: d(arccoshx)/dx = 1/√(x² - 1), x > 1
• Derivative of arctanhx: d(arctanhx)/dx = 1/(1 - x²), |x| < 1
• Derivative of arccothx: d(arccothx)/dx = 1/(1 - x²), |x| > 1
• Derivative of arcsechx: d(arcsechx)/dx = -1/x√(1 - x²), 0 < x < 1
• Derivative of arccschx: d(arccschx)/dx = -1/|x|√(1 + x²), x ≠ 0

How Do You Take the Derivative of Hyperbolic Function Sinx?

We can find the derivative of sinhx by expressing it as d(sinhx)/dx = (e^x - e^-x)/2. So, we have d(sinhx)/dx = d[(e^x - e^-x)/2] / dx = (e^x + e^-x)/2 = cosh x.