Improper Integral Calculator
The improper integral is the reversing of the process of differentiation. An improper integral is an integral which have an upper limit and a lower limit. The improper integral also find the area under the curve from the lower limit to the upper limit. Improper integral is also known as a definite integral.
What is an Improper Integral Calculator?
An 'Improper Integral Calculator' is a free online tool that helps to calculate the improper integral value for a given function. In this calculator, you can enter the function, upper, and lower limit and the value of improper integral will be displayed within a few seconds.
Improper Integral Calculator
How to Use Improper Integral Calculator?
Follow the steps given below to use the calculator:
- Step 1: Enter the function, upper and lower limit in the space provided.
- Step 2: Click on "Calculate".
- Step 3: Click on "Reset" to clear the field and enter new values.
How to Find an Improper Integral?
Integration is defined as the reverse process of differentiation. The integration is represented by ' ∫ '. Improper integrals are integrals that have upper and lower limits. It is represented as b∫af(x)dx. The fundamental theorem of calculus tells us that to calculate the area under a curve y = f(x) from x = a to x = b, we first calculate the integration g(x) of f(x),
g(x) = ∫ f(x) dx
And then evaluate g(b) - g(a). That is, the area under the curve f(x) from x = a to x = b is
\(\int_{a}^{b} f(x) dx = g(a) - g(b)\)
There are common functions and rules we follow to find the integration.
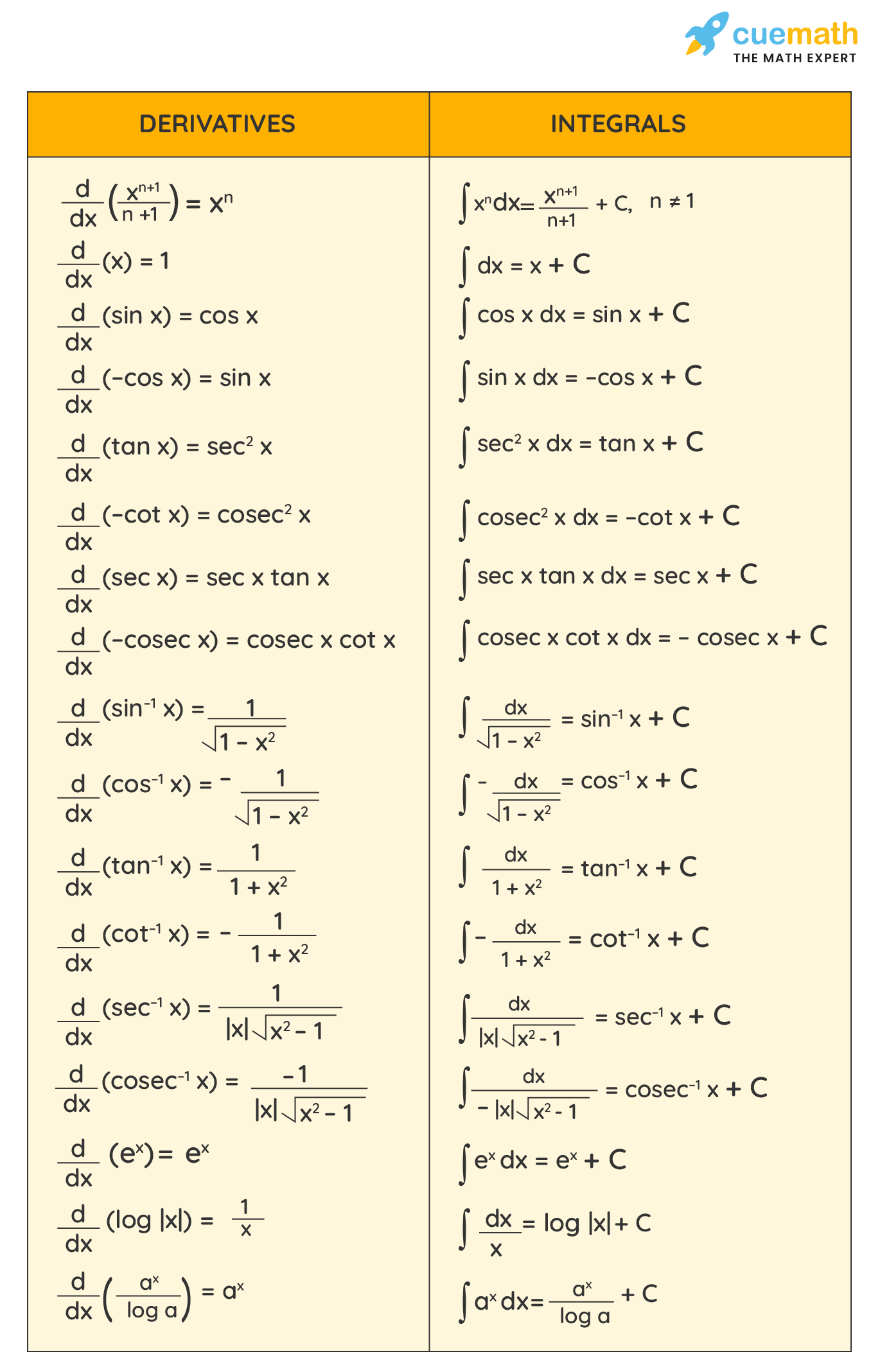
Solved Examples on Improper Integral Calculator
Example 1:
Find the integration of value \(\int_{6}^{9} (2x - 7) dx\) and verify it using improper integral calculator.
Solution:
\(\int_{6}^{9} (2x - 7) dx\)
= \(\int_{6}^{9} 2x dx - \int_{6}^{9} 7 dx\)
= \(x^2|_6^9 - 7x|_6^9\)
= (92 - 62) - 7(9 - 6)
= (81 - 36) - 7(3)
= 45 - 21
= 24
Example 2:
Find the integration of value \(\int_{2}^{3} (5x2) dx\) and verify it using improper integral calculator.
Solution:
\(\int_{2}^{3} (5x2) dx\)
= (5/3)\(x^3|_2^3\)
= 5/3(33 - 23)
= 5/3(27 - 8)
= 5/3(19)
= 95/3
= 31.666