Binomial Expansion Formulas

Before learning binomial expansion formulas, let us recall what is a "binomial". A binomial is an algebraic expression with two terms. For example, a + b, x - y, etc are binomials. We have a set of algebraic identities to find the expansion when a binomial is raised to exponents 2 and 3. For example, (a + b)² = a² + 2ab + b². But what if the exponents are bigger numbers? It is tedious to find the expansion manually. The binomial expansion formula eases this process. Let us learn the binomial expansion formula along with a few solved examples.

What Are Binomial Expansion Formulas?

As we discussed in the earlier section, the binomial expansion formulas are used to find the powers of the binomials which cannot be expanded using the algebraic identities. The binomial expansion formula involves binomial coefficients which are of the form \(\left(\begin{array}{l}n \\k\end{array}\right)\) (or) \(n_{ C_{k}}\) and it is calculated using the formula, \(\left(\begin{array}{l}n \\k\end{array}\right)\) =n! / [(n - k)! k!]. The binomial expansion formula is also known as the binomial theorem. Here are the binomial expansion formulas.

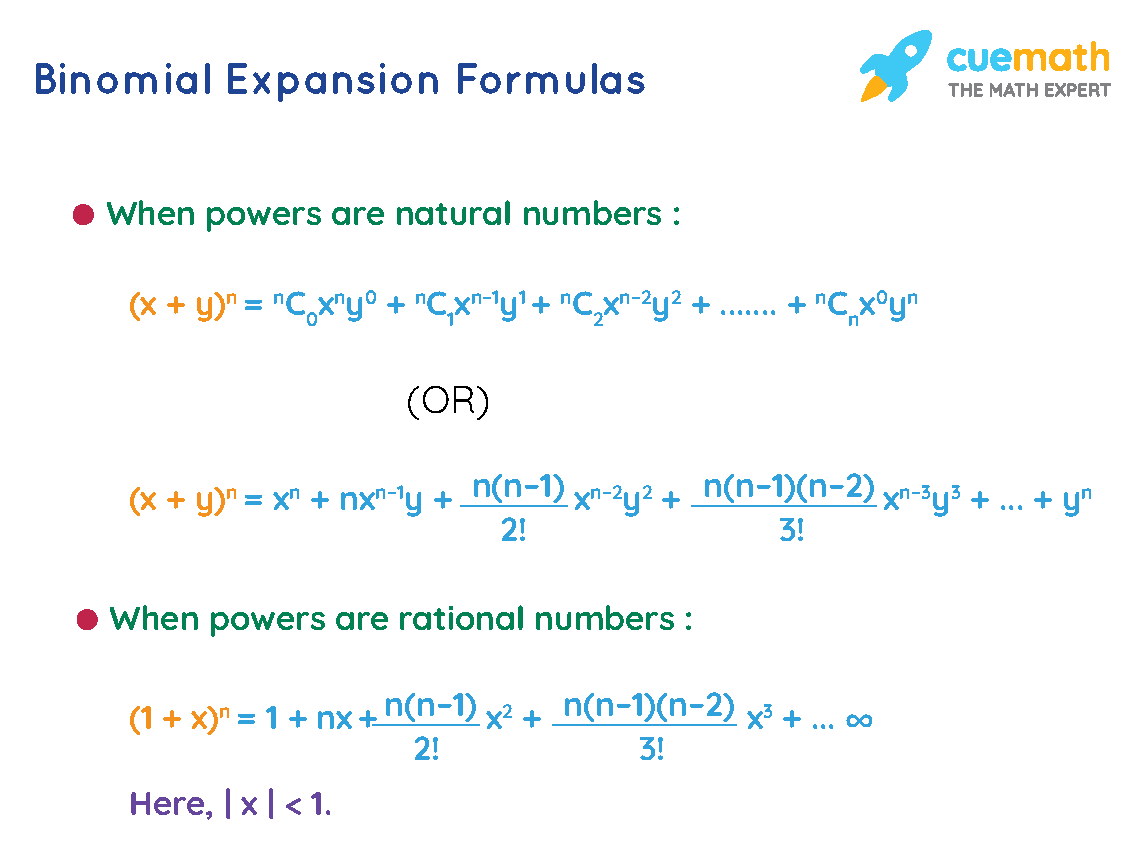
Binomial Expansion Formula of Natural Powers

This binomial expansion formula gives the expansion of (x + y)ⁿ where 'n' is a natural number. The expansion of (x + y)ⁿ has (n + 1) terms. This formula says:

(x + y)ⁿ = ⁿC\(_0\) xⁿ y⁰ + ⁿC\(_1\) x^{n - 1} y¹ + ⁿC\(_2\) x^n-2 y² + ⁿC\(_3\) x^{n - 3} y³ + ... + ⁿC\(_{n-1}\) x y^{n - 1} + ⁿC\(_n\) x⁰yⁿ

Here we use nC\(_k\) formula to calculate the binomial coefficients which says ⁿC\(_k\) = n! / [(n - k)! k!]. By applying this formula, the above binomial expansion formula can also be written as,

(x + y)ⁿ = xⁿ + n x^{n - 1} y¹ + [n(n - 1)/2!] x^n-2 y² +  [n(n - 1)(n - 2)/3!]  x^{n - 3} y³ +... + n x y^{n - 1} + yⁿ

Note: If we observe just the coefficients, they are symmetric about the middle term. i.e., the first coefficient is the same as the last one, the second coefficient is as same as the one that is second from the last, etc.

Binomial Expansion Formula of Rational Powers

This binomial expansion formula gives the expansion of (1 + x)ⁿ where 'n' is a rational number. This expansion has an infinite number of terms.

(1 + x)ⁿ = 1 + n x + [n(n - 1)/2!] x² +  [n(n - 1)(n - 2)/3!]  x³ +... 

Note: To apply this formula, the value of |x| should be less than 1.

Examples Using Binomial Expansion Formulas

Example 1: Find the expansion of (a + b)³.

Solution:

To find: (a + b)³

Using binomial expansion formula,

(x + y)ⁿ = ⁿC\(_0\) xⁿ y⁰ + ⁿC\(_1\) x^{n - 1} y¹ + ⁿC\(_2\) x^n-2 y² + ⁿC\(_3\) x^{n - 3} y³ + ... + ⁿC\(_{n-1}\) x y^{n - 1} + ⁿC\(_n\) x⁰yⁿ

(a + b)³ = ³C\(_0\) a³ + ³C\(_1\) a^{(3 - 1)} b + ³C\(_2\) a^{(3 - 2)} b² + ³C\(_3\) a^{(3 - 3)} b³ 

= ( 3! / [(3-0)!0!] )  a³ + ( 3! / [(3-1)!1!] ) a^{(3 - 1)} b + ( 3! / [(3-2)!2!] ) a^{(3 - 2)} b² + ( 3! / [(3-3)!3!] ) a^{(3 - 3)} b³

= (1) a³ + (3) a² b + (3) a¹ b² + (1) a⁰b³

= a³ + 3a² b + 3ab² + b³

Answer: (a + b)³ = a³ + 3a² b + 3ab² + b³.

Example 2: Find the expansion of (x + y)⁶.

Solution:

Using the binomial expansion formula,

(x + y)ⁿ = ⁿC\(_0\) xⁿ y⁰ + ⁿC\(_1\) x^{n - 1} y¹ + ⁿC\(_2\) x^n-2 y² + ⁿC\(_3\) x^{n - 3} y³ + ... + ⁿC\(_{n-1}\) x y^{n - 1} + ⁿC\(_n\) x⁰yⁿ

(x + y)⁶ = ⁶C\(_0\) x⁶ + ⁶C\(_1\) x⁵ y + ⁶C\(_2\) x⁴y² + ⁶C\(_3\) x³y³ + ⁶C\(_4\) x²y⁴ + ⁶C\(_5\) xy⁵ + ⁶C\(_6\) y⁶

= ( 6! / [(6-0)!0!] ) x⁶ + ( 6! / [(6-1)!1!] ) x⁵ y + ( 6! / [(6-2)!2!] ) x⁴y² + ( 6! / [(6-3)!3!] ) x³y³ + ( 6! / [(6-4)!4!] ) x²y⁴ + ( 6! / [(6-5)!5!] ) xy⁵ + ( 6! / [(6-6)!6!] ) y⁶ 

= x⁶ + 6x⁵ y + 15x⁴ y² + 20x³ y³ + 15x² y⁴ + 6x y⁵ + y⁶

Answer: (x + y)⁶ = x⁶ + 6x⁵ y + 15x⁴ y² + 20x³ y³ + 15x² y⁴ + 6x y⁵ + y⁶.

Example 3: Find the expansion of (3x + y)^1/2 upto the first three terms using the binomial expansion formula of rational exponents where \(\left|\dfrac y {3x}\right|\) < 1.

Solution:

(3x + y)^1/2 = 3x (1 + y/(3x))^1/2

Comparing (1 + y/(3x))^1/2 with (1 + x)ⁿ, we have x =y/(3x) and n = 1/2.

The expansion of (1 + y/(3x))^1/2 upto the first three terms using the binomial expansion formula is,

1 + n x + [n(n - 1)/2!] x² = 1 + (1/2) (y / (3x)) + [(1/2) ((1/2) - 1)/2!] (y / (3x))²

= 1 + y / (6x) - y² / (72x²)

Thus, the expansion of 3x (1 + y/(3x))^1/2 upto the first three terms is:

3x [ 1 + y / (6x) - y² / (72x²) ] = 3x + y / 2 - y² / (24x)

Answer: (3x + y)^1/2 = 3x + y / 2 - y² / (24x).