Altitude of a Triangle Formula

The perpendicular drawn from the vertex to the opposite side of the triangle is called the altitude of a triangle. The altitude of a triangle formula gives us the height of the triangle. The altitude of a triangle formula is interpreted and different formulas are given for different types of triangles. The altitude is used for the calculation of the area of a triangle.

What Is the Altitude of A Triangle Formula?

The altitude of a triangle formula can be expressed as follows. Here the altitude is represented by the alphabet h. Further, we can also see below the different altitudes of triangle formulas for different triangles.

General Formula for Altitude of a Triangle (h) = (2 × Area) ÷ base

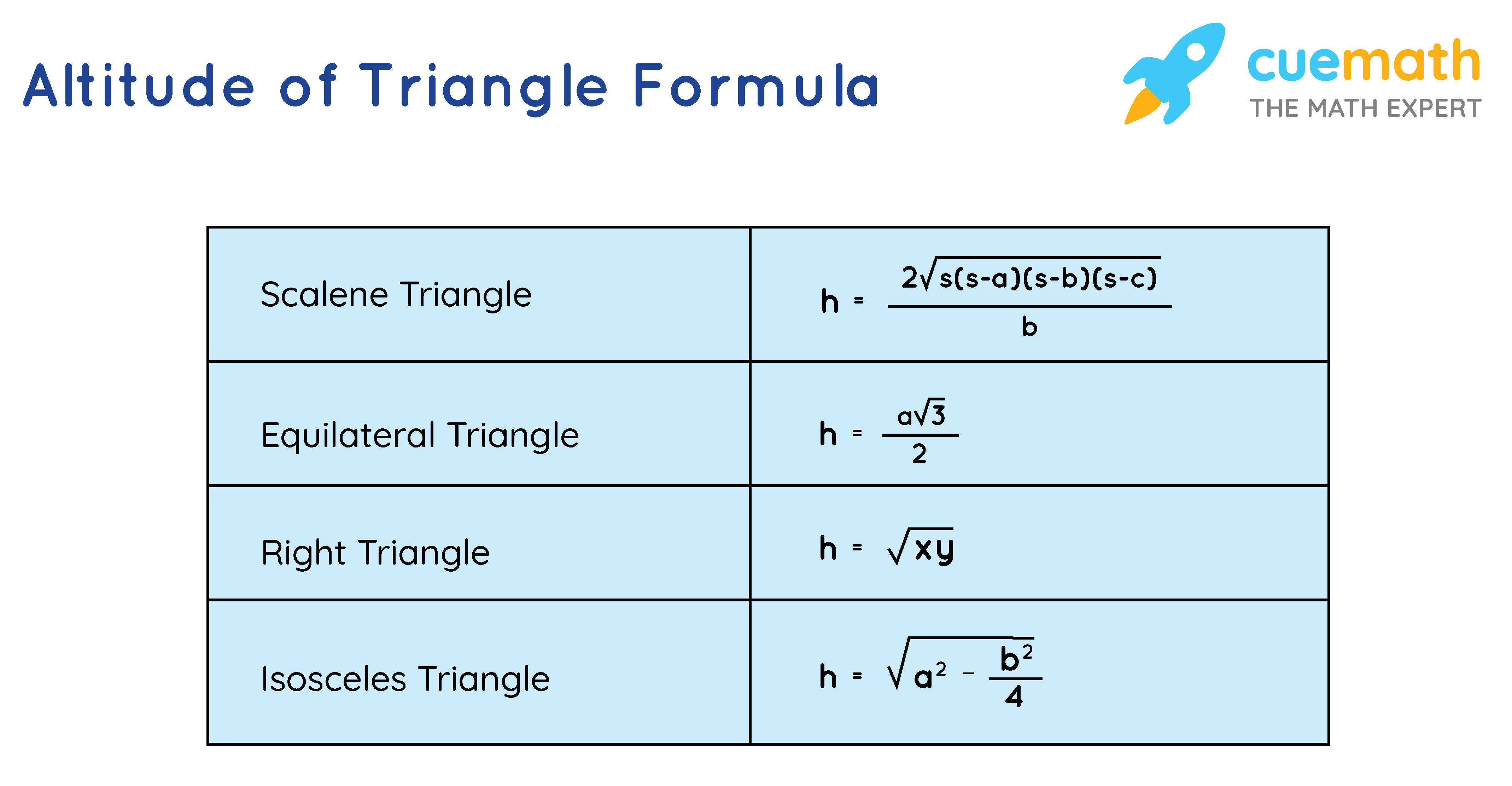
Altitude of A Triangle Formula for Different Triangles

We know that triangles are classified on the basis of sides and angles. Let us learn different altitude formulas on various different conditions for different types of triangles.

Altitude of a Triangle Formula for Scalene Triangle

Altitude of a scalene triangle is given as: \(h_a = \dfrac{2 \sqrt{s(s-a)(s-b)(s-c)}}{a}\), \(h_b = \dfrac{2 \sqrt{s(s-a)(s-b)(s-c)}}{b}\) and \(h_c = \dfrac{2 \sqrt{s(s-a)(s-b)(s-c)}}{c}\)Where a,b,c are the sides of the triangle, and s is the semi perimeter

Altitude of a Triangle Formula for an Equilateral Triangle

Altitude of an equilateral triangle is given as: \(h= \dfrac{a\sqrt{3}}{2}\)

Where 'a' is a side of a triangle

Altitude of a Triangle Formula for a Right Triangle

Altitude of a right triangle is given as: \(h= \sqrt{xy}\)

where x and y are the length of segments of hypotenuse divided by altitude. The altitude is the geometric mean of the line segments x and y.

Altitude of a Triangle Formula for an Isosceles Triangle

Altitude of an isosceles triangle is given as: \(h= \sqrt{a^2- \frac{b^2}{4}}\) where a and b are the side of a triangle.

Examples on Altitude of A Triangle Formula

Let us take a look at a few examples to understand the altitude of a triangle formula

Example 1: The area of a right triangular board is 720 sq. units. Find the length of the altitude if the length of the base is 90 units.

Solution:   To find: The length of the altitude.

The area of a right triangular board = 720 sq. units(given)
The length of the base = 90 units
Using altitude of a triangle formula,
Altitude of a triangle(h) = (2× Area) ÷ base
=(2 × 720)/90
= 16 units

Answer:  The length of the altitude of a triangular board is 16 units.

Example 2: Calculate the length of the altitude of a triangle drawn from vertex A, whose sides a,b.c are 8 feet, 7 feet, 9 feet respectively. 

Solution:  To find: Altitude of a triangle.
Semi-perimeter= s = (9 + 7 + 8)/2 = 24/2 = 12 feet 
Using altitude of a triangle formula,
Altitude of the triangle (h) = \(\dfrac{2 \sqrt{s(s-a)(s-b)(s-c)}}{a}\)
Altitude \(h_a= \dfrac{2 \sqrt{12(12-8)(12-7)(12-9)}}{8}\)
= \(\dfrac{2 \sqrt{12\ \times 4\ \times 5\ \times 3}}{8}\)
= (12√ 5)/4
= 3√ 5 feet

Answer: The length of the altitude of a triangle drawn from vertex A is 3√ 5 feet.

Example 3: Find the altitude of an equilateral triangle whose length of a side is 8 units.

Solution:   To find: The length of the altitude of an equilateral triangle.
Using altitude of a triangle formula for an equilateral triangle h= (a√ 3)/2 where 'a' is a side of a triangle
h= (8√ 3)/2 feet = 6.928 units.

Answer:  The length of the altitude of an equilateral triangle is 6.928 units.