Zero Function
There are different kinds of functions that we study in mathematics. A function is a relation that maps the elements in the domain to the elements in the co-domain such that each element in the domain is mapped to only one element in the co-domain. Zero function is a function whose domain consists of all real numbers and the range consists of a single element, that is, 0. Zero function is also a constant function as its value never changes with changes in inputs. In this article, we will explore the properties of a zero function and its nature.
| 1. | What is a Zero Function? |
| 2. | Zero Function Graph |
| 3. | Characteristics of Zero Function |
| 4. | Is Zero Function Even or Odd? |
| 5. | FAQs on Zero Function |
What is a Zero Function?
A zero function is a constant function for which the output value is always zero irrespective of the inputs. The input of a zero function can take any value from the real numbers whereas the output of the zero function is fixed, that is, 0. Since the image of every element in the domain is 0, therefore zero function is not a one-to-one function.
Zero Function Meaning
A function f: R → R defined as f(x) = 0, for all values of x in R, is called a zero function. The range of a zero function is a singleton set, that is, {0}. Just like any other constant function graph parallel to the x-axis, the graph of the zero function is the x-axis itself as the value of the y-coordinate is 0 throughout the graph. It is a many-to-one function as all elements in the domain have the same image, that is, 0.
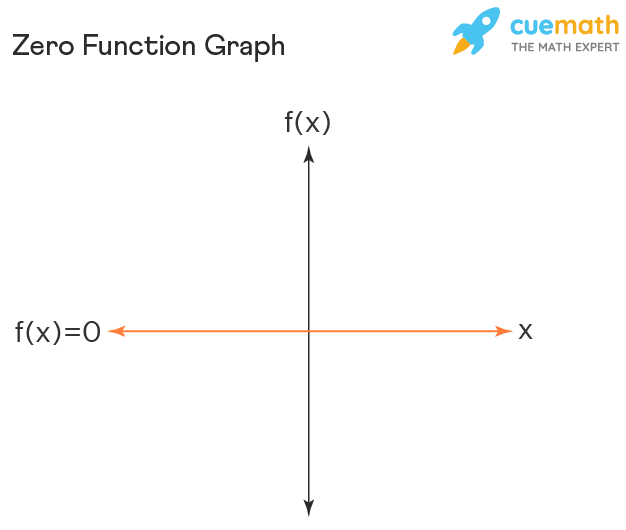
Zero Function Graph
The graph of a zero function f(x) = 0 is similar to other constant functions graphs which are parallel to the x-axis. Any function can be considered as a constant function if it is of the form y = k, where k is a constant and k is any real number. It is also written as f(x) = k. Since the range is zero for the zero function and the value of the y-coordinate is always zero, therefore the graph of the zero function is the X-axis itself. In other words, we can say that the zero function graph is the horizontal axis.
Characteristics of Zero Function
Functions have various characteristics such as slope, domain, range, differentiability, limit, and continuity. Let us now explore the various characteristics of a zero function. Being a type of a constant function, the zero function has properties similar to the constant functions.
- Slope of Zero Function: Zero function can also be written as y = 0x + 0. Comparing this form with the slope-intercept form of a line y = mx + b, where m is the slope of the line and b is the y-intercept, we get the slope of the zero function is 0.
- Domain and Range of Zero Function: A zero function is a linear function whose range contains only one element irrespective of the number of elements in the domain. Since the zero function is defined for all values of x, therefore the domain is all real numbers R, and the range of the zero function is {0}.
- Derivative of Zero Function: The differentiation of any constant function zero. The derivative is considered to be the slope of the function at any given point, and we already know that the slope of the zero function is always 0. Hence, the derivative of the zero function is 0.
- Limit of Zero Function: According to the properties of limits, the limit of a constant function is equal to the same constant. Hence, the limit of the zero function is equal to 0.
- Continuity of Zero Function: The constant functions are continuous as they represent horizontal lines that extend continuously on both sides without any break. As zero function is a constant function, therefore the zero function is a continuous function without any break throughout the domain.
Is Zero Function Even or Odd?
A function f is said to be even if f(-x) = f(x), for all values of x in the domain of f and the function is said to odd if f(-x) = -f(x), for all values of x in the domain of f. Zero function is the only function that satisfies both these conditions together for all values of x in the domain of f. Hence, the zero function is both even and odd. If f(x) = 0 is the zero function, then f(-x) = f(x) = -f(x) = 0 as the output always remains the same.
Important Notes on Zero Function
- The output (range) of a zero function is always 0.
- Zero function is both even and odd.
- The graph of zero function is the X-axis and it is a continuous function.
Related Topics to Zero Function
Examples on Zero Function
-
Example 1: Determine whether the function f(x) = 6 is a zero function.
Solution: f(x) = 6 is a constant function whose range is always 6 and 6 is not equal to 0. For zero function, the range is equal to {0} and is of the form f(x) = 0 for all x. Therefore f(x) = 6 is not a zero function.
Answer: No, f(x) = 6 is not a zero function.
-
Example 2: What is the limit of the zero function f(x) = 0 as x tends to infinity?
Solution: The limit of a constant function is always equal to the value of the constant (output) at all values of x. Therefore, the limit of the zero function f(x) = 0 is 0 when x tends to infinity.
Answer: The limit of the zero function 0 as x tends to infinity.

FAQs on Zero Function
What is Zero Function in Maths?
A function f: R → R defined as f(x) = 0, for all values of x in R, is called a zero function. The range of a zero function is a singleton set, that is, {0}. A zero function is a constant function for which the output value is always zero irrespective of the inputs.
What is Non-Zero Function?
A function whose output is not equal to zero, for all values of inputs, is called a non-zero function. A non-zero function can have 0 as the output for some values in the domain but if it is equal to zero for all the elements in the domain, then it is a zero function.
Is Zero Function Even or Odd?
A zero function is the only function that is both even and odd as it satisfies both the conditions f(-x) = f(x) and f(-x) = -f(x) for all values of x in the domain of f.
What is the Integral of the Zero Function?
The integral of a zero function f(x) = 0 is an arbitrary constant C that can take any value of the real numbers. As the derivative of any constant is zero, hence the integral of the zero function is an arbitrary constant.
How do you Find the Zeros of a Function?
The zeros of a function can be calculated using different methods such as the quadratic formula and factorizing the polynomial.
visual curriculum