Types of Polynomials

In algebra, a polynomial is an expression that is made up of variables and constants in which the exponents of the variables are only positive integers and not fractions. The polynomial terms are separated mostly by either addition or subtraction operators. Polynomials find their applications in writing polynomial equations and defining polynomial functions. An example of a polynomial expression is x²+2x-3. This polynomial has a degree of 2 since the term with the highest power of 'x' is 'x²'. This polynomial has 3 terms. Polynomials can be categorized with respect to their total number of terms and their degree.

Let us see what are different types of polynomials along with examples.

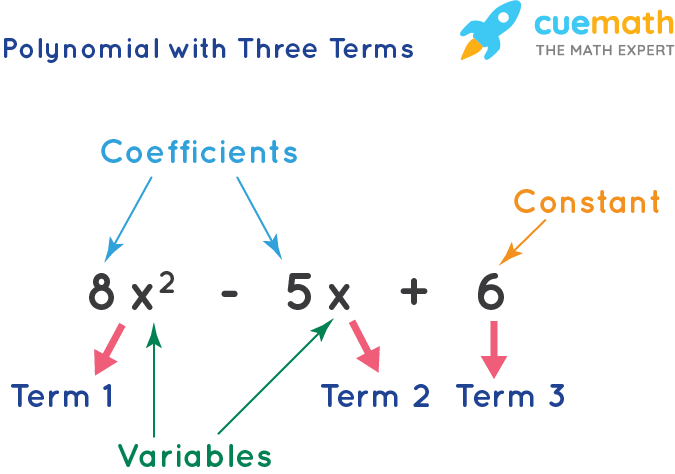
Polynomials are algebraic expressions in which the variables have only non-negative integer powers. For example,: 5x² - x + 1 is a polynomial. The algebraic expression 3x³ + 4x + 5/x + 6x^3/2 is not a polynomial, since one of the powers of 'x' is a fraction and the other is negative. Polynomials are expressions with one or more terms having a non-zero coefficient. The terms comprise variables, exponents, and constants. The first term of the polynomial in standard form is called the "leading term". A standard polynomial is one where the highest degree is the first term, and the subsequent terms are arranged in descending order of the powers or the exponents of the variables followed by constant values. The number multiplied by a variable is called the "coefficient". The number without any variable is called a "constant".

The polynomials can be classified in two ways: based on degree and based on the number of terms.

• The types of polynomials based on degree are zero polynomial, linear, quadratic, and cubic polynomials.
• The types of polynomials based on the number of terms are monomials, binomials, trinomials, etc.

Let us see each of these types in the upcoming sections in detail.

The power of the leading term or the highest power of the variable is called the degree of the polynomial. This is obtained by arranging the polynomial terms in the descending order of their powers. Based on the degree of the polynomial, they can be classified into 4 major types. They are,

• Zero or constant polynomial
• Linear polynomial
• Quadratic polynomial
• Cubic polynomial

Look at the table given below to understand the meaning of the types of polynomials with examples.

There are different types of polynomials with respect to their number of terms. There are polynomials with one term, two terms, three terms, and even more. Based on the number of terms, polynomials are classified as:

• Monomials: A monomial is a polynomial expression that contains only one term. For example 4t, 21x, 2y, 9pq. Furthermore, 2x + 5x + 10x is a monomial because these are like terms added together that result in 17x.
• Binomials: A binomial is a polynomial with two, unlike terms. For example, 3x + 4x² is a binomial as it contains two unlike terms, that is, 3x and 4x². and 10pq + 13p².
• Trinomials: A trinomial is a polynomial with three, unlike terms. For example, 3x + 5x² – 6x³ and 12pq + 4x² – 10.

We can also have more than 3 terms in a polynomial expression. Polynomials that have 4 unlike terms are called four-term polynomials. Similarly, polynomials with 5 terms are called five-term polynomials, and so on.

Important Notes on Types of Polynomials:

• In a polynomial, two terms are separated by the addition or subtraction sign. Multiplication and division operators are not used to create more terms in a polynomial. For example, 3xy is considered as 3 × x × y, is a monomial, whereas 3x+y is a binomial.
• Based on the terms in a polynomial, it can be classified into the following 3 types. They are monomial, binomial, trinomial.
• Based on the degree of a polynomial, it can be classified into 4 types. They are zero polynomial, linear polynomial, quadratic polynomial, cubic polynomial.
• Polynomials should have a whole number as the degree. Expressions with negative exponents are not polynomials. For example, x^-2 is not a polynomial.
• Polynomials do not have variables in their denominator. For example, 2/(x+2) is not a polynomial.

☛ Related Topics:

Check out some interesting articles related to types of polynomials.

• Polynomial Calculator
• Polynomial Functions
• Polynomial Identity
• n^th Degree Polynomial

What are Different Types of Polynomials?

A polynomial is an expression that is made up of variables and constants. Polynomials are categorized based on their degree and the number of terms. Based on the number of terms in a polynomial, there are 3 types of polynomials. They are monomial, binomial and trinomial. Based on the degree of a polynomial, they can be categorized as zero polynomials, linear polynomials, quadratic polynomials, and cubic polynomials.

What are the Types of Polynomials Based on the Number of Terms?

Polynomials are categorized according to the number of terms as follows. They are,

• Monomials: It is a polynomial with one term. Examples: 3x, 4y, -7t, etc,.
• Binomials: It is a polynomial with two unlike terms. Examples: 3x - 5y, 7y + 10 z, etc.
• Trinomials: It is a polynomial with three unline terms. Examples: x + y + 3z, x² + 5y + 2, etc.

In a polynomial, there should not be any negative number as the exponent of the variable. The variable should not be in the denominator of the polynomial that is in fractional form.

pqr is What Type of Polynomial?

pqr is just one term because there is no addition/subtraction being happened here. Thus, pqr is a monomial.

What are the Types of Polynomials Based on Degree?

Polynomials can be classified on the basis of their degree as follows. They are,

• Zero polynomial: The degree of zero polynomials is 0. For example, 2 or 2y⁰.
• Linear polynomial: The degree of linear polynomials is 1. For example, p + q - 2.
• Quadratic polynomial: The degree of quadratic polynomials is 2. For example, x² + 3x + 3.
• Cubic polynomial: The degree of cubic polynomials is 3. For example, 5x³ - 1.

What Type of Polynomial is a Polynomial with 5 Terms?

Polynomials with 5 terms are known as five-term polynomials. For example, 3x³-xy+y²+6x²-8.

What is a Polynomial with 4 Terms Called?

Polynomials with 4 terms are known as quadrinomials. For example, x³+3x-2y+5.