Standard Form of Linear Equations
The standard form of linear equations is a method of writing linear equations. A linear equation can be written in different forms like the standard form, the slope-intercept form, and the point-slope form. The standard form of linear equations is also known as the general form and is represented as Ax + By = C. Let us learn more about the standard form of linear equations in one variable and two variables in this article.
| 1. | What is the Standard Form of Linear Equations? |
| 2. | Standard Form of Linear Equations in Two Variables |
| 3. | FAQs on Standard Form of Linear Equations |
What is the Standard Form of Linear Equations?
An equation in which the highest power of the variable is 1 is called a linear equation or a one-degree equation. For example, 4x + y = 6 is a linear equation because the highest power of both the variables x and y is 1. The standard form of linear equation is represented as: Ax + By = C; where A, B, and C are integers and the letters x and y are the variables.
Standard Form of Linear Equations in One Variable
Linear equation in one variable means that the equation has only one variable in it. It means that this linear equation has one solution to it. The standard form or the general form of linear equations in one variable is written as,
Ax + B = 0
- Where A and B are integers, and
- x is the single variable.
- For example, 3x + 6 = 12 is the standard form of a linear equation with a single variable and when we solve for the value of x we get only one solution which is 4.
Standard Form of Linear Equations in Two Variables
When a linear equation has two variables in it, it has two solutions. The standard form of linear equations (general form of linear equations) in two variables is expressed as,
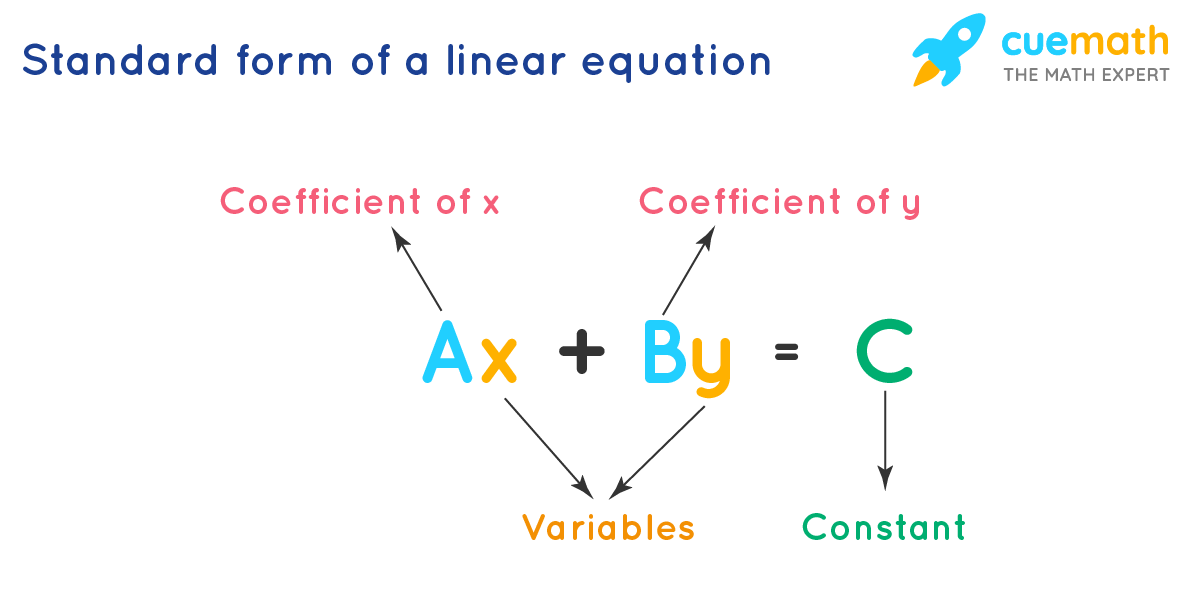
Ax + By = C
- Where A, B and C are integers, and
- x and y are the variables
- For example, 3x + 4y = 8 is the standard form of a linear equation in two variables.
How to Write the Standard Form of Linear Equations in Two Variables?
When we need to rewrite a given linear equation in its standard form, we can easily convert it in the general form, that is, Ax + By = C, where A, B, and C should be integers and the order of the terms should be as given.
Example: Rewrite the linear equation in standard form, 2y = -5x + 7
Solution: In order to rewrite the given equation in standard form, we will transpose the term -5x on the left-hand side. This means it will become 2y + 5x = 7. Now, we can arrange the terms on the left-hand side as per the order given in the standard form. This will make it 5x + 2y = 7. This equation is in its standard form.
☛ Related Articles
FAQs on Standard Form of Linear Equations
What is the Standard Form of Linear Equations?
The standard form of linear equations is one of the ways in which a linear equation is written. It is expressed as Ax + By = C, where A, B, and C are integers, and x and y are variables. This is the general form of a linear equation that has two variables in it. For linear equations with one variable, the standard form is expressed as, Ax + B = 0. Here, A and B are integers and 'x' is the only variable.
What is the General Form of Linear Equations in Two Variables?
The standard form of linear equations is also known as the general form. When we talk about the general form of a linear equation in two variables, it is expressed as Ax + By = C, where A, B and C are integers, and x and y are the two variables. There are many examples in which we need to rewrite a given equation in the standard form. So, following this standard form, we can easily convert and rewrite any linear equation in its standard form.
What is the Standard Form of Linear Equations in One Variable?
The standard form of linear equations in one variable is expressed as Ax + B = 0, where x is the single variable and A and B are integers. For example, 6x + 7 = 18 is a linear equation in one variable which is written in its standard form.
How is the Standard Form of Linear Equation Different from the Slope-Intercept Form?
Linear equations can be written in different forms like the standard form, the slope-intercept form, and the point-slope form. The slope-intercept form of a linear equation is y = mx + b. Here, 'm' is the slope, and 'b' is the y-intercept. For example, y = 4x - 3 is an equation written in the slope-intercept form. This form is usually easier to graph. The standard form of a linear equation is Ax + By = C, where A, B, and C are integers. For example, 5x + 6y = 15.
How to Write the Standard Form of Linear Equations?
Sometimes we need to rewrite a given linear equation in its standard form. We can easily convert it in the general form, that is, Ax + By = C, where A, B and C should be integers. For example, let us write the following equation in the standard form: -6y = 4x + 10. Now, in order to rewrite this equation in its standard form, we will transpose -6y to the right-hand side of the equation and move 10 to the left-hand side of the equation. This will make it, -10 = 4x + 6y. Then, we will flip the sides and write it as 4x + 6y = -10.