Square Root of 89

A number’s square root is the value which, when multiplied by itself, gives the original number. Let's explore the simulation below to find the square root of any number, such as the square root of 89. Once you grasp the basic concept of square roots, any lesson can be easily solved.
In this lesson, you will learn the square root of 89 and how to find the square root of  89.

• Square Root of 89: √89=9.43 (up to 2 decimal place)
• Square of 89: 89² =7921

Table of Contents

• What Is the Square Root of 89?
• Is Square Root of 89 Rational or Irrational?
• How to Find the Square Root of 89?
• FAQs on Square Root of 89
• Tips and Tricks
• Solved Examples on Square Root of 89 
• Interactive Questions on Square Root of 89 
• Challenging Questions

The square root of a number n can be written as √n. It means that there is a number aa such that a×a=n. This can also be written as, a²=n ⇒ a=√n. So, a is equal to the square root of n.
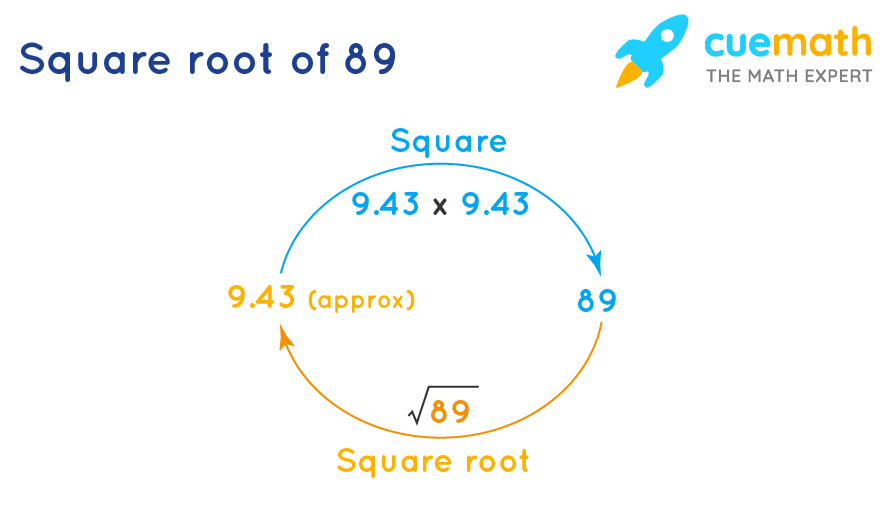
Now, if n=89, then a=√89 is the square root of 89. In the radical form, the square root of 89 can be represented by √89. The square root of 89 = 9.43  in the decimal form up to 2 decimal places. 

A rational number is a number that can be expressed in the form of p/q. A number that is not a rational number is called an irrational number. Due to the nature of its non-repeating and non-terminating decimal expansion, the square root of 89 cannot be written in the form of p/q, hence, it is an irrational number.
The value of the square root of 89 in decimal form is 9.433981.. so on, The square root of a number can have two values, a positive and a negative value. So, √89=+9.433981. Hence, The square root of 89 is an irrational number with never-ending digits.

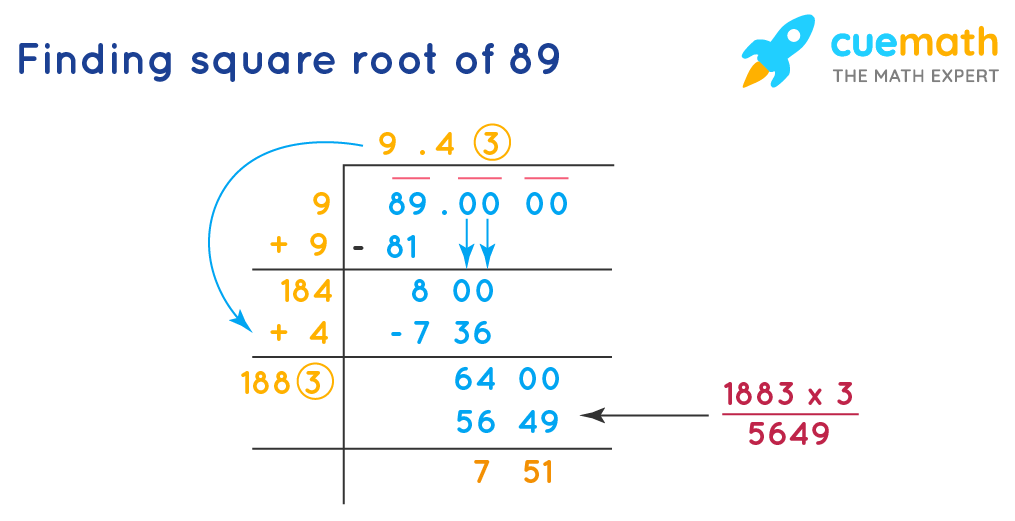
The long division method helps us to find a more accurate value of the square root of a number. Let's see the steps to find the square root of 89 by the long division method.

• Step 1: Divide the number 89 by 9, (because 9² =81 is a perfect square number just less than 89).
• Step 2: The divisor and the quotient need to be the same numbers; in this case, it is 9. Multiply the quotient and the divisor and subtract the result from 89.
• Step 3: For the next divisor, add the previous divisor with the quotient. (9 + 9 = 18); place it as the new divisor with a blank on its right.
• Step 4: Put a decimal after quotient '9' and bring down two zeros to place it after 8 so that it becomes 800. (184 × 4 = 736). Subtract 736 from 800.(800 − 736 = 64)
• Step 5: Bring down another pair of zeros and place it after 64, so that it becomes 6400. Take the new quotient 4 and add it to 184. (184 + 4 = 188)
• Step 6: Repeating the above steps, we need to fill the blank with a number such that when the new divisor is multiplied with the new quotient, the product is less than or equal to the dividend 6400. (1883 × 3 = 5649)
• Step 7: Write the same number after 4 in the quotient. Subtract 5649 from 6400. (6400 − 5649  = 751)
• Step 8: Repeat the process until we get the remainder equal to zero. The square root of 89 up to two places is obtained as 9.43 by the long division method.

Explore Square roots using illustrations and interactive examples

• Square Root of 65
• Square Root of 17
• Square Root of 5
• Square Root of 11
• Square Root of 13

• Use the hit and trial method while calculating the square root of any number.
• The square root of a number can have two values.
• The square root of any number can be assumed between the square root of two nearest perfect squares of that number. For example, the square root of 89 lies between the square root of 81 and 100. So value would be greater than 9 and less than 10.

• Find the square root of 899 by the approximation method.
• Find the largest 5-digit number which is a perfect square.

What is the square root of 89 simplified?

Since 89 is prime, the square root of 89 cannot be simplified.

What is a square root of 89?

The square root of 89 = 9.43  in the decimal form up to 2 decimal places.

Is the square root of 89 a rational number?

No, the square root of 89 is an irrational number.

Is 89 a perfect square?

No, 89 a not a perfect square.

What is the square root of 89 to the nearest tenth?

The square root of 89 to the nearest tenth is 9.4.