Square root of 80
The square root of 80 is a number which when multiplied by itself, results in the number 80. Without saying more, let us see how to calculate the value of the square root of 80 and see some problems to help us understand this topic.
Square Root of 80: √80 = 8.9442719...
Square of 80: 80² = 6400
| 1. | What is the Square Root of 80? |
| 2. | Is Square Root of 80 Rational or Irrational? |
| 3. | How to Find the Square Root of 80? |
| 4. | FAQs on Square Root of 80 |
What is the square root of 80?
The square root of a number n is written as √n. This number when squared or multiplied by itself results in the original number n. The square root of 80 can be written in multiple ways
- Radical form: √80 = 4√5
- Decimal form: 8.944
- Exponent form: (80)¹⁺²
Is Square Root of 80 Rational or Irrational?
- 80 is a number that is not a perfect square, meaning it does not have a natural number as its square root.
- Also, its square root cannot be expressed as a fraction of the form p/q which confirms to us that the square root of 80 is an irrational number.
How to Find the Square Root of 80?
There are multiple ways to find the square root of 80-
- Long Division Method
- Estimation and Approximation
- Prime Factorization
One can find out more about these methods by clicking here. We will be looking at the Long Division Method and Prime Factorization Method.
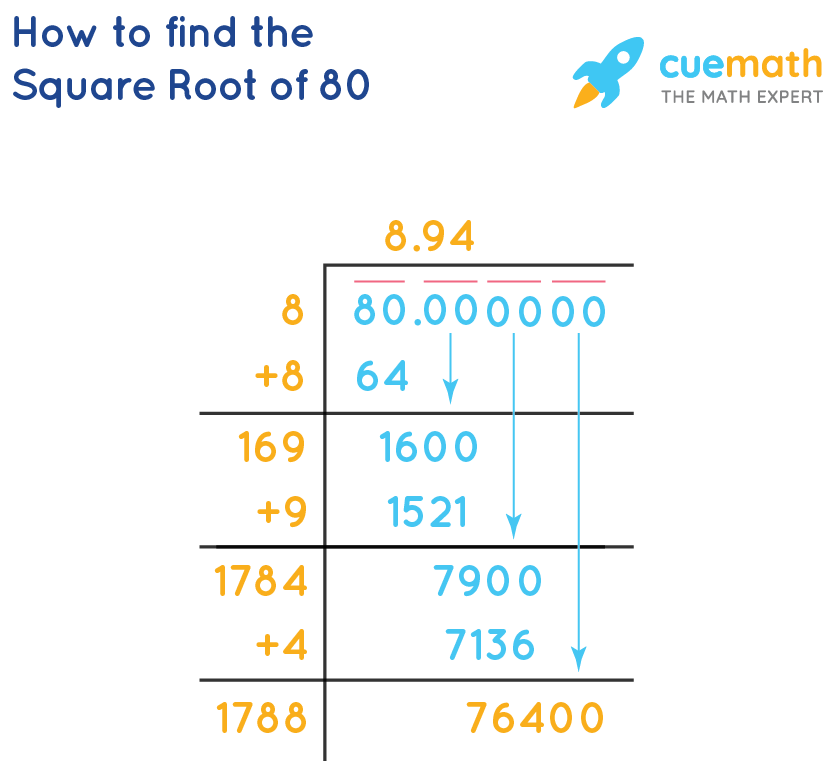
Long Division Method
The square root of 80 by long division method consists of the following steps:
- Step 1: Pair 80 and place a bar over the digits. We also pair the 0s in decimals in pairs of 2 from left to right.
- Step 2: Find a number that, when multiplied to itself, gives the product less than or equal to 80. The number 8 fits here as 8 squared gives 64. Dividing 80 by 8, we get the quotient as 8 and the remainder as 16.
- Step 3: Drag a pair of 0’s down and fill it next to 16 to make the dividend 1600.
- Step 4: Double the divisor 8, and enter 16 below with a blank digit on its right. Guess the largest possible digit(X) to fill in the blank and the quotient for which the product of 16X and X results in a value less than or equal to 1600. Divide and write the remainder.
- Step 5: Repeat this process to get the decimal places you want.
Prime Factorization Method
The first step in this method is to find the prime factors of 80.
80 = 2 × 2 × 2 × 2 × 5
To find the root from here, we can simplify this to
√80 = √(2 × 2 × 2 × 2 × 5)
√80 = 4√5
We can either leave it at this or find the root of 5 which is 2.23 and simplify further
Therefore, Square Root of 80 = 4√5 ≅ 8.92
Explore Square roots using illustrations and interactive examples
Important Notes
- There exists a positive and negative root of 80, 8.944 and -8.944
- The square root of 80 is an irrational number that is non-terminating
Solved Examples
-
Example 1: Lucas wants to calculate the side length of his land he recently bought. The square footage of the land is 80.
Solution:
To find the side of the land, we will have to find the square root of 80. The square root is √80 = 4√5. Hence, the side length of the garden is 4√5 feet.
-
Example 2: Joel had a doubt. He knew that 8.944 is a square root of 80. He wanted to know if -8.944 is also a square root of 80? Can you clarify his doubt?
Solution:
Let us take an example of a perfect square number and extend the same logic to clarify her doubt. We know that 6 is a square root of 36 because when 6 is multiplied by itself it gives 36.
But what about -6? Let us multiply and check.
-6 × -6 = 36, because (- × - = +). Therefore -6 is also a square root of 36
Going by the same logic, -8.944 is also the square root of 80.

FAQs on Square Root of 80
What is the prime factorization of 80?
Prime factorization of 80: 24 × 5
Is 80 a perfect square root?
80 is not a perfect square. 80 is a natural number, but since there is no other natural number that can be squared to result in the number 80, it is NOT a perfect square root.
What is the decimal value of the square root of 80?
The decimal value of the square root of 80 is 8.944.
Is the square root of 80 a rational number?
No, the square root of 80 is not a rational number since the square root of 80 is non-terminating and cannot be represented in the form of p/q.
Can we find the square root of 80 by the repeated subtraction method?
No, we can’t find the square root of 80 by repeated subtraction method as it can be used only for perfect squares. 80 is not a perfect square.
visual curriculum