Square Root of 7
The square root of 7 is expressed as √7 in the radical form and as (7)½ or (7)0.5 in the exponent form. The square root of 7 rounded up to 8 decimal places is 2.64575131. It is the positive solution of the equation x2 = 7.
- Square Root of 7: 2.6457513110645907
- Square Root of 7 in exponential form: (7)½ or (7)0.5
- Square Root of 7 in radical form: √7
Let's explore more about finding the square root of 7 in this mini-lesson.
What Is the Square Root of 7?
- The square root of a number is the number that when multiplied to itself gives the original number as the product.
- √7 = 2.645 x 2.645 or -2.645 x -2.645
Is the Square Root of 7 Rational or Irrational?
- A rational number is defined as a number that can be expressed in the form of a quotient or division of two integers, i.e. p/q, where q is not equal to 0.
- √7 = 2.645751311064591. Due to its never-ending nature after the decimal point, √7 is irrational.
How to Find the Square Root of 7?
The square root of 7 can be calculated using the average method or the long division method. √7 cannot be simplified any further as it is prime. The radical form of the square root of 7 is √7.
Square Root of 7 by Average Method
- The square root of 7 will lie between the square root of the two perfect squares closer to 7.
- We will first identify the square root of 4 and the square root of 9. √4 < √7 < √9.
- Thus, we determine that the square root of 7 lies between 2 and 3. 2 < √7 < 3
- Using the average method, find 7 ÷ 3 or 7 ÷ 2.
- 7 ÷ 3 = 2.33
- Find the average of this quotient obtained and 3. Average = (2.33 + 3) ÷ 2 = 5.33 ÷ 2 = 2.66
- Thus, √7 = 2.66 by the average method.
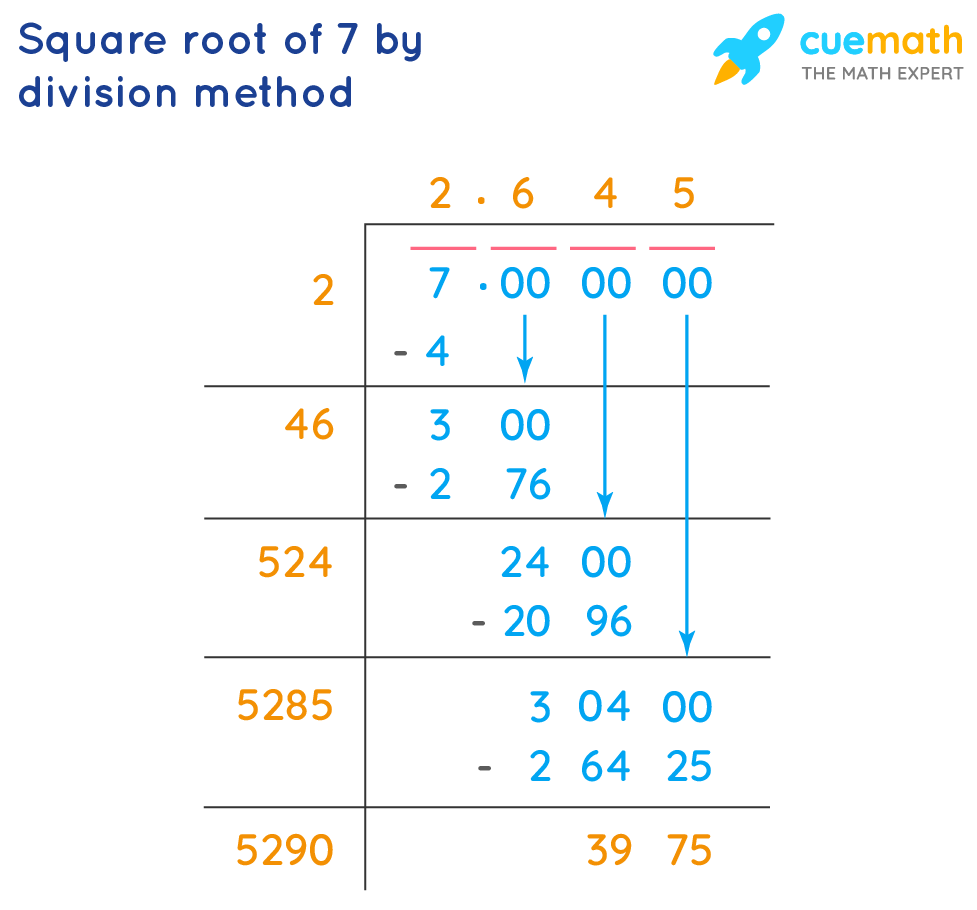
Square Root of 7 by Long Division Method
- Write 7 as 7.000000. Consider the number in pairs from the right. So 7 stands alone.
- Now divide 7 with a number such that number × number gives 7 or a number lesser than that. We determine 2 × 2 = 4
- Complete the division process. Obtain 2 as the quotient and 3 as the remainder. Bring down the first pair of zeros.
- Double the quotient obtained. Now 2 × 2 forms the new divisor in the tens place.
- Find a number which in the units place along with 40, fetches the product 300 or a number lesser than that.
- We find that 6 × 46 gives 276. Complete the division and get the remainder as 24.
- Now our quotient is 2.6. Double this and get 520 as our new divisor.
- Bring down the next pair of zeros. Find the number that with 520 gives 2400 or a number lesser than that.
- We conclude 4 × 524 = 2096. Complete the division.
- Repeat the same division process until we get the quotient approximated to 3 digits.
- Thus, we have evaluated √7 = 2.645.
Explore square roots using illustrations and interactive examples.
Important Notes
- The square root of 7 is expressed as √7 in the radical form and as 7½ in the exponential form.
- The square root of a number is both negative and positive for the same numerical value, i.e., the square root of 7 is +2.645 or -2.645.
Tips and Tricks
- The square root of 7 lies between the perfect squares closer to 7. Thus, √7 lies between 2 and 3.
- Use the average method to determine the approximate value of 7 and the division method to determine the accurate value of √7.
Square Root of 7 Solved Examples
-
Example 1: The area of the pizza that Mike bought is 22 square units. What will be the radius of the pizza?
Solution:
Area of the pizza = π r2 square units
π r2 = 22
r2 = 22 × 7 / 22
r2 = 7. This implies r = √7
Thus, the radius of the pizza is 2.645 units.
-
Example 2 : If a2 = 0.07, find a.
Solution:
Given a2 = 0.07
a2 = (7/100)
a = √(7/100)
= √7/√100
= √7/10
= 2.645/10
Thus, a = 0.2645
-
Example 3: In a right-angled triangle, the two legs measure √3 and 2 respectively. What is the measure of the hypotenuse?
Solution:
According to the Pythagorean theorem,
Hypotenuse2 = leg12+ leg22
Hypotenuse2 = ( √3)2 + 22
Taking square root, we get √Hypotenuse2 = √(( √3)2 + 22 )
Hypotenuse = √(3+4) = √7 = 2.645
Thus, the hypotenuse measures 2.645.

FAQs on the Square Root of 7
What is the Value of the Square Root of 7?
The square root of 7 is 2.64575.
Why is the Square Root of 7 an Irrational Number?
The number 7 is prime. This implies that the number 7 is without its pair and is not in the power of 2. Therefore, the square root of 7 is irrational.
Is the number 7 a Perfect Square?
The number 7 is prime. This implies that the square root of 7 cannot be expressed as a product of two equal integers. Therefore, the number 7 is not a perfect square.
What is the Square Root of 7 in Simplest Radical Form?
The number 7 is a prime number. This implies that the number 7 is without its pair and is not in the power of 2. Therefore, the radical form of square root of 7 cannot be simplified further.
What is the Value of 20 square root 7?
The square root of 7 is 2.646. Therefore, 20 √7 = 20 × 2.646 = 52.915.
What is the Square Root of -7?
The square root of -7 is an imaginary number. It can be written as √-7 = √-1 × √7 = i √7 = 2.645i
where i = √-1 and it is called the imaginary unit.
visual curriculum
