Square Root of 63

We all know that the length of each side of a square is the square root of its area. Can you think of a number that is a square root of 63? In this mini-lesson, we will explore the world of the square root of 63 various numbers. We will walk through the definition of the square root of 63, find out whether the square root of 63 is rational or irrational, and see how to find the square root of 63 by the long division method.

• Square Root of √63: 7.937 (up to 3 decimal place)
• Square of 63: 63² = 3969

Square root of 63 in the radical form is expressed as √63 and in exponent form it is expressed as 63^1/2. The square root of 63 rounded to 3 decimal places is ±7.937. We can also express the square root of 63 in its lowest radical form as 3√737. Numbers that have a radical symbol in its lowest form are called surds.

A number that cannot be expressed as a ratio of two integers is an irrational number. The decimal form of the irrational number will be non-terminating (i.e it never ends) and non-recurring (i.e the decimal part of the number never repeats a pattern). Now let us look at the square root of 63, √63=7.937. Do you think the decimal part stops after 7.937? No, it is never-ending and you cannot see a pattern in the decimal part.
So √63 is an irrational number.

• Square root of 63 in the radical form is expressed as √63
• In exponent form square root of 63 is expressed as 63^1/2
• The value of √63 are ±7.94 rounded to 2 decimal places

There are two different methods to find the square root of 63

• By prime factorization method
• Long division method.

Let us first find the square root of 63 by the prime factorization method. To find the square root of 63, let us first express 63 as a product of its prime factors.
Prime factorization of 63 = 3 × 7, therefore √63 = √7 × 3² = 3√7 = 7.937
Now let us try to find the square root of 63 by the long division method.

Square Root of 63 by Long Division Method

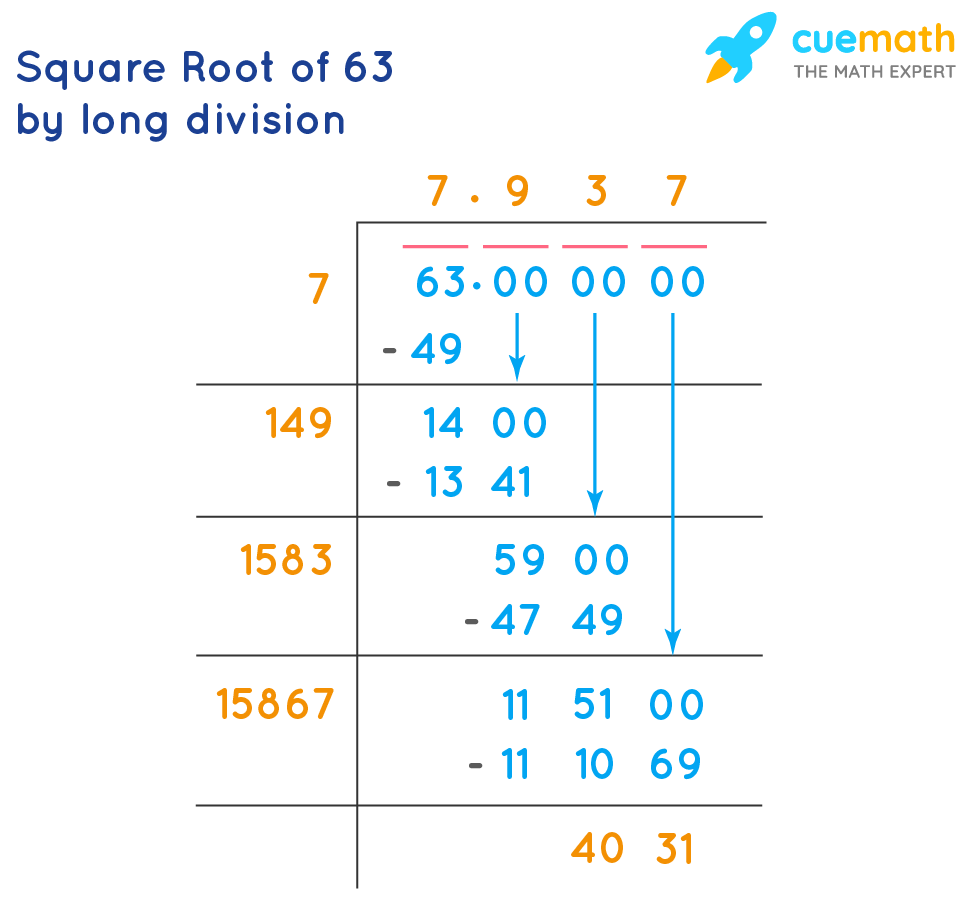
Let us follow the steps to find the square root of 63 by long division.

• Step 1: Make a pair of digit (by placing a bar over it ) from the one's place  since our number is 63.Let us represent it as inside the division symbol.
• Step 2: Find a number such that when you multiply it with itself, the product is less than or equal to 63. We know that  7 ×× 7 is 49 and is less than 63. Now let us divide 63 by 7
• Step 3: Let us place a decimal point and zeros' pairs and continue our division. Now, multiply the quotient by 2 and the product becomes the starting digit of our next divisor.
• Step 4: Choose a number in the unit's place for the new divisor such that  its product with a number is less than or equal to 1400. We know that 4 is in the ten's place and our product has to be 1400 and the closest multiplication is 149 × 9 = 1341
• Step 5:  Bring down the next pair of zeros and multiply the quotient 79 (ignore the decimal) by 2, which is 158 and the starting digit of the new divisor.
• Step 6: Choose a number in the unit's place for the new divisor such that its product with a number is less than or equal to 5900. We see that 1583, when multiplied by 3, gives 4749
• Step 7: Add more zero pairs and repeat the process of finding the new divisor and product as in step 2

1. What is the value of √√√√63
2. Simplify ((√63)^1/2)^1/2

What is the value of square root of 63 up to 2 decimal places?

The square root of 63 up to 2 decimal places is 7.93.

Is square root of 63 a rational number?

√63=7.937
We know that the decimal part does not stop after 7.937
Hence, it is never-ending and you cannot see a pattern in the decimal part.
So √63 is an irrational number.

What is the square number of 63?

Square number of 63 = 63² = 63 × 63 = 3969
Note that √3969 = 63

What is the value of √63?

The value of √63=±7.937

What is the value of √63 × √63?

The value of √63 × √63 = 63