A day full of math games & activities. Find one near you.

Square Root of 3200

The square root of 3200 is expressed as √3200 in the radical form and as (3200)^½ or (3200)^0.5 in the exponent form. The square root of 3200 rounded up to 8 decimal places is 56.56854249. It is the positive solution of the equation x² = 3200. We can express the square root of 3200 in its lowest radical form as 40 √2.

Square Root of 3200: 56.568542494923804
Square Root of 3200 in exponential form: (3200)^½ or (3200)^0.5
Square Root of 3200 in radical form: √3200 or 40 √2

Square Root of 3200

1.	What is the Square Root of 3200?
2.	How to find the Square Root of 3200?
3.	Is the Square Root of 3200 Irrational?
4.	FAQs

What is the Square Root of 3200?

The square root of 3200, (or root 3200), is the number which when multiplied by itself gives the product as 3200. Therefore, the square root of 3200 = √3200 = 40 √2 = 56.568542494923804.

☛ Check: Square Root Calculator

How to Find Square Root of 3200?

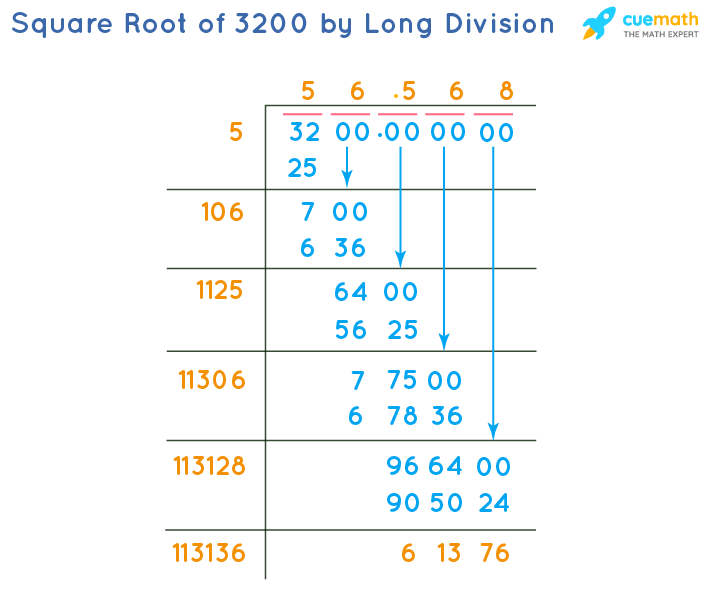
Square Root of 3200 by Long Division Method

Value of √3200 by Long Division Method

Explanation:

Forming pairs: 32 and 00
Find a number Y (5) such that whose square is <= 32. Now divide 32 by 5 with quotient as 5.
Bring down the next pair 00, to the right of the remainder 7. The new dividend is now 700.
Add the last digit of the quotient (5) to the divisor (5) i.e. 5 + 5 = 10. To the right of 10, find a digit Z (which is 6) such that 10Z × Z <= 700. After finding Z, together 10 and Z (6) form a new divisor 106 for the new dividend 700.
Divide 700 by 106 with the quotient as 6, giving the remainder = 700 - 106 × 6 = 700 - 636 = 64.
Now, let's find the decimal places after the quotient 56.
Bring down 00 to the right of this remainder 64. The new dividend is now 6400.
Add the last digit of quotient to divisor i.e. 6 + 106 = 112. To the right of 112, find a digit Z (which is 5) such that 112Z × Z <= 6400. Together they form a new divisor (1125) for the new dividend (6400).
Divide 6400 by 1125 with the quotient as 5, giving the remainder = 6400 - 1125 × 5 = 6400 - 5625 = 775.
Bring down 00 again. Repeat above steps for finding more decimal places for the square root of 3200.

Therefore, the square root of 3200 by long division method is 56.5 approx.

Is Square Root of 3200 Irrational?

The actual value of √3200 is undetermined. The value of √3200 up to 25 decimal places is 56.56854249492380195206755. Hence, the square root of 3200 is an irrational number.

☛ Also Check:

Square Root of 169 - √169 = 13
Square Root of 68 - √68 = 8.24621
Square Root of 30 - √30 = 5.47723
Square Root of 120 - √120 = 10.95445
Square Root of 48 - √48 = 6.92820
Square Root of 52 - √52 = 7.21110
Square Root of 196 - √196 = 14

Square Root of 3200 Solved Examples

Example 1: Solve the equation x² − 3200 = 0

Solution:

x² - 3200 = 0 i.e. x² = 3200
x = ±√3200
Since the value of the square root of 3200 is 56.569,
⇒ x = +√3200 or -√3200 = 56.569 or -56.569.
Example 2: If the surface area of a cube is 19200 in². Find the length of the side of the cube.

Solution:

Let 'a' be the length of the side of the cube.
⇒ Area of the cube = 6a² = 19200 in²
⇒ a = ±√3200 in
Since length can't be negative,
⇒ a = √3200
We know that the square root of 3200 is 56.569.
⇒ a = 56.569 in
Example 3: If the area of a square is 3200 in². Find the length of the side of the square.

Solution:

Let 'a' be the length of the side of the square.
⇒ Area of the square = a² = 3200 in²
⇒ a = ±√3200 in
Since length can't be negative,
⇒ a = √3200 = 56.569 in

go to slide go to slide go to slide

Ready to see the world through math’s eyes?

Math is at the core of everything we do. Enjoy solving real-world math problems in live classes and become an expert at everything.

Book a Free Trial Class

FAQs on the Square Root of 3200

What is the Value of the Square Root of 3200?

The square root of 3200 is 56.56854.

Why is the Square Root of 3200 an Irrational Number?

Upon prime factorizing 3200 i.e. 2⁷ × 5², 2 is in odd power. Therefore, the square root of 3200 is irrational.

Evaluate 4 plus 17 square root 3200

The given expression is 4 + 17 √3200. We know that the square root of 3200 is 56.569. Therefore, 4 + 17 √3200 = 4 + 17 × 56.569 = 4 + 961.665 = 965.665

What is the Square of the Square Root of 3200?

The square of the square root of 3200 is the number 3200 itself i.e. (√3200)² = (3200)^2/2 = 3200.

What is the Square Root of 3200 in Simplest Radical Form?

We need to express 3200 as the product of its prime factors i.e. 3200 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5. Therefore, √3200 = √2 × 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 = 40 √2. Thus, the square root of 3200 in the lowest radical form is 40 √2.

What is the Square Root of -3200?

The square root of -3200 is an imaginary number. It can be written as √-3200 = √-1 × √3200 = i √3200 = 56.568i
where i = √-1 and it is called the imaginary unit.

Math worksheets and
visual curriculum