A day full of math games & activities. Find one near you.

Square Root of 300

The square root of 300 is expressed as √300 in the radical form and as (300)^½ or (300)^0.5 in the exponent form. The square root of 300 rounded up to 9 decimal places is 17.320508076. It is the positive solution of the equation x² = 300. We can express the square root of 300 in its lowest radical form as 10 √3.

Square Root of 300: 17.320508075688775
Square Root of 300 in exponential form: (300)^½ or (300)^0.5
Square Root of 300 in radical form: √300 or 10 √3

1.	What Is the Square Root of 300?
2.	Is Square Root of 300 Rational or Irrational?
3.	How to Find the Square Root of 300?
4.	FAQs on Square Root of 300

What Is the Square Root of 300?

The square root of a number n is written as √n. This number when squared or multiplied by itself results in the original number n. The square root of 300 can be written in multiple ways:

Radical form: √300 = 10√3
Decimal form: 17.320
Exponent form: (300)^1/2

Is Square Root of 300 Rational or Irrational?

300 is a number that is not a perfect square. This indicates that it does not have a natural number as its square root.
Also, its square root cannot be expressed as a fraction of the form p/q which tells us that the square root of 300 is an irrational number.

How to Find the Square Root of 300?

There are 2 important methods to find the square root of 300.

Long Division Method
Prime Factorization

One can find out other methods by clicking here

Long Division Method

The square root of 300 by long division method consists of the following steps:

Step 1: Starting from the right, we will pair up the digits 300 by putting a bar above 00 and 3 separately. We will also pair the 0s in the decimal from left to right.
Step 2: Find a number that when multiplied by itself gives a product less than or equal to 3. In this case, it is 1, so place 1 in the quotient and the divisors place which will result in the remainder being 2.
Step 3: Drag down 00 beside the remainder 2. Also, add the divisor to itself and write it below. (1+1=2)
Step 4: Find a number X such that 2X × X results in a number less than or equal to 200. The number 7 fits here, so place it next to 2 in the divisor as well as next to 1 in the quotient.
Step 5: Find the remainder and now drag down the pair of 0s from the decimal part of the number. Double the quotient to get the new divisor. The new divisor is 34.
Step 6: Repeat this process to get the decimal places you want.

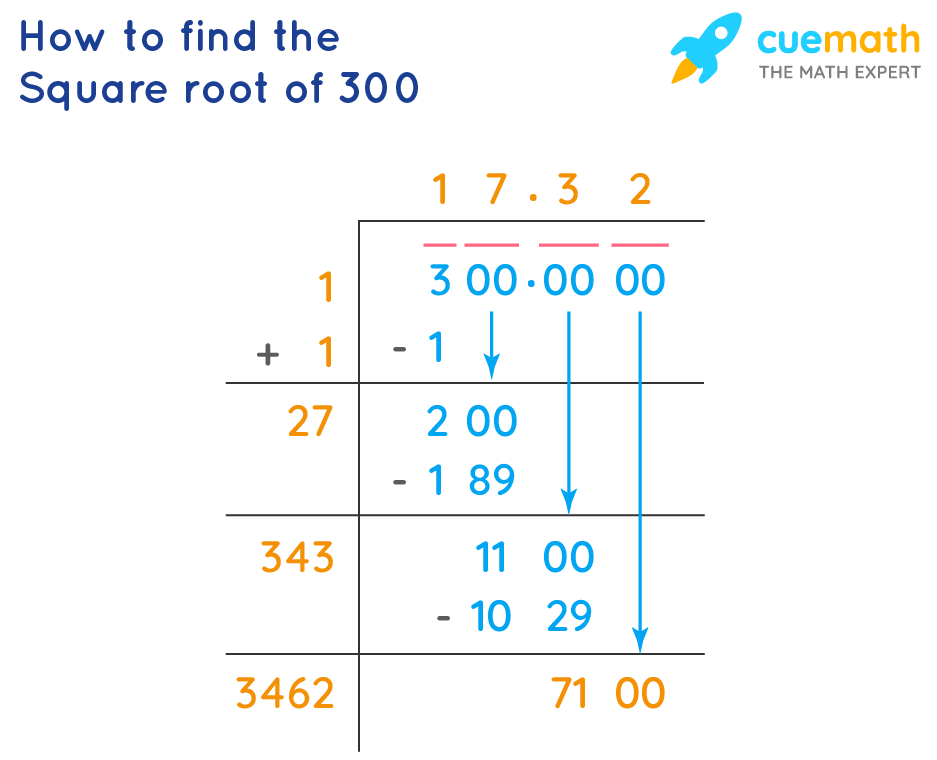
Square Root of 300

Therefore, the square root of 300 = 17.320

Prime Factorization

To find the square root of 300, we will first express it in terms of its prime factors.
300 = 2 × 2 × 3 × 5 × 5.
Next, this can be reduced further to
300 = 2² × 3 × 5²
It is now easy to find the root from here:
√300 = √(2² × 3 × 5²)
√300 = 10√3 = 17.320

Therefore, the square root of 300 ≅ 17.320

Explore square roots using illustrations and interactive examples

Important Notes

There exists a positive and negative root of 300; 17.320 and -17.320
There will be n/2 digits in the square root of an even number with n digits.
There will be (n+2)/2 digits in the square root of an odd number with n digits.

Solved Examples

Example 1: Jake wants to buy a new painting for his living room. In the store, he finds a square painting that has an area of 90,000 sq. cm. How long is each side of the painting?

Solution:

We know that area of a square is equal to side². To find the side of the painting, we will have to find the square root of 90,000.
The square root is √90000 = 300.
Hence, the length of each side of the painting is approximately 300 cm.
Example 2: What is the radius of a circle having an area of 300π square inches?

Solution:

The area of a circle formula is πr². From the given information, we have:
πr² = 300π
r² = 300
r = 17.3
Therefore, the radius of the circle is ≅ 17.3 inches
Example: Solve the equation x² − 300 = 0

Solution:

x² - 300 = 0 i.e. x² = 300
x = ±√300
Since the value of the square root of 300 is 17.321,
⇒ x = +√300 or -√300 = 17.321 or -17.321.

go to slide go to slide go to slide

Breakdown tough concepts through simple visuals.

Math will no longer be a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

FAQs on the Square Root of 300

What is the Value of the Square Root of 300?

The square root of 300 is 17.3205.

Why is the Square Root of 300 an Irrational Number?

Upon prime factorizing 300 i.e. 2² × 3¹ × 5², 3 is in odd power. Therefore, the square root of 300 is irrational.

What is the Square Root of -300?

The square root of -300 is an imaginary number. It can be written as √-300 = √-1 × √300 = i √300 = 17.32i
where i = √-1 and it is called the imaginary unit.

Is the number 300 a Perfect Square?

The prime factorization of 300 = 2² × 3¹ × 5². Here, the prime factor 3 is not in the pair. Therefore, 300 is not a perfect square.

Evaluate 16 plus 7 square root 300

The given expression is 16 + 7 √300. We know that the square root of 300 is 17.321. Therefore, 16 + 7 √300 = 16 + 7 × 17.321 = 16 + 121.244 = 137.244

What is the Square Root of 300 in Simplest Radical Form?

We need to express 300 as the product of its prime factors i.e. 300 = 2 × 2 × 3 × 5 × 5. Therefore, √300 = √2 × 2 × 3 × 5 × 5 = 10 √3. Thus, the square root of 300 in the lowest radical form is 10 √3.

Math worksheets and
visual curriculum