A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
LearnPracticeDownload
 

Square Root of 260

The square root of 260 is expressed as √260 in the radical form and as (260)½ or (260)0.5 in the exponent form. The square root of 260 rounded up to 8 decimal places is 16.12451550. It is the positive solution of the equation x2 = 260. We can express the square root of 260 in its lowest radical form as 2 √65.

  • Square Root of 260: 16.1245154965971
  • Square Root of 260 in exponential form: (260)½ or (260)0.5
  • Square Root of 260 in radical form: √260 or 2 √65
1. What Is the Square Root of 260?
2. Is Square Root of 260 Rational or Irrational?
3. How to Find the Square Root of 260?
4. Important Notes on Square Root of 260
5. FAQs on Square Root of 260

What is the Square Root of 260?

  • The square root of 260 in decimal form is = 16.1245
  • The square root of 260 can be written as √260 and (260)1/2
  • The number 260 is not a perfect square as its square root is not an integer.

Is Square Root of 260 Rational or Irrational?

The square root of 260 is a non-terminating and non-repeating number
Therefore, the square root of 260 is an irrational number because it cannot be expressed in the form of p/q where q ≠ 0.

How to Find the Square root of 260?

Now we will calculate the square root of 260 using the following methods

Square Root of 260 Using Prime Factorization Method

  • The prime factorization of 260: 22 × 5 × 13
  • The prime factors of 260 in pairs: (2 × 2) × 5 × 13
  • Now, the square root of 260: √260 = √((2 × 2) × 5 × 13)
  • So, the square root of 260 = 2 × √(5 × 13) = 2√65

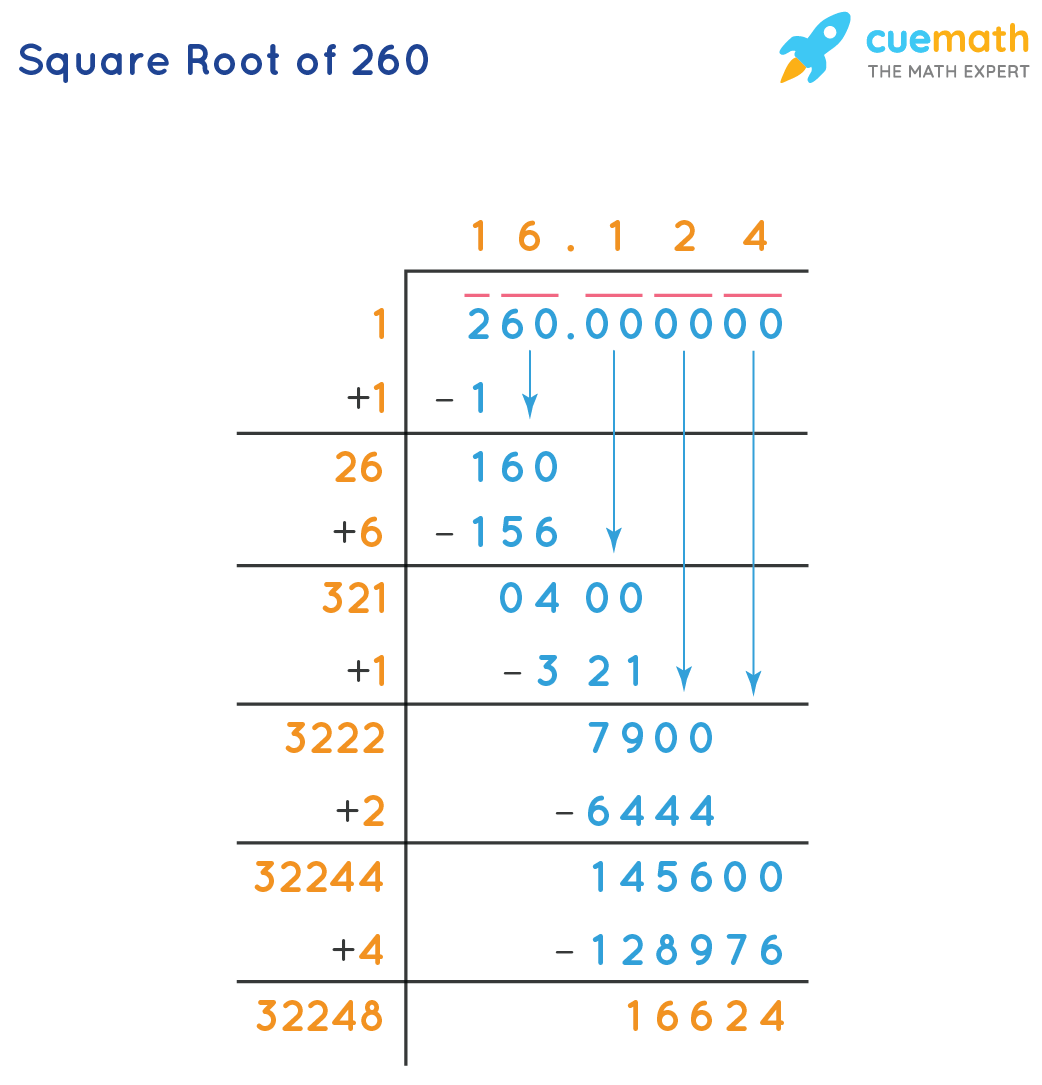
Square Root of 260 By Long Division

  • Start Grouping the digits from the unit’s place in pairs of two by drawing a line on top of them. We get two pairs in this case (2 & 60).
  • Find a number(n) which when multiplied with itself n × n ≤ 2. So, n will be 1 as 1 × 1 = 1.
  • Now we get the quotient and remainder as 1. Also, we have to add the divisor n with itself. Thus, we get the new divisor 2.
  • Bring down the pair of 60. So, our new dividend is 160. Now find a number(m) such that 2m × m ≤ 160. The number m will be 6 as 26 × 6 = 156 ≤ 160. Therefore, the remainder is 4.
  • Add a decimal in the dividend and quotient part simultaneously. Also, add 3 pairs of zero in the dividend part (260. 00 00 00) and repeat the above step for all the pairs of zero.

So, we get the square root of √260 = 16.124 by the long division method.

Square Root of 260

Explore square roots using illustrations and interactive examples

Important Notes:

  • The number 260 is not a perfect square.
  • The square root of 260 is an irrational number.
  • The square root of -260 is an imaginary number.
 

Square Root of 260 Solved Examples

  1. Example 1: Mario wants to find out the square root of -260. Can you help Mario?

    Solution:
    The square root of negative numbers is imaginary numbers.
    Because the square of any number (positive or negative) will result in a positive number.
    So, the square of -260 is written as √-260 = ±16.1245i. (where i = √-1)

  2. Example 2: Find the square root of 260 using the repeated subtraction method?

    Solution:

    • First, find two consecutive perfect squares between which the number 260 will lie. The two perfect squares are 256 (162) and 289 (172).
    • Therefore, the whole number part of the square root of 260 will be 16
    • Now, for the decimal part, we will use the below-mentioned formula
    • (Given number - smaller perfect square) / (Greater perfect square - smaller perfect square)
    • = (260 - 256)/(289 - 256) = 4/33 = 0.12

    Hence, the square root of 260 via the approximation method is 16.12

  3. Example: If the surface area of a sphere is 1040π in2. Find the radius of the sphere.

    Solution:

    Let 'r' be the radius of the sphere.
    ⇒ Area of the sphere = 4πr2 = 1040π in2
    ⇒ r = ±√260 in
    Since radius can't be negative,
    ⇒ r = √260
    The square root of 260 is 16.125.
    ⇒ r = 16.125 in

Check Answer >

go to slidego to slidego to slide

 

How can your child master math concepts?
Math mastery comes with practice and understanding the ‘Why’ behind the ‘What.’ Experience the Cuemath difference.

Book a Free Trial Class

FAQs on the Square Root of 260

What is the Value of the Square Root of 260?

The square root of 260 is 16.12451.

Why is the Square Root of 260 an Irrational Number?

Upon prime factorizing 260 i.e. 22 × 51 × 131, 5 is in odd power. Therefore, the square root of 260 is irrational.

What is the Square of the Square Root of 260?

The square of the square root of 260 is the number 260 itself i.e. (√260)2 = (260)2/2 = 260.

What is the Square Root of 260 in Simplest Radical Form?

We need to express 260 as the product of its prime factors i.e. 260 = 2 × 2 × 5 × 13. Therefore, √260 = √2 × 2 × 5 × 13 = 2 √65. Thus, the square root of 260 in the lowest radical form is 2 √65.

Evaluate 18 plus 16 square root 260

The given expression is 18 + 16 √260. We know that the square root of 260 is 16.125. Therefore, 18 + 16 √260 = 18 + 16 × 16.125 = 18 + 257.992 = 275.992

What is the Value of 4 square root 260?

The square root of 260 is 16.125. Therefore, 4 √260 = 4 × 16.125 = 64.498.

Math worksheets and
visual curriculum