Square Root of 245
Did you know that 245 is an odd composite non-perfect square number. It has more than two factors but 245 cannot be expressed as square of a number. In this lesson, we will learn to calculate the square root of 245 by long division method. We will also go through a few solved examples and interactive questions related to the square root of 245.
- Square Root of 245: √245 = 15.652
- Square of 245: 2452 = 60,025
| 1. | What Is the Square Root of 245? |
| 2. | Is Square Root of 245 Rational or Irrational? |
| 3. | Tips and Tricks |
| 4. | How to Find the Square Root of 245? |
| 5. | FAQs on Square Root of 245 |
| 6. | Challenging Questions |
What Is the Square Root of 245?
The integer which on squaring gives 245 is the square root of 245. There is no such integer which on multiplying with itself gives 245 exactly, hence the square root of 140 is not a whole number.
Is the Square Root of 245 Rational or Irrational?
The square root of 245 is 15.65247 (approximately) which is a non-recurring and non-terminating decimal number. This shows that 245 is not a perfect square which proves that the square root of 245 is an irrational number.
Tips and Tricks:
- The square root of any number n, which is not a perfect square, is always an irrational number. As 245 is not a perfect square, the square root of 245 is an irrational number.
How to Find the Square Root of 245?
As 245 is not a perfect square, the square root of 245 is found using the long division method. The simplified radical form of the square root of 245 is given below.
Simplified Radical Form of Square Root of 245
245 is expressed as the product of 49 and 5. It is given as:
√245 = √(49 × 5) = √(7 × 7 × 5) = 7√5
As we know, 5 is not a perfect square. Hence it stays within the root sign. 49 can be written as 7 × 7. The number repeated within square root is 7. Thus, the simplified radical form of the square root of 245 is 7√5.
Square Root of 245 by Long Division Method
The square root of 245 is found using the long division method. The steps to be followed are:
- Step 1: Pair the digits of 245 starting with a digit at one's place. Put a horizontal bar to indicate pairing.
- Step 2: Now we find a number which on multiplication with itself gives a product of less than or equal to 1. As we know 1 × 1 = 1 < 2.
- Step 3: Now, we have to bring down 45 and multiply the quotient by 2. This give us 2. Hence, 2 is the starting digit of the new divisor. We bring down 45.
- Step 4: 5 is placed at one's place of new divisor because when 25 is multiplied by 5 we get 125. The obtained answer now is 20 and we bring down 00.
- Step 5: The quotient now becomes 15 on multiplication by 2 gives 30, which becomes the starting digit of the new divisor.
- Step 6: 6 is placed at one's place of new divisor because on multiplying 306 by 6 we get 1236. The answer now obtained is 20 and we bring 00 down.
- Step 7: Now the quotient is 15 when multiplied by 2 gives 30, which will be the starting digit of the new divisor.
- Step 8: 6 is placed at one's place of the divisor because on multiplying 156 by 6 we will get 1836. The answer obtained is 164 and we bring 00 down.
- Step 9: Now the quotient is 156 when multiplied by 2 gives 312, which will be the starting digit of the new divisor.
- Step 10: 5 is placed at one's place of the divisor because on multiplying 3125 by 5, we get 15625. The answer obtained is 775 and we bring 00 down.
- Step 11: Now the quotient is 1565 when multiplied by 2 gives 3130, which will be the starting digit of the new divisor.
- Step 12: 2 is placed at one's place of the divisor because on multiplying 31302 by 2, we get 62604. The answer obtained is 14896 and we bring 00 down.
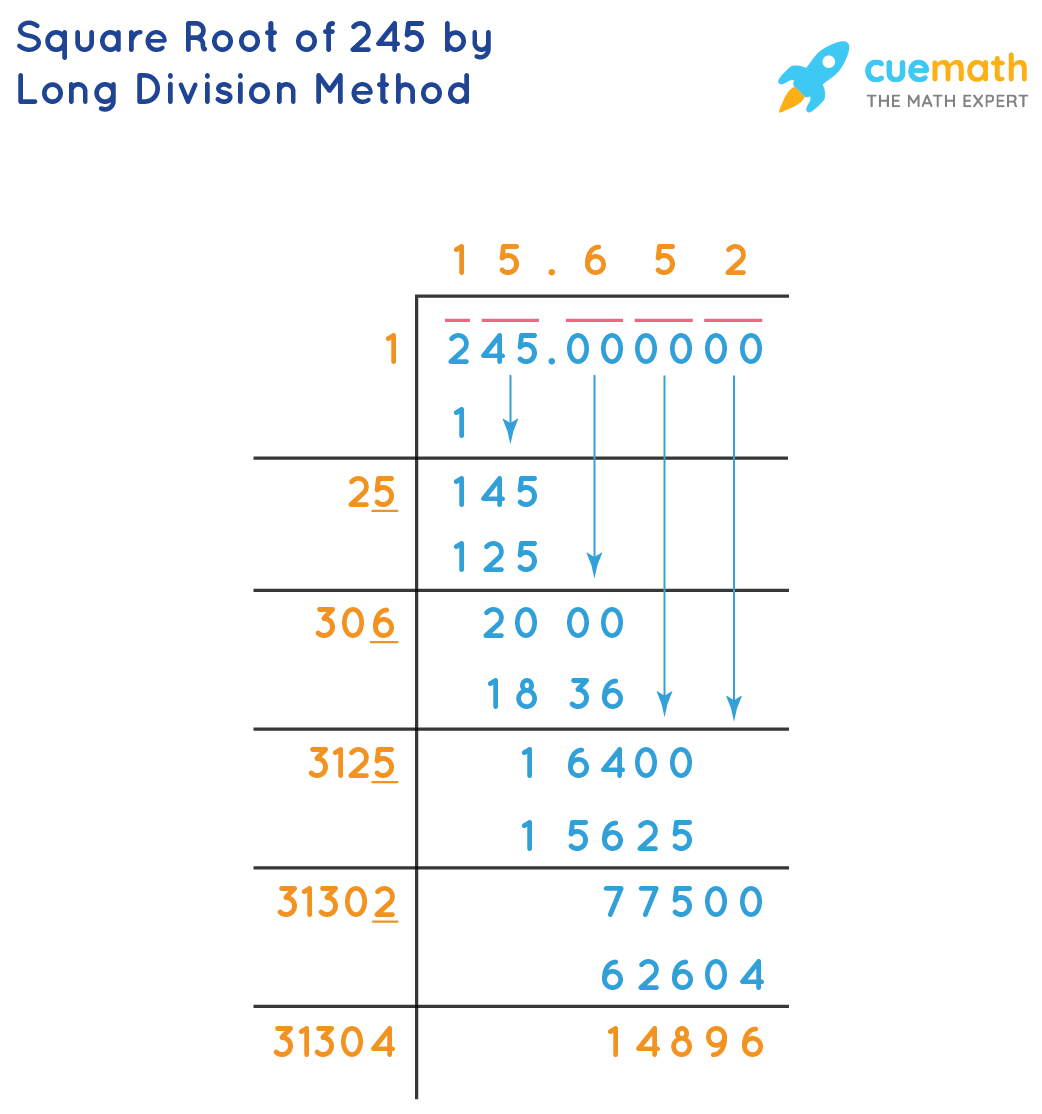
Hence, √245 = 15.652
Explore square roots using illustrations and interactive examples
Challenging Questions:
- Find the square root of 245 using the long division method up to 8 decimal places?
- Can Mandy express the square root of 245 in terms of square root of 735?
Square Root of 245 Solved Examples
-
Example 1: Evaluate (√245 + 4√5) - (√245 - 2√5)
Solution
As we know, √245 = 7√5.
(√245 + 4√5) = (7√5 + 4√5) = 11√5
(√245 - 2√5) = (7√5 - 2√5) = 5√5
Hence, (√245 + 4√5) - (√245 - 2√5) = 11√5 - 5√5 = 6√5. -
Example 2: What is the diameter of circle if the area of circle is 245π square inches?
Solution
The area of circle is given as πr2.
Hence, area = πr2 = 245π ⇒ r2 = 245 ⇒ r = √245 = 15.65 ≈ 15.7 inches.
Diameter of circle = 2r = 2 × 15.7 = 31.4 inches. Hence, the diamter of circle if the area of circle is 245π square inches is 31.4 inches.

FAQs on Square Root of 245
What is the square root of 245 in the simplest form?
The square root of 245 in the simplest form is 2√35.
How do you find the square root of 245?
As 245 is not a perfect square number. Hence, the square root of 245 is obtained by using long division method.
What is approximate square root of 245?
The square root of 245 is 15.6524 (approximately).
Is the square root of 245 rational or irrational?
The square root of 245 is irrational.
Is the square root of 245 a real number?
Yes, the square root of 245 is a real number.
visual curriculum