LearnPracticeDownload
 

Square Root of 225

The square root of 225 is expressed as √225 in the radical form and as (225)½ or (225)0.5 in the exponent form. The square root of 225 is 15. It is the positive solution of the equation x2 = 225. The number 225 is a perfect square.

  • Square Root of 225: 15
  • Square Root of 225 in exponential form: (225)½ or (225)0.5
  • Square Root of 225 in radical form: √225
1. What Is the Square Root of 225?
2. Is Square Root of 225 Rational or Irrational?
3. How to Find the Square Root of 225?
4. FAQs on Square Root of 225

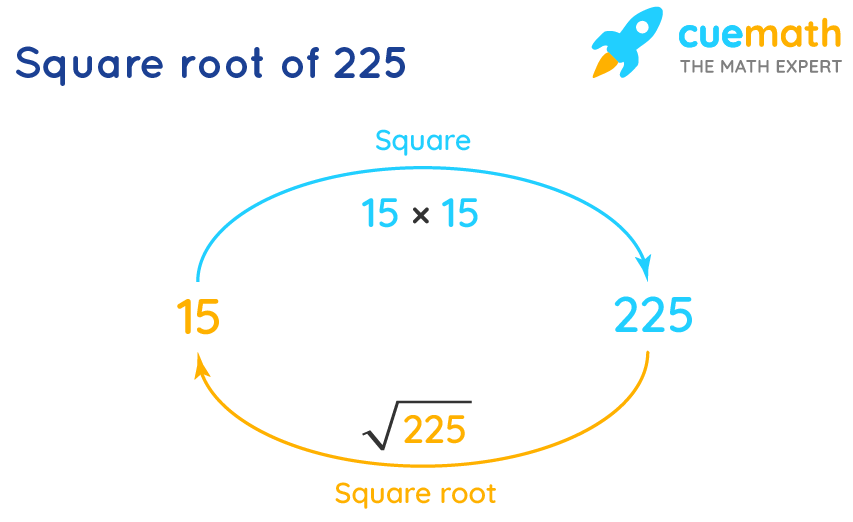
What Is the Square Root of 225?

  • For real numbers a and b,  if a2 = b, we can express a = √b
  • This means that a is the second root of b.
  • The square root of 225 is the inverse operation of squaring 15
  • 15 × 15 = 225 and -15 ×-15 = 225.
  • Therefore, √25 = 15

 

square root of 225 

 

Is the Square Root of 225 Rational or Irrational?

225 can be expressed as the ratio of two integers.
As 225 can be expressed as 225 = 225/1.
Thus 225 is rational.

How to Find the Square Root of 225?

The square root of 225 can be found by various methods.

Square Root of 225 by Prime Factorization

  • Get the prime factorization done for the number 225 by the ladder method or the factor tree method to get the factors.
  • After getting the prime factors, arrange them in such a way that they can be expressed as a product of number x number.
  • √225 = √(5 × 5 × 3 × 3)
  • Squaring on both the sides, we get, √225 =√(52 × 32)
  • This gives, 5 × 3 = 15

Square Root of 225 by Long Division Method

Here are the steps to find the square root of 225

  • Step 1: Write the pair of digits starting from one's place. Here 25 is the pair. 
  • Step 2: On finding a divisor "n" such that n × n results in the product ≤ 2. We find 1 × 1 = 1, follow the process of long division and obtain the remainder. Here it is 1.
  • Step 3: Now, bring down the next pair of numbers. Here it's 25. Multiply the quotient 1 by 2 and write it in the new divisor's place. Here it's 2.
  • Step 4: Find a divisor "n" such that n × n results in the product ≤ 125. Get the next quotient place as 5. Now we get our new divisor as 25, as 5 × 125 = 225. Divide and get the remainder. Here we get 0. Thus finding the square root of 225 by long division is completed. Therefore, 15 is the square root of 225.

finding square root of 225 by division method

Explore Square roots using illustrations and interactive examples

Important Notes:
  • Finding the square root is an inverse process of squaring the number.
  • Irrational numbers cannot be expressed as a ratio of two integers. Example: π and √sqrt 2. This makes 225 is rational as it can be expressed as 225/1.
  • 225 is a perfect square.

Tips and Tricks

  • Subtract from 225 by successive odd natural numbers, until you obtain 0. The number of times you subtract gives you the square root of 225. 
  • Check by the known multiplication fact that 225 = 15 × 15.
 

Square Root of 225 Solved Examples

  1. Example 1: Mark wants to fence his square backyard. He knows his backyard is 225 square feet. How many feet of fencing will Mark need? 

    Solution:

    To fence he needs to know each side of his backyard. All the sides of the fence are equal. 
    Area = Side × Side = 225 sq feet.
    As, 15 × 15 = 225
    Each side will be = 15 feet

    He needs to fence 4 × 15 = 60 sq feet.

  2. Example 2: James wants to buy a new rug for his living room. In the store, he finds a square rug that has an area of 25 sq feet.
    a. How long is each side of the rug?
    b. How many of those rugs are needed to cover an area of 225 square feet?

    Solution

    a. Area is 25 sq feet = side × side sq feet.
    The length of each side of the rug will be 5 feet.

    b. To cover an area of 225 square feet, he needs 225 ÷ 5 = 45 rugs.

  3. Example 3: If the area of a circle is 225π in2. Find the radius of the circle.

    Solution:

    Let 'r' be the radius of the circle.
    ⇒ Area of the circle = πr2 = 225π in2
    ⇒ r = ±√225 in
    Since radius can't be negative,
    ⇒ r = √225
    The square root of 225 is 15.
    ⇒ r = 15 in

View More >

go to slidego to slidego to slide

Break down tough concepts through simple visuals.
Math will no longer be a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

FAQs on the Square Root of 225

What is the Value of the Square Root of 225?

The square root of 225 is 15.

Why is the Square Root of 225 a Rational Number?

Upon prime factorizing 225 i.e. 32 × 52, we find that all the prime factors are in even power. This implies that the square root of 225 is a positive integer. Therefore, the square root of 225 is rational.

Evaluate 5 plus 3 square root 225

The given expression is 5 + 3 √225. We know that the square root of 225 is 15. Therefore, 5 + 3 √225 = 5 + 3 × 15 = 5 + 45 = 50

If the Square Root of 225 is 15. Find the Value of the Square Root of 2.25.

Let us represent √2.25 in p/q form i.e. √(225/100) = 15/10 = 1.5. Hence, the value of √2.25 = 1.5

Is the number 225 a Perfect Square?

The prime factorization of 225 = 32 × 52. Here, all the numbers are in the power of 2. This implies that the square root of 225 is a positive integer. Therefore, 225 is a perfect square.

What is the Square of the Square Root of 225?

The square of the square root of 225 is the number 225 itself i.e. (√225)2 = (225)2/2 = 225.

Math worksheets and
visual curriculum