A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
LearnPracticeDownload

Square Root of 181

The square root of 181 is expressed as √181 in the radical form and as (181)½ or (181)0.5 in the exponent form. The square root of 181 rounded up to 5 decimal places is 13.45362. It is the positive solution of the equation x2 = 181.

  • Square Root of 181: 13.45362404707371
  • Square Root of 181 in exponential form: (181)½ or (181)0.5
  • Square Root of 181 in radical form: √181
1. What Is the Square Root of 181?
2. Is Square Root of 181 Rational or Irrational?
3. How to Find the Square Root of 181?
4. FAQs on Square Root of 181
5. Important Notes on Square Root of 181

What is the Square Root of 181?

  • Square root of 181 = √181 = 13.4536
  • Square root of 181 is expressed as √181 in radical form.
  • Square root of 181 is represented as (181)1/2 in exponential form.

Is Square Root of 181 Rational or Irrational?

  • The square root of 181 is a non-integer number.
  • A number is a rational number when it can be represented in the form of p/q where q ≠ 0.
  • Therefore, the square root of 181 is an irrational number.

How to Find the Square Root of 181?

Let’s calculate the square root of 181 by approximation and long division method.

Square Root of 181 Approximation method

  • Firstly, find two consecutive perfect squares such that 181 lies between them. The two perfect squares are 169 (132) and 196 (142).
    So, the whole number part of the square root of 181 will be 13.
  • Now, for the decimal part we will use the formula:
    (Given number - Smaller perfect square) / (Greater perfect square - smaller perfect square)
    = (181 - 169)/(196 – 169) = 12/27 = 0.44
  • Hence, the approx. the square root of 181 by the approximation method is 13.44

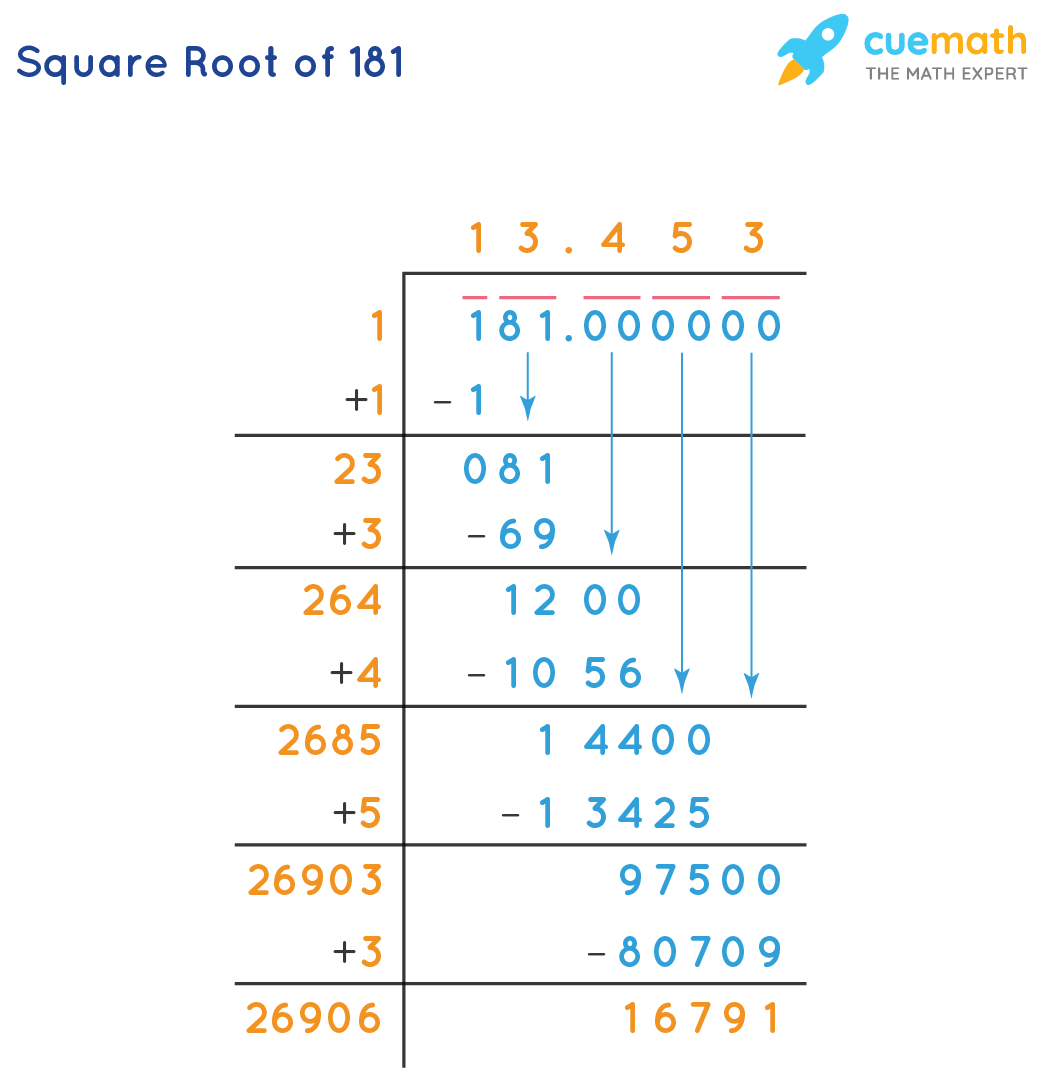
Square Root of 181 By Long Division

  • Write 181 as shown in the figure. Pair the digits from the right end in pairs of two by putting a bar on top of them. So, we got two pairs of 1 and 81.
  • Now find a number (n) such that n × n is a number less than equal to 1. Hence, n = 1.
  • Subtract the obtained value from the first pair in the dividend part and add the divisor with itself (n +n). Bring the second pair down (81).
  • Now find a number (m) such that 2m × m is less than equal to 81. Here m will be 3 as 23 × 3 = 69.
  • Put a decimal after 181 in the dividend part and 13 in the quotient part simultaneously. Add three pair of zeros after 181 (181. 00 00 00)
  • Repeat the step 3&4 for the three pair of zeros.

So, we get a square root of √181 = 13.453 by the long division method.

Square Root of 181

Explore square roots using illustrations and interactive examples

Important Notes:

  • There will be n/2 digits in the square root of an even number with n digits.
  • There will be (n+2)/2 digits in the square root of an odd number with n digits.
  • 181 is not a perfect square.

Challenging Questions:

  • Find the square root of the first three multiples of 181?
  • Find the square root of -181?
 

Square Root of 181 Solved Examples

 

  1. Example 1: Jason has land with an area of 181 square yards and he wants to make two different square-shaped fields with an area of 100 and 81 square yards in that land. What is the length of the sides of the square-shaped fields?

    Solution:
    Area of the land = 181 sq. yards
    Area of smaller field = 81 sq. yards
    Length of the side of smaller field = √81 = 9 yards
    Area of bigger field = 100 sq. yards
    Length of the side of bigger field = √10 = 100 yards

  2.  

    Example 2: Find the value of √100 + √81 and compare it with √181.

    Solution:
    The square root of 100 = 10
    The square root of 81 = 9
    The square root of 181 = 13.4536
    Therefore, √181 < (√100 + √81).

  3. Example: If the area of a square is 181 in2. Find the length of the side of the square.

    Solution:

    Let 'a' be the length of the side of the square.
    ⇒ Area of the square = a2 = 181 in2
    ⇒ a = ±√181 in
    Since length can't be negative,
    ⇒ a = √181 = 13.454 in

View Answer >

go to slidego to slidego to slide

 

Ready to see the world through math’s eyes?
Math is a life skill. Help your child perfect it through real-world application.

Book a Free Trial Class

FAQs on the Square Root of 181

What is the Value of the Square Root of 181?

The square root of 181 is 13.45362.

Why is the Square Root of 181 an Irrational Number?

The number 181 is prime. This implies that the number 181 is pairless and is not in the power of 2. Therefore, the square root of 181 is irrational.

What is the Square of the Square Root of 181?

The square of the square root of 181 is the number 181 itself i.e. (√181)2 = (181)2/2 = 181.

What is the Square Root of 181 in Simplest Radical Form?

The number 181 is a prime number. This implies that the number 181 is pairless and is not in the power of 2. Therefore, the radical form of square root of 181 cannot be simplified further.

What is the Value of 14 square root 181?

The square root of 181 is 13.454. Therefore, 14 √181 = 14 × 13.454 = 188.351.

If the Square Root of 181 is 13.454. Find the Value of the Square Root of 1.81.

Let us represent √1.81 in p/q form i.e. √(181/100) = 1.81/10 = 1.345. Hence, the value of √1.81 = 1.345

Math worksheets and
visual curriculum