A day full of math games & activities. Find one near you.

Square root of 180

180 can be expressed as the sum of six consecutive prime numbers. 180 = 19 + 23 + 29 + 31 + 37 + 41. In this mini-lesson, we will learn to calculate the value of square root using long division method and we will find the simplified radical form of the square root of 180.

Square Root of 180: √180 = 13.416
Square of 180: 180² = 32400

1.	What Is the Square Root of 180?
2.	Is Square Root of 180 Rational or Irrational?
3.	How to Find the Square Root of 180?
4.	Important Notes on Square Root of 180
5.	FAQs on Square Root of 180
6.	Challenging Questions on Square Root of 180

What Is the Square Root of 180?

Finding square root of a number 'n' = the inverse of squaring the number 'a'
If a²= n , then a = ±√n.
(13.416)² = 180 and √180 = ± 13.416

Is Square Root of 180 Rational or Irrational?

180 is not a perfect square as √180 = 6√5.
The square root of 180 cannot be expressed as a whole number; it can only be expressed as a non-terminating decimal.
√180 = 13.41640786499874
Therefore, √180 is an irrational number.

How to Find the Square Root of 180?

The square root of 180 or any number can be calculated in many ways. A few of the common methods are prime factorization method, the average method, and the long division method.

Square Root of 180 by Average Method

Take two perfect square numbers such that one is a little smaller than 180 and the other just a little greater than 180. √169 < √180 < √196
13 < √ 180 < 14
Using the average method, divide 180 by 13 or 14
Let us divide by 14 ⇒ 180 ÷ 14 = 12.85
Find the average of 12.85 and 14
(12.85 + 14 ) / 2 = 26.85 ÷ 2 = 13.42
√180 ≈ 13.42

Square Root of 180 by the Long Division Method

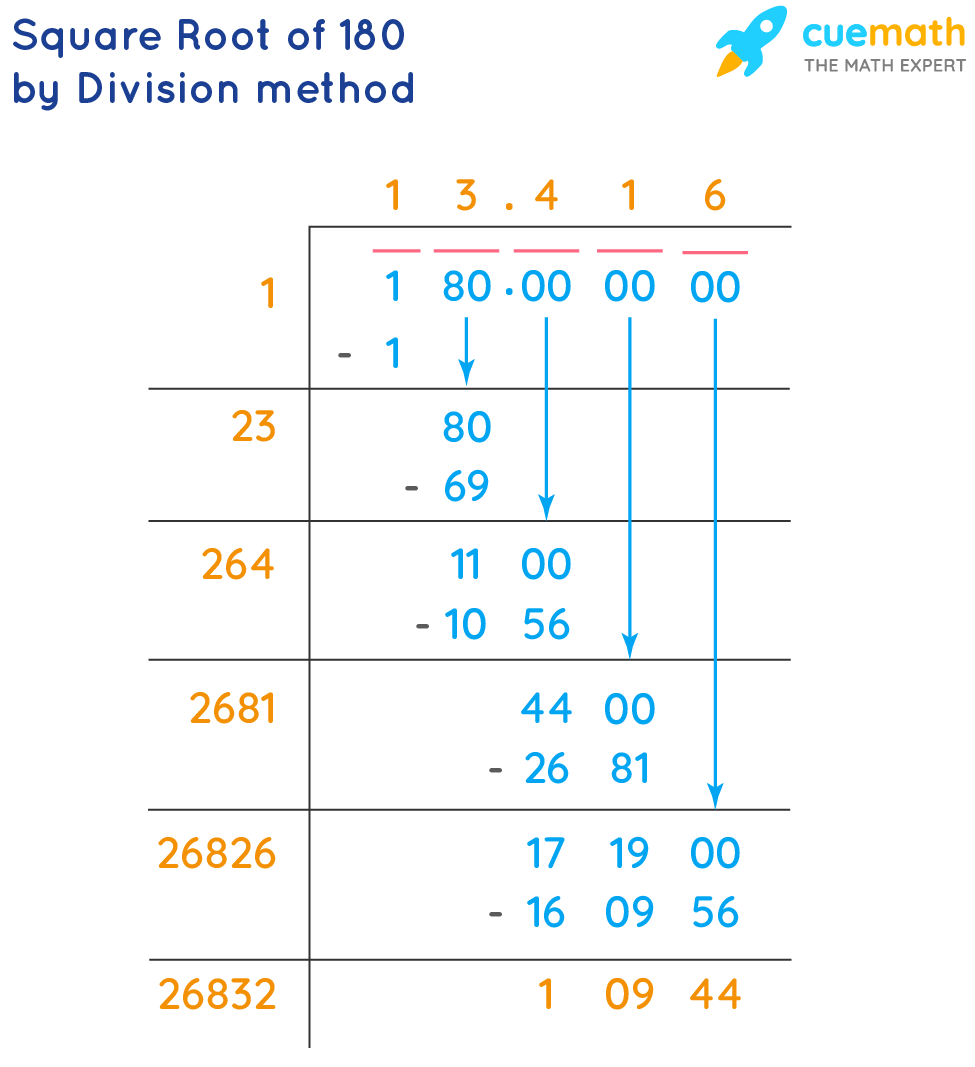
Let's see how to find the square root of 180 by the long division method. Using this method, we can determine its accurate value.

Step 1: Express 180 as 180.000000. We take the number in pairs from the right. 80 stands as a pair while 1 stands alone.
Step 2: Now find a quotient which is the same as the divisor. 1 × 1 = 1. Multiply quotient and the divisor and subtract the result from 1.
Step 3: Now double the quotient obtained in step 2, which is, 1 × 2 = 2. The new divisor is now 2x. Bring down 80 as a pair and this will be the new dividend.
Step 4: Find 2x × x such that the product is less than or equal to 80. We find 23 × 3 = 69. Subtract this from 80 and obtain the remainder. It is 11.
Step 5: Apply decimal after quotient '13' and bring down two zeros. We have 1100 as the dividend now. The new divisor is 13 × 2 = 26x
Step 6: Find 26x × x which would give us the product less than/equal to 1100. 264 × 4 = 1056. Subtract this from 1100 and obtain the remainder as 44.
Step 7: Repeat the process until we get 3 decimal places in the quotient. Thus √ 180 = 13.416

square root of 180 by division method

Explore square roots using illustrations and interactive examples :

Important Notes

The square root of 180 is 13.416 approximated to 3 decimal places.
The simplified form of √180 in its radical form is 6√5
√180 is an irrational number.

Challenging Questions

Find the smallest number by which 180 must be multiplied to get a perfect square. Determine the square root of the perfect square obtained.
Evaluate √180 + 2√180 in the radical form.

Example 1: Sally has plans to decorate one wall of her room by putting borders along the top. If the square wall measures about 180 square feet, what is the length of the border she needs for the decor to the nearest tenth?

Solution:

The length of the border she needs = side of the wall

side² = area of the wall

side = √area = √180 = 13.416

The length of the border to the nearest tenth = 13.4
Example 2: Evaluate: x²= 180 ÷ 45

Solution:

x = √(180 ÷ 45) =

= √180 ÷ √45

√180 = √( 2 × 2 × 3 × 3 × 5) = 6 √5

√45 = √3 × 3 × 5) = 3 √5

√180 ÷ √45 = 6 √5 ÷ 3 √5

= 6 ÷ 3 = 2

Thus x = 2
Example 3: Mia walks 6 miles North and then 12 miles East. How far has she walked away from her starting point?

Solution:

6 miles North and 12 miles East could be assumed as the two legs of the right triangle.

Joining the other ends of the legs, i.e., the hypotenuse, gives the distance travelled by Mia.

By Pythagorean Theorem, we know that Hypotenuse²= leg1² + leg2²

Distance²= 6² + 12²

= 36 + 144 = 180

Distance²= 180

Distance = √180 = 13.416 miles

go to slide go to slide go to slide

Breakdown tough concepts through simple visuals.

Math will no longer be a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

go to slide go to slide go to slide

FAQs on Square Root of 180

What is the simplified form of square root of 180?

The square root of 180 in its simplest radical form is 6√5.

How do you find the square root of 180 in its simplified form?

√180 = √( 2 × 2 × 3 × 3 × 5) = 2 × 3 × √5 = 6

How do you find the square root of 180?

We find the accurate value of square root of 180 by the long division method.

Is √180 a rational number?

√180 is an irrational number because the value of √180 is a non-terminating decimal = 13.41640786499874

Between which two whole numbers does the square root of 180 lie?

The square root of 180 lies between 13 and 14.

Math worksheets and
visual curriculum