A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
A day full of math games & activities. Find one near you.
LearnPracticeDownload

Square Root of 121

The square root of 121 is expressed as √121 in the radical form and as (121)½ or (121)0.5 in the exponent form. The square root of 121 is 11. It is the positive solution of the equation x2 = 121. The number 121 is a perfect square.

  • Square Root of 121: 11
  • Square Root of 121 in exponential form: (121)½ or (121)0.5
  • Square Root of 121 in radical form: √121
1. What Is the Square Root of 121?
2. Is Square Root of 121 Rational or Irrational?
3. How to Find the Square Root of 121?
4. FAQs on Square Root of 121

What Is the Square Root of 121?

We know that addition has an inverse operation in subtraction and multiplication has an inverse operation in the division. Similarly, finding the square root is an inverse operation of squaring. The square root of 121 is the number that gets multiplied to itself to give the number 121.

Is the Square Root of 121 Rational or Irrational?

A rational number is a number that can be expressed in the form of p/q, where p and q are integers and q is not equal to 0. We already found that √121 = 11. The number 11 is a rational number. So, the square root of 121 is a rational number.

How to Find the Square Root of 121?

We will discuss two methods of finding the square root of 121

  • Prime Factorization
  • Long division

Square Root of 121 By Prime Factorization

Prime factorization is a way of expressing a number as a product of its prime factors. The prime factorization of 121 is 121 = 11 × 11 = 112. To find the square root of 121, we take one number from each pair of the same numbers and we multiply them.

  • 121 = 11 × 11 
  • √121 = 11

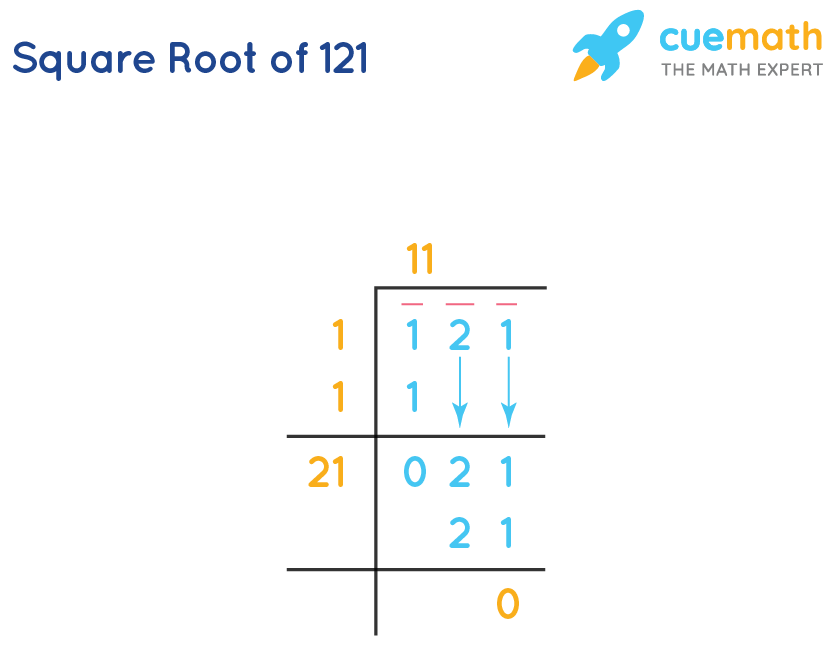
Square Root of 121 By Long Division

The value of the square root of 121 by long division method consists of the following steps:

  • Step 1: Starting from the right, we will pair up the digits by putting a bar above them.
  • Step 2: Find a number which, when multiplied to itself, gives the product less than or equal to 1. So, the number is 1. Putting the divisor as 1, we get the quotient as 1 and the remainder 0
  • Step 3: Double the divisor and enter it with a blank on its right. Guess the largest possible digit to fill the blank which will also become the new digit in the quotient, such that when the new divisor is multiplied to the new quotient the product is less than or equal to the dividend. Divide and write the remainder.

square root of 121 by long division

Explore Square roots using illustrations and interactive examples

Important Notes:

  • The square root is the inverse operation of squaring.
  • We can find the square root of 121 using prime factorization, repeated subtraction, and the long division method.
  • The square root of a number is both negative and positive for the same numerical value i.e., the square root of 121 will be 11.

Think Tank:

  • We know that (-11) × (-11) =121. So, can we say that -11 is a square root of 121?
  • Can you determine a quadratic equation whose roots are 121 and -121?
 

Square Root of 121 Solved Examples

  1. Example 1: Tim's teacher asked him to find the square root of 121 using the prime factorization method. Can you help him do this?

    Solution

    Prime factorization of 121 = 11 × 11 
    Prime factors of 11 in pairs: (11 × 11) 
    Square root of 121: √(11 × 11) = √(112)
    Therefore, √121 = 11

  2. Example 2: A gardener bought 121 plants. He wants to plant them in such a way that the number of plants in each row and column is the same. How many plants will he plant in each row? 

    Solution

    If the gardener plants an equal number of plants in each row and column, then we need to find a number whose square is 121

    We know that, √121 = 11

    Hence, the gardener will plant 11 plants in each row

  3. Example: If the area of a circle is 121π in2. Find the radius of the circle.

    Solution:

    Let 'r' be the radius of the circle.
    ⇒ Area of the circle = πr2 = 121π in2
    ⇒ r = ±√121 in
    Since radius can't be negative,
    ⇒ r = √121
    The square root of 121 is 11.
    ⇒ r = 11 in

View More >

go to slidego to slidego to slide

Breakdown tough concepts through simple visuals.
Math will no longer be a tough subject, especially when you understand the concepts through visualizations.

Book a Free Trial Class

FAQs on the Square Root of 121

What is the Value of the Square Root of 121?

The square root of 121 is 11.

Why is the Square Root of 121 a Rational Number?

Upon prime factorizing 121 i.e. 112, we find that all the prime factors are in even power. This implies that the square root of 121 is a positive integer. Therefore, the square root of 121 is rational.

What is the Square Root of -121?

The square root of -121 is an imaginary number. It can be written as √-121 = √-1 × √121 = i √121 = 11i
where i = √-1 and it is called the imaginary unit.

Evaluate 18 plus 6 square root 121

The given expression is 18 + 6 √121. We know that the square root of 121 is 11. Therefore, 18 + 6 √121 = 18 + 6 × 11 = 18 + 66 = 84

What is the Square of the Square Root of 121?

The square of the square root of 121 is the number 121 itself i.e. (√121)2 = (121)2/2 = 121.

If the Square Root of 121 is 11. Find the Value of the Square Root of 1.21.

Let us represent √1.21 in p/q form i.e. √(121/100) = 11/10 = 1.1. Hence, the value of √1.21 = 1.1

Math worksheets and
visual curriculum