Square root of 116

The square root of 116 is a number which gives the product as 116 when the number is multiplied by itself. That number can be an integer or a decimal. Finding the square root of 116 is raising it to the power half. In this mini lesson, let us learn about the square root of 116, find out whether the square root of 116 is rational or irrational, and see how to find the square root of 116 by long division method. 

• Square Root of 116: √116 = 10.770
• Square of 116: 116² = 13,456

• Finding the square root of a number, say 'n', is finding that number, such that 'a' multiplied by itself equals the number 'n'. a × a = n ⇒ a² = n. Thus, a = √n. 
•  √116 = √(a × a). √116 = √(10.770 × 10.770) or √(-10.770 × -10.770) ⇒ √116 = ±10.770
• We know that on prime factorization, 116 = 2 × 2 × 29. Thus, in the simplest radical form √116 =  √(2 × 2 × 29) =  2√29

Irrational numbers are the real numbers that cannot be expressed as the ratio of two integers. √116 = 10.77032961426901 and hence, the square root of 116 is an irrational number where the numbers after the decimal point go up to infinity.

The square root of 116 or any number can be calculated in many ways. Two of them are the approximation method and the long division method.

Square Root of 116 by Approximation Method

• Take two perfect square numbers which are just smaller than 116 and just greater than 116. √100 < √116 < √121
• 10 <  √116 < 11
• Using the average method, divide 116 by 10 or 11.
• Let us divide by 11 ⇒ 116 ÷ 11 = 10.54
• Find the average of 10.54 and 11.
• (10.54 + 11) / 2 = 21.54 ÷ 2 = 10.77
• √116 ≈ 10.77 

Square Root of 116 by the Long Division Method

The long division method helps us find a more accurate value of the square root of any number. The following are the steps to evaluate the square root of 116 by the long division method.

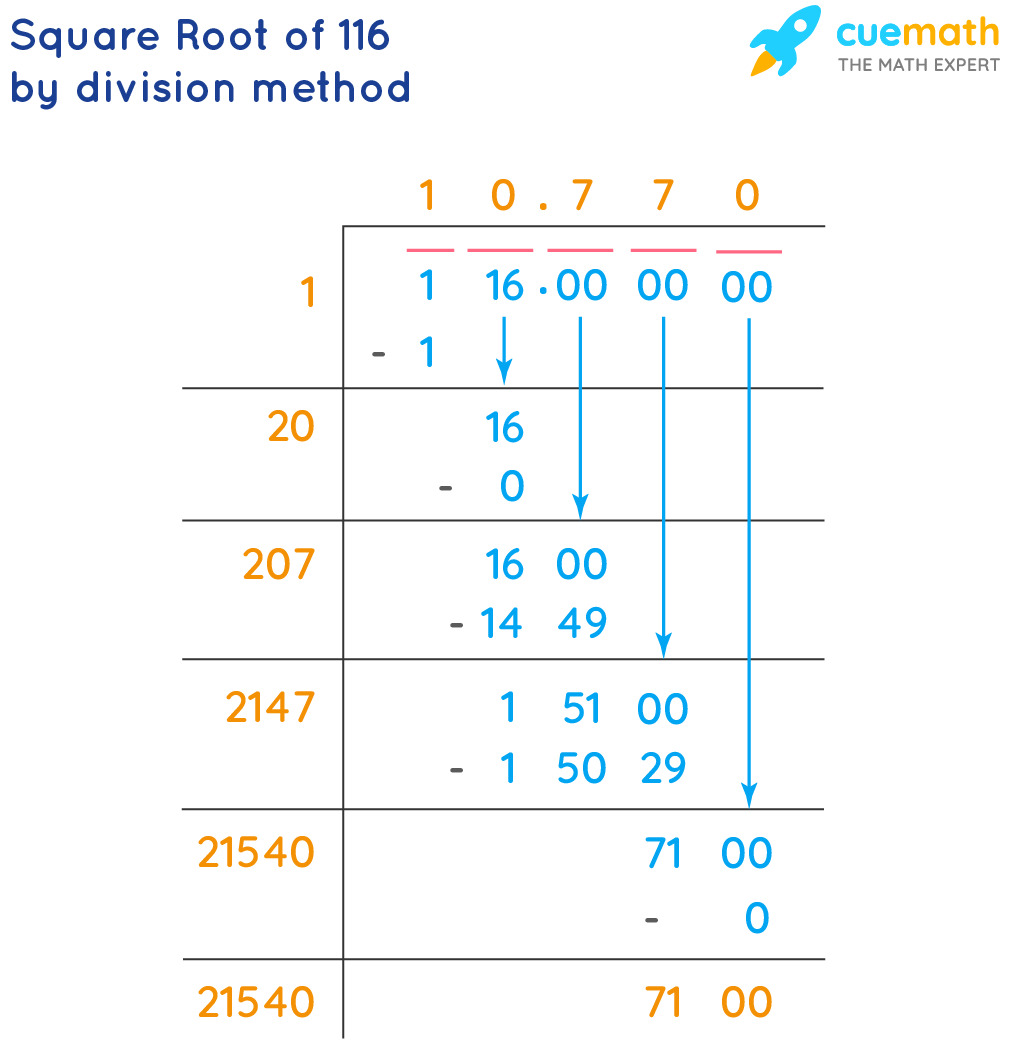
• Step 1: Write 116.000000. Take the number in pairs from the right. 1 stands alone. Now divide 1 by a number such that (number × number) gives ≤ 1.
• Obtain quotient = 1 and remainder = 0. Double the quotient. We get 2. Our new divisor is 20. Bring down 16 for division.
• Step 2: Find a number such that (20 + that number) × that number gives the product ≤ 16.  We cannot find a number that divides 16. Bring down two zeros. 1600 is our new dividend.
• 10 is our quotient and doubling it, we get 200 as our new divisor. Find a number such that (200 + the number) × number gets 1600 or less than that. We find 7 is such number. 207 × 7 = 1449
• Step 3: Quotient is 10.7 and the remainder is 151. Bring down the next pair of zeros and 15100 becomes the new dividend. 
• Double the quotient. 107 × 2 = 214. Have 2140  in the place of the new divisor. Find a number such that (2140 + that number) × number ≤ 15100.
• We find 2147 × 7 = 15029. Subtract this from 15100 and get the remainder. 
• Repeat the steps until we approximate the square root to 3 decimal places. √116 =  10.770

Explore square roots using illustrations and interactive examples: 

• Square root of 98
• Square root of 54
• Square root of 74
• Square root of 180
• Square root of 120

• The square root of 116 lies between the square root of 100 and 121. Therefore, 10 < √116 < 11. We can use the average method to evaluate the approximate value of √116.
• 16 is the least number to be subtracted and 5 is the least number to be added to 116 to make it a perfect square. (116 - 16 = 100) and (116 + 5 = 121)