Square Root of 105
The square root of 105 is the inverse of the square of a number (a) such that a × a = 105. The number 105 have both positive and negative square root. The square root can be a real or imaginary number. We will now find the value of the square root of 105 using the long division method and the approximation method.
- Square root of 105: √105 = 10.24695
- Square of 105: (105)2 = 11025
| 1. | What Is the Square Root of 105? |
| 2. | Is Square Root of 105 Rational or Irrational? |
| 3. | How to Find the Square Root of 105? |
| 4. | Important Notes on Square Root of 105 |
| 5. | FAQs on Square Root of 105 |
What is the Square Root of 105?
- The square root of 105 in decimal form is 10.2469
- The square root of 105 is expressed as √105 in radical form.
- The square root of 105 is expressed as (105)1/2 in exponential form.
Is Square Root of 105 Rational or Irrational?
- The square root of 105 is a non-terminating and non-repeating number.
- Hence, it cannot be represented in the form of p/q where q ≠ 0.
- Therefore, the square root of 105 is an irrational number.
How to Find the Square Root of 105?
We will now find the square root of 105 using the below-given methods:
Square Root of 105 Using Approximation Method
- Find two consecutive perfect squares among which 105 lies.
In this case, the numbers are 10 (100) and 11 (121).
So, the whole number part of the square root of 105 is 10 - Now, for the decimal part we will use the below-given formula:
(Given number – Smaller perfect square) / (Greater perfect square – smaller perfect square)
= (105 – 100)/(121 – 100) = 5/21 = 0.238 - Hence, the approx. value of the square root of 105 by the approximation method is 10.238
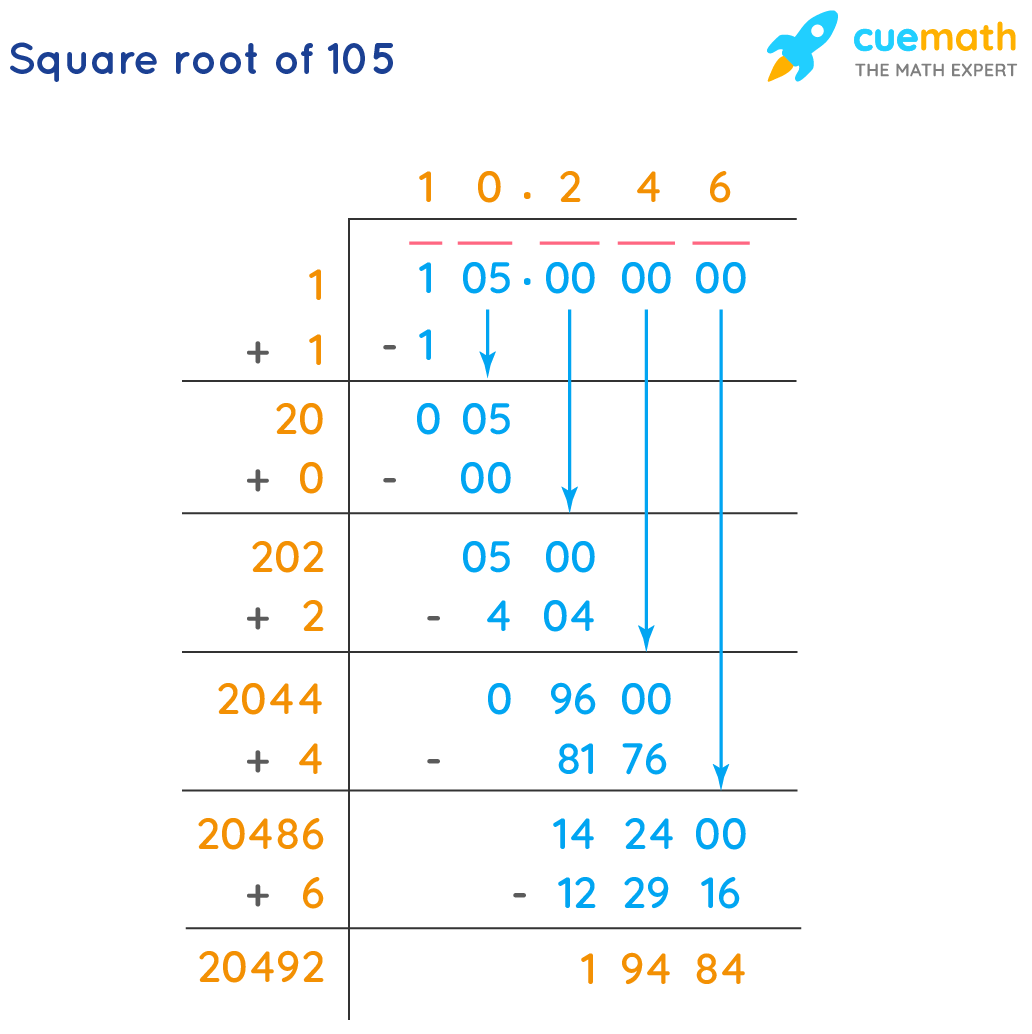
Square Root of 105 By Long Division
Now we will calculate the square root of 105 by the long division method.
- Start pairing the digits by placing a bar on top of them from the right side of number 105 in pairs of two. Here we will have two pairs 05 and 1(pairing from right).
- Now, find a number(n) whose square is ≤ 1. The value of n will be 1 as 1 × 1 = 1≤ 1.
- We get the quotient (1). Now, by adding divisor n with itself and get the new divisor 2n (2).
- Drag the next pair down (new dividend becomes 005) and find a number (A) such that 2A × A ≤ 5. In this case, the value of A will be 0.
- Now, put a decimal after 5 in the dividend part and after 1 in the quotient part simultaneously. Also, place 3 pairs of zero in the dividend after the decimal (105. 00 00 00) and repeat the above step for the remaining three pairs of zero.
So, we get the value of the square root of √105 = 10.246 by the long division method.
Explore square roots using illustrations and interactive examples
Important Notes:
- The square root of 105 is an irrational number.
- The number 105 is not a perfect square.
- The square root of -105 is an imaginary number.
Square Root of 105 Solved Examples
-
Example 1: What is the square root of -√105?
Solution:
The square root of negative numbers is represented by imaginary numbers
Because the square of any positive or negative number is a positive number
So, the square root of -√105 is written as √105 i. (where i = -√1) -
Example 2: Tom wants to find the value of (3√35/√105). Can you help Tom?
Solution:
We know that √105= √35 × √3
And 3 = √3 × √3
Now (3√35/√105) = (√3 × √3 × √35) / (√35 × √3)
= √3

FAQs on Square Roots of 105
What is the square of 105?
The square of 105 is (105)2 = 11025.
What is the negative square root of 105?
The negative square root of 105 is -10.2469
What is the value of square root of 105?
The square root of 105 is 10.2469.
Is square root of 105 rational or irrational?
The square root of 105 is irrational.
Is the number 105 a perfect square?
No, 105 is not a perfect square
Because the square root of 105 is not a natural number.
visual curriculum