Square Root of 10

Square root of 10 is written as √10. It lies between the two numbers, that is, 3 and 4. In this mini-lesson, we will explore all about the square root of 10 by learning the square root rules, finding the square root by long division method, and applying these concepts to solve problems. As we go along, we will also discover interesting facts about them.

Let us see what the square root of 10 is.

• Square Root of 10: √10 = 3.16227
• Square of 10: 10² = 100

1.	What Is the Square Root of 10?
2.	Is Square Root of 10 Rational or Irrational?
3.	How to Find the Square Root of 10?
4.	Tips and Tricks
5.	Challenging Questions
6.	FAQs on Square Root of 10

The square root of any number n can be written as √n. This indicates that there is a number a such that:

• a × a = n

Now, it can also be written as:

• a² = n
• a = √n

Here, a is called as the square root of n. Now, if n = 10, then a = √10 is the square root of 10.

• The square root of 10 is 3.16227.

---

The square root of 10 is an irrational number with never-ending digits. The square root of numbers which are perfect squares like 9, 16, 25, and 100 are integer numbers, but the square root of numbers which are not perfect squares are irrational with never-ending digits. The value of the square root of 10 in decimal form is 3.16227... The square root of any number has two values; one is positive and the other is negative.

• √10 = 3.16227 and √10 = - 3.16227

---

The square root of 10 or any number can be calculated in many ways. Here are two methods: approximation (Hit and Trial) and long division method. 

Let's see how to find √10 by approximation method:

• √9 < √10 < √16
• 3 < √10 < 4

Multiply each by 10.

• 30 < 10 √10 < 40
• √900 < 10√10 < √1600

Let's move closer to the inequality.

• √961 < 10√10 < √1024
• 31 < 10√10 < 32

Divide each by 10.

• 3.1 < √10 < 3.2

Take the average of both lower and upper limits.

• √10 approx. = (3.1+3.2)/2
• √10 approx. = 3.15

Square Root of 10 By Long Division

The long division method helps us find more accurate values of the square roots of any number. Let's see how to find the square root of 10 using the long division method.

• Step 1: Divide the number 10 by 3 (because 3² = 9 is a perfect square number less than 10)
• Step 2: Take the same divisor 3 as the quotient. Multiply the quotient and the divisor and subtract the result from 10.
• Step 3: Take the same quotient 3 and add with the divisor 3. 
• Step 4: Add a decimal point after the quotient 3 and bring down two zeros and place it after 1 so that it becomes 100. We need to find a digit which, when placed at the end of 6 and multiplying the resultant number with the same digit, gives a number less than 100.
  61 × 1 = 61
  Subtract 61 from 100.
  100 - 61 = 39
• Step 5: Bring down two zeros again and place it after 39, so that it becomes 3900. Take 1 and add it to 61. 61 + 1 = 62. We need to again find a number that when placed at the end of 62 and when the result is multiplied with the same number, we get a number less than 3900.
  626 × 6 = 3756
• Write the same number after 1 in the quotient. Subtract 3756 from 3900.
  3900 - 3756 = 144

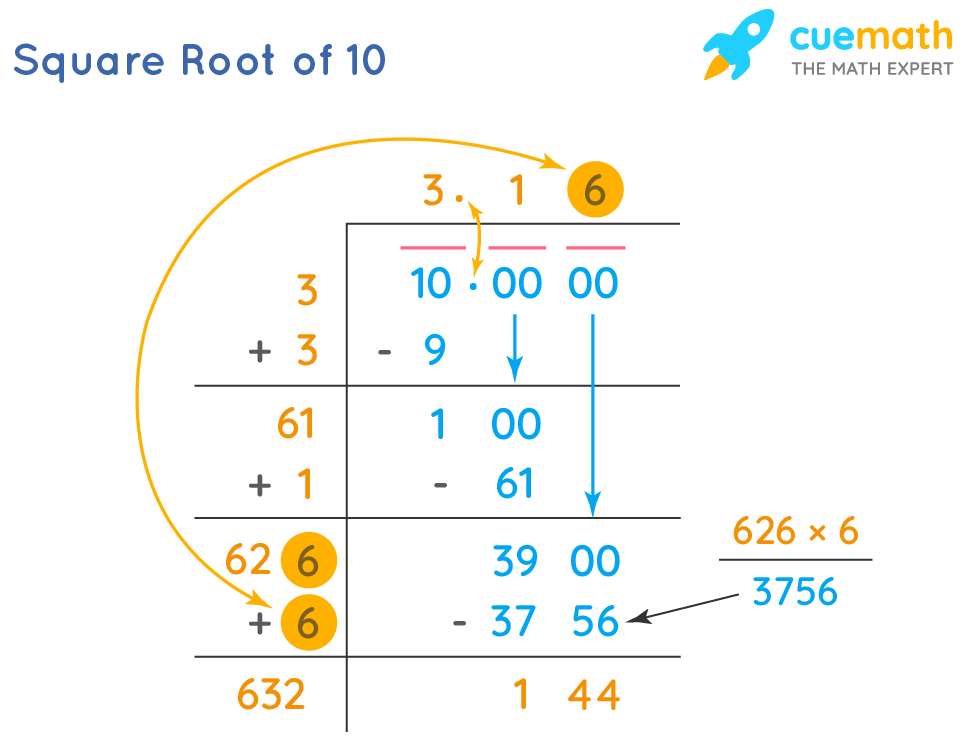
• Step 6: Repeat the process until we get the remainder equal to zero.

The square root of 10 up to two places is obtained by the long division method.

Explore square roots using illustrations and interactive examples

• Square Root of 120
• Square Root of 100
• Square Root of 1000
• Square Root of 30
• Square Root of 60

• Use the hit and trial method while calculating the square root of any number.
• The square root of any number can be assumed as the number between the square roots of the two nearest perfect squares of that number.
  For example, square root of 54 lies between the square roots of 49 and 64.
  i.e. √49 < √54 < √64
  7 < √54 < 8
• Square root of a number n can have two values ±√n

• Find the square root of 1000 by approximation method.
• Find the square root of 10000 by long division method.

 

What is the square root of 10?

The square root of 10 is 3.16227.

What is the square of 10?

The square of 10 is 100.

10² = 100

How do you work out the square root of 10?

We can find the square root of 10 using various methods.

1. Repeated Subtraction 
2. Prime Factorization
3. Estimation and Approximation
4. Long Division

If you want to learn more about each of these methods, click here.

What is the value of square root of 10 up to 3 decimal places?

The square root of 10 up to 3 decimapl place is √10 = 3.162

What is the square root of 10 in the simplest radical form?

√10 is the simplest radical form.

Is the square root of 10 a rational number?

√10 = 3.16227. √10 is an irrational number.