Simplifying Exponents
Simplifying exponents is a method of simplifying the algebraic expressions involving exponents into a simpler form such that they cannot further be simplified. There are rules in algebra for simplifying exponents with different and same bases that we can use. Various arithmetic operations like addition, subtraction, multiplication, and division can be applied to simplify exponent algebraic expressions, exponents in fractions, and negative exponents using the laws of exponents.
In this article, we will learn how to simplify exponents in algebraic expressions, fractions, negative exponents, and with different bases using the simplifying exponents' rules. We will also solve various examples related to the concept for a better understanding.
What is Simplifying Exponents?
Let us first recall the concept of exponents before learning to simplify exponents. The exponent of a number shows how many times the number is multiplied by itself. When we apply arithmetic operations on exponents, we use the laws of exponents for simplifying exponent expressions. Simplifying exponents is a simple process of reducing the mathematical expressions involving exponents into a simpler form such that they cannot further be simplified. Let us first go through some of the important rules for simplifying exponents in the next section.
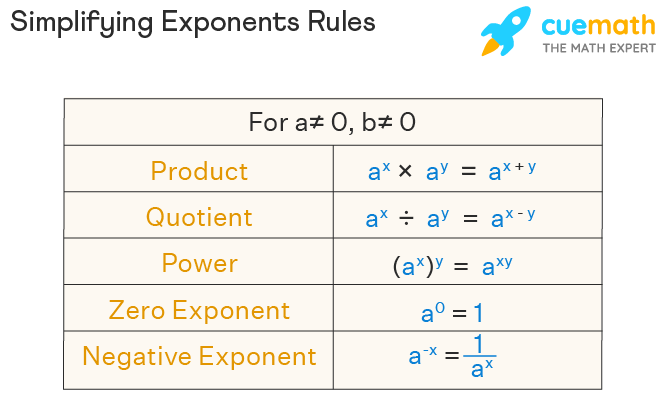
Simplifying Exponents Rules
Given below is a list of rules that we for simplifying exponents in algebraic expressions. These rules are also known as the laws of exponents and are named as per the operation involved. Let us have a look at these rules that we will use later for simplifying exponents:
- Product Rule: am × an = am+n
- Quotient Rule: am/an = am-n
- Zero Exponent Rule: a0 = 1
- Identity Exponent Rule: a1 = a
- Negative Exponents Rule: a-m = 1/am; (a/b)-m = (b/a)m
- Power of a Power Rule: (am)n = amn
- Power of a Product Rule: (ab)m = ambm
- Power of a Quotient Rule: (a/b)m = am/bm
Simplifying Exponents With Different Bases
When we multiply exponents or divide exponents with different bases, there can be two cases: i) when the exponents are the same, ii) when the exponents are different. Let us discuss each of these cases and understand the process of simplifying exponents in such cases with the help of examples.
Simplifying Exponents With Different Bases and Same Power
When simplifying exponents with different bases and the same power, we follow the rules:
- am × bm = (ab)m
- am ÷ bm = (a÷b)m
Let us simplify the following exponents: i) 24 × 34, ii) 43 ÷ 23
i) 24 × 34
= (2×3)4
= 64
ii) 43 ÷ 23
= (4÷2)3
= 23
= 8
Simplifying Exponents With Different Bases and Different Power
When we have to simplify exponents with different bases and different power, we simplify the terms separately and then apply the arithmetic operation involved. Let us solve an example to understand this better. Simplify 23 × 52. Now, here the bases and powers both are different. So, for simplifying exponents in this expression, we will simplify the terms separately first.
23 × 52
= 8 × 25
= 200
Simplifying Exponents In Fractions
In this section, we will learn how to simplify exponents in fractions. When we are given algebraic expressions in fractions, we use the laws of exponents to simplify them. Let us understand this with the help of a few examples solved below:
Example 1: Simplify (35x3y2z) / (7xy4)
Solution: We will simplify the given algebraic expression, using the simplifying exponents rules discussed above. So, we have
(35x3y2z) / (7xy4)
= (35/7) (x3/x) (y2/y4) (z)
= 5 × x3-1 × y2-4 × z
= 5x2y-2z
Answer: (35x3y2z) / (7xy4) = 5x2y-2z
Example 2: Use simplifying exponents rules to simplify (2a3b5c) × (5ab6c2).
Solution: We will combine the like terms and simplify them separately. So, we have
(2a3b5c) × (5ab6c2)
= (2×5) (a3×a) (b5×b6) (c×c2)
= 10 a3+1b5+6c1+2
= 10a4b11c3
Answer: (2a3b5c) × (5ab6c2) = 10a4b11c3
Simplifying Rational Exponents
Now that we have understood how to apply simplifying exponents rules, let us now learn to simplify rational exponents. We apply the rules in the same way for simplifying rational exponents as we did for whole numbers. Some of the common rules that we will use here are:
- ax/y × am/n = ax/y + m/n
- ax/y ÷ am/n = ax/y - m/n
- (a/b)m/n = (b/a)-m/n
- am/n = (1/a)-m/n
- (am/n)x/y = a(m/n) × (x/y)
Simplifying Negative Exponents
As we discussed in the previous section, for simplifying negative exponents, we apply the laws of exponents in the same way. Some of the common rules that we use for simplifying such exponents are:
- a-m × a-n = a(-m)+(-n)
- a-m/a-n = a-m-(-n)
- a-m = 1/am; (a/b)-m = (b/a)m
- (a-m)-n = a-m×-n
- (ab)-m = a-mb-m
- (a/b)-m = a-m/b-m
Important Notes on Simplifying Exponents
- Simplifying exponents is a simple process of reducing the mathematical expressions involving exponents into a simpler form such that they cannot further be simplified.
- When we apply arithmetic operations on exponents, we use the laws of exponents for simplifying exponent expressions.
- We can apply the rules of simplifying exponents for simplifying rational and negative exponents.
☛ Related Articles:
Simplifying Exponents Examples
-
Example 1: Simplify (4a3b6c-3) ÷ (2a4bc2)
Solution: For simplifying exponent expression (4a3b6c-3) ÷ (2a4bc2), we have
(4a3b6c-3) ÷ (2a4bc2)
= (4/2) (a3/a4) (b6/b) (c-3/c2)
= 2 a3-4 b6-1 c-3-2
= 2a-1b5c-5
= 2b5/(ac5)
Answer: (4a3b6c-3) ÷ (2a4bc2) = 2b5/(ac5)
-
Example 2: Evaluate 43 × 4-1 using simplifying exponents rules.
Solution: We will use the rule am × an = am+n to simplify the given expression. So, we have
43 × 4-1
= 43 + (-1)
= 42
= 16
Answer: 43 × 4-1 = 16
-
Example 3: Simplify [a-1/2 / b-2/3 ] 1/2
Solution: Using the rules of simplifying rational exponents, we have
[a-1/2 / b-2/3 ] 1/2
= [a-1/2]1/2 / [b-2/3]1/2
= a-1/4 / b-1/3
Answer: [a-1/2 / b-2/3 ] 1/2 = a-1/4 / b-1/3

FAQs on Simplifying Exponents
What is Simplifying Exponents in Math?
Simplifying exponents is a method of simplifying the algebraic expressions involving exponents into a simpler form such that they cannot further be simplified. There are rules in algebra for simplifying exponents with different and same bases.
What are the Rules for Simplifying Exponents?
Given below is a list of rules that we for simplifying exponents in algebraic expressions:
- Product Rule: am × an = am+n
- Quotient Rule: am/an = am-n
- Zero Exponent Rule: a0 = 1
- Identity Exponent Rule: a1 = a
- Negative Exponents Rule: a-m = 1/am; (a/b)-m = (b/a)m
- Power of a Power Rule: (am)n = amn
- Power of a Product Rule: (ab)m = ambm
- Power of a Quotient Rule: (a/b)m = am/bm
What is Simplifying Exponents in Fractions?
When algebraic expressions involving exponents are given in fractions, we simplify them using the laws of exponents. For example, (35x3y2z) / (7xy4), (5x2y7z) / (xy-1), etc.
How to Simplify Negative Exponents?
For simplifying expressions with negative exponents, we use the same laws of exponents as we use for whole numbers. Some of the commonly used rules are:
- a-m × a-n = a(-m)+(-n)
- a-m/a-n = a-m-(-n)
- a-m = 1/am; (a/b)-m = (b/a)m
- (a-m)-n = a-m×-n
- (ab)-m = a-mb-m
- (a/b)-m = a-m/b-m
How to Simplify Rational Exponents?
We apply the rules in the same way for simplifying rational exponents as we did for whole numbers. Some of the common rules that we will use here are:
- ax/y × am/n = ax/y + m/n
- ax/y ÷ am/n = ax/y - m/n
- (a/b)m/n = (b/a)-m/n
- am/n = (1/a)-m/n
- (am/n)x/y = a(m/n) × (x/y)
What is Simplifying Exponents with Different Bases?
When we multiply exponents or divide exponents with different bases, there can be two cases: i) when the exponents are the same, ii) when the exponents are different. When simplifying exponents with different bases and the same power, we follow the rule:
- am × bm = (ab)m
- am ÷ bm = (a÷b)m
When we have to simplify exponents with different bases and different power, we simplify the terms separately and then apply the arithmetic operation involved.
What is the Difference Between Simplifying Exponents and Evaluating Exponents?
Simplifying exponents means reducing an expression with an exponent to a simpler form such that it cannot further be reduced. Evaluating exponents implied determining the value of an expression by substituting the value of the variable involved or by doing the calculations involved.
visual curriculum
