Roots of Quadratic Equation
For a given quadratic equation ax2 + bx + c = 0, the values of x that satisfy the equation are known as its roots. i.e., they are the values of the variable (x) which satisfies the equation. The roots of a quadratic function are the x-coordinates of the x-intercepts of the function. Since the degree of a quadratic equation is 2, it can have a maximum of 2 roots. We can find the roots of quadratic equations using different methods.
- Factoring (when possible)
- Quadratic Formula
- Completing the Square
- Graphing (used to find only real roots)
Let us understand more about the roots of the quadratic equation along with discriminant, nature of the roots, the sum of roots, the product of roots, and more along with some examples.
Roots of Quadratic Equation
The roots of a quadratic equation are the values of the variable that satisfy the equation. They are also known as the "solutions" or "zeros" of the quadratic equation. For example, the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and x = 5 because they satisfy the equation. i.e., when each of them is substituted in the given equation we get 0.
- when x = 2, 22 - 7(2) + 10 = 4 - 14 + 10 = 0.
- when x = 5, 52 - 7(5) + 10 = 25 - 35 + 10 = 0.
But how to find the roots of a general quadratic equation ax2 + bx + c = 0? Let us try to solve it for x by completing the square.
ax2 + bx = - c
Dividing both sides by 'a',
x2 + (b/a) x = - c/a
Here, the coefficient of x is b/a. Half of it is b/(2a). Its square is b2/4a2. Adding b2/4a2 on both sides,
x2 + (b/a) x + b2/4a2 = (b2/4a2) - (c/a)
[ x + (b/2a) ]2 = (b2 - 4ac) / 4a2 (using (a + b)^2 formula)
Taking square root on both sides,
x + (b/2a) = ±√ [(b2 - 4ac) / 4a2]
x + (b/2a) = ±√ (b2 - 4ac) / 2a
Subtracting b/2a from both sides,
x = - (b/2a) ±√ (b2 - 4ac) / 2a
x = (-b ± √ (b2 - 4ac) )/2a
This is known as the quadratic formula and it can be used to find any type of roots of a quadratic equation.
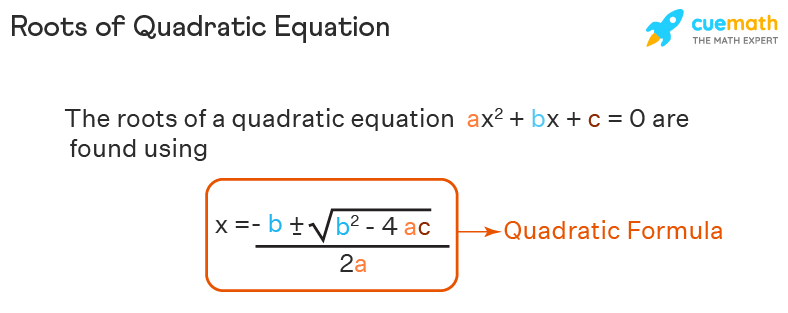
How to Find the Roots of Quadratic Equation?
The process of finding the roots of the quadratic equations is known as "solving quadratic equations". In the previous section, we have seen that the roots of a quadratic equation can be found using the quadratic formula. Along with this method, we have several other methods to find the roots of a quadratic equation. To know about these methods in detail, click here. Let us discuss each of these methods here by solving an example of finding the roots of the quadratic equation x2 - 7x + 10 = 0 (which was mentioned in the previous section) in each case. Note that, in each of these methods, the equation should be in the standard form ax2 + bx + c = 0.
Finding Roots of Quadratic Equation by Factoring
- Factor the left side part.
- Set each of these factors to zero and solve.
Example: Find the roots of the quadratic equation x2 - 7x + 10 = 0 by factoring.
Solution:
By factoring the quadratic expression, we get (x - 2) (x - 5) = 0.
Now, setting each factor to zero and solving, we get
x - 2 = 0, x - 5 = 0
x = 2, x = 5.
Finding Roots of Quadratic Equation by Quadratic Formula
- Find a, b, and c values by comparing the given equation with ax2 + bx + c = 0.
- Substitute them in the quadratic formula and simplify.
Example: Find the roots of quadratic equation x2 - 7x + 10 = 0 using quadratic formula.
Solution:
Here, a = 1, b = -7 and c = 10. Then by quadratic formula:
x = [-(-7) ± √((-7)2 - 4(1)(10))] / (2(1))
= [ 7 ± √(49 - 40) ] / 2
= [ 7 ± √(9) ] / 2
= [ 7 ± 3 ] / 2
= (7 + 3) / 2, (7 - 3) / 2
= 10/2, 4/2
= 5, 2
Therefore, x = 2, x = 5.
Finding Roots of Quadratic Equation by Completing Square
- Complete the square on the left side.
- Solve by taking square root on both sides.
Example: Find the quadratic roots of x2 - 7x + 10 = 0 by completing square.
Solution:
By completing the square, we get (x - (7/2) )2 = 9/4.
Now, taking the square root on both sides:
x - 7/2 = ± 3/2
x - 7/2 = 3/2, x - 7/2 = -3/2
x = 10/2, x = 4/2
x = 5, x = 2
Finding Roots of Quadratic Equation by Graphing
- Graph the left side part (the quadratic function) either manually or using the graphing display calculator (GDC).
- Identify the x-intercepts which are nothing but the roots of the quadratic equation.
Example: Find the roots of the quadratic equation x2 - 7x + 10 = 0 by graphing.
Solution: To solve this, we just need to graph f(x) = x2 - 7x + 10 and identify the x-intercepts.
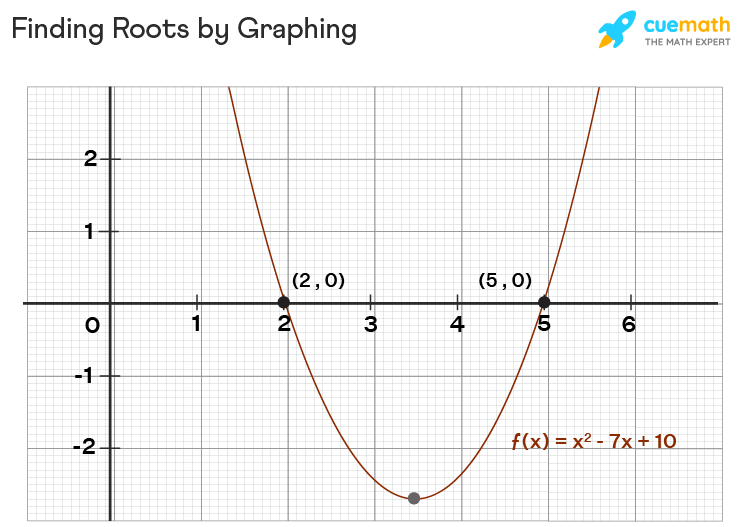
Therefore, the roots of the quadratic equation are x = 2 and x = 5.
We can observe that the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and x = 5 in each of the methods. Note that the factoring method works only when the quadratic equation is factorable; and we cannot find the complex roots of the quadratic equation using the graphing method. So the best methods that always work for finding the roots are the quadratic root formula and completing the square methods.
Nature of Roots of Quadratic Equation
The nature of the roots of a quadratic equation talks about "how many roots the equation has?" and "what type of roots the equation has?". A quadratic equation can have:
- two real and different roots
- two complex roots
- two real and equal roots (it means only one real root)
For example, in the above example, the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and x = 5, where both 2 and 5 are two different real numbers, and so we can say that the equation has two real and different roots. But for finding the nature of the roots, we don't actually need to solve the equation. We can determine the nature of the roots by using the discriminant. The discriminant of the quadratic equation ax2 + bx + c = 0 is D = b2 - 4ac.
The roots of quadratic equation formula is x = (-b ± √ (b2 - 4ac) )/2a. So this can be written as x = (-b ± √ D )/2a. Since the discriminant D is in the square root, we can determine the nature of the roots depending on whether D is positive, negative, or zero.
Nature of Roots When D > 0
Then the above formula becomes,
x = (-b ± √ positive number )/2a
and it gives us two real and different roots. Thus, the quadratic equation has two real and different roots when b2 - 4ac > 0.
Nature of Roots When D < 0
Then the above formula becomes,
x = (-b ± √ negative number )/2a
and it gives us two complex roots (which are different) as the square root of a negative number is a complex number. Thus, the quadratic equation has two complex roots when b2 - 4ac < 0.
Note: A quadratic equation can never have one complex root. The complex roots always occur in pairs. i.e., if a + bi is a root then a - bi is also a root.
Nature of Roots When D = 0
Then the above formula becomes,
x = (-b ± √ 0)/2a = -b/2a
and hence the equation has only one real root. Thus, the quadratic equation has only one real root (or two equal roots -b/2a and -b/2a) when b2 - 4ac = 0.
Sum and Product of Roots of Quadratic Equation
We have seen that the roots of the quadratic equation x2 - 7x + 10 = 0 are x = 2 and x = 5. So the sum of its roots = 2 + 5 = 7 and the product of its roots = 2 × 5 = 10. But the sum and the product of roots of a quadratic equation ax2 + bx + c = 0 can be found without actually calculating the roots. Let us see how.
We know that the roots of an equation ax2 + bx + c = 0 by quadratic formula are (-b + √ (b2 - 4ac)) /2a and (-b - √ (b2 - 4ac) )/2a. Let us represent these by x1 and x2 respectively.
Sum of Roots of Quadratic Equation
The sum of the roots = x1 + x2
= (-b + √ (b2 - 4ac)) /2a + (-b - √ (b2 - 4ac) )/2a
= -b/2a - b/2a
= -2b/2a
= -b/a
Therefore, the sum of the roots of the quadratic equation ax2 + bx + c = 0 is -b/a.
For the equation, x2 - 7x + 10 = 0, the sum of the roots = -(-7)/1 = 7 (which was the sum of the actual roots 2 and 5).
Product of Roots of Quadratic Equation
The product of the roots = x1 · x2
= (-b + √ (b² - 4ac) )/2a · (-b - √ (b² - 4ac) )/2a
= (-b/2a)2 - ( √ (b2 - 4ac)/ 2a)2 ( by a² - b² formula)
= b2 / 4a2 - (b2 - 4ac) / 4a2
= (b2 / 4a2) - (b2 / 4a2) + (4ac / 4a2)
= 4ac / 4a2
= c/a
Therefore, the product of the roots of the quadratic equation ax2 + bx + c = 0 is c/a.
For the equation, x2 - 7x + 10 = 0, the product of the roots = 10/1 = 10 (which was the product of the actual roots 2 and 5).
Important Formulas on Roots of Quadratic Equations:
For a quadratic equation ax2 + bx + c = 0,
- The roots are calculated using the formula, x = (-b ± √ (b2 - 4ac) )/2a.
- Discriminant is, D = b2 - 4ac.
If D > 0, then the equation has two real and distinct roots.
If D < 0, the equation has two complex roots.
If D = 0, the equation has only one real root. - Sum of the roots = -b/a
- Product of the roots = c/a
☛ Related Topics:
Examples on Roots of Quadratic Equation
-
Example 1: Find the roots of the quadratic equation √2 p2 + 7p + 5√2 = 0.
Solution:
Let us find the roots using the factoring method.
Comparing the given equation with ap2 + bp + c = 0, a = √2, b = 7 and c = 5√2.
Here, ac = (√2) (5√2) = 10 and b = 7.
Two numbers whose sum is 7 and whose product is 10 are 2 and 5. Let us split the middle term using these numbers.
√2 p2 + 2p + 5p + 5√2 = 0
√2 p (p + √2) + 5 (p + √2) = 0
(p + √2) (√2 p + 5) = 0
p + √2 = 0; √2 p + 5 = 0
p = -√2; p = -5/√2 (or) -5√2/2
Answer: The roots of the given quadratic equation are -√2 and -5√2 / 2.
-
Example 2: Find the value(s) of k if the quadratic equation 3x2 + kx + 2 = 0 has equal roots.
Solution:
The quadratic equation ax2 + bx + c = 0 has equal roots if its discriminant b2 - 4ac = 0.
Here, a = 3, b = k, and c = 2.
b2 - 4ac = 0
k2 - 4(3)(2) = 0
k2 - 24 = 0
k2 = 24
k = ± √24
k = ± 2√6
Answer: When the given quadratic equation has equal roots, k = 2√6 or k = -2√6.
-
Example 3: Find the sum and the product of the roots of the equation (p + 1) x2 - 2px + (q + 1) = 0 in terms p and q.
Solution:
Comparing the given equation with ax2 + bx + c = 0,
a = p + 1, b = -2p, and c = q + 1.
The sum of the roots = -b/a = -(-2p) / (p + 1) = 2p / (p + 1).
The product of the roots = c/a = (q + 1) / (p + 1).
Answer: The sum of the roots is 2p / (p + 1) and the product of the roots is (q + 1) / (p + 1).

FAQs on Roots of Quadratic Equation
What are the Roots of a Quadratic Equation?
The roots of a quadratic equation ax2 + bx + c = 0 are the values of the variable (x) that satisfy the equation. For example, the roots of the equation x2 + 5x + 6 = 0 are -2 and -3. The roots of an equation ax2 + bx + c = 0 can be found by the quadratic formula x = (-b ± √ (b2 - 4ac)) /2a.
How Can We Find the Roots of Quadratic Equation?
The roots of a quadratic equation ax2 + bx + c = 0 can be found using the quadratic formula that says x = (-b ± √ (b2 - 4ac)) /2a. Alternatively, if the quadratic expression is factorable, then we can factor it and set the factors to zero to find the roots.
What are the Three Types of Roots of Roots of Quadratic Equation?
A quadratic equation ax2 + bx + c = 0 can have:
- two real and distinct roots when b2 - 4ac > 0.
- two complex roots when b2 - 4ac < 0.
- two real and equal roots when b2 - 4ac = 0.
How to Find the Roots of Quadratic Equation by Completing Square?
To find the roots of a quadratic equation ax2 + bx + c = 0 by completing square, complete the square on the left side first. Then solve for x by taking the square root on both sides.
Where to Find Quadratic Root calculator?
The quadratic root calculator can be found by clicking here. This allows us to enter a quadratic equation and then it shows the roots along with step-by-step calculation.
How to Determine the Nature of Roots of Quadratic Equation?
The nature of the roots of an equation ax2 + bx + c = 0 is determined by its discriminant, D = b2 - 4ac.
- If D > 0, the equation has two real and distinct roots.
- If D < 0, the equation has two complex roots.
- If D = 0, the equation has two equal real roots.
How to Find the Roots of Quadratic Equation Using Quadratic Formula?
The quadratic formula says the roots of a quadratic equation ax2 + bx + c = 0 are given by x = (-b ± √ (b2 - 4ac)) /2a. To solve any quadratic equation, convert it into standard form ax2 + bx + c = 0, find the values of a, b, and c, substitute them in the roots of quadratic equation formula and simplify.
How to Find the Sum and Product of Roots of Quadratic Equation?
For any quadratic equation ax2 + bx + c = 0 whose roots are α and β,
- the sum of the roots, α + β = -b/a
- the product of the roots, α × β = c/a
Can Both the Roots of Quadratic Equation be Zeros?
Yes, both the roots of a quadratic equation can be zeros. For example, the two roots of the quadratic equation x2 = 0 are 0 and 0.
How to Find the Roots of Quadratic Equation by Factoring?
To find the roots of an equation ax2 + bx + c = 0 by factoring, factor its left side part, set each of the factors to zero and solve.
visual curriculum