Prove that Root 5 is Irrational Number
Is root 5 an irrational number? A number that can be represented in p/q form where q is not equal to 0 is known as a rational number whereas numbers that cannot be represented in p/q form are known as irrational numbers. An irrational number can also be denoted as a number that does not terminate and keeps extending after the decimal point. Now that we know about rational and irrational numbers let us take a look at the detailed discussion and prove that root 5 is irrational.
| 1. | Prove that Root 5 is Irrational Number |
| 2. | Prove That Root 5 is Irrational by Contradiction Method |
| 3. | Prove That Root 5 is Irrational by Long Division Method |
| 4. | Solved Examples |
| 5. | FAQs on Is Root 5 an Irrational? |
![]() Prove that Root 5 is Irrational Number
Prove that Root 5 is Irrational Number
Problem statement: Prove that Root 5 is Irrational Number
Given: The number 5
Proof: On calculating the value of √5, we get the value √5 = 2.23606797749979...As discussed above a decimal number that does not terminate after the decimal point is also an irrational number. The value obtained for the root of 5 does not terminate and keeps extending further after the decimal point. This satisfies the condition of √5 being an irrational number.
Hence, √5 is an irrational number.
The square root of 5 is commonly also called "root 5". The root of a number "n" is represented as √n. Thus, we define the root of a number as the number that on multiplication to itself gives the original number. For example, √5 on multiplication to itself gives the number 5. In order to prove that root 5 is an irrational number, we use different methods like the contradiction method and long division method.
Prove That Root 5 is Irrational by Contradiction Method
Given: Number 5
To Prove: Root 5 is irrational
Proof: Let us assume that square root 5 is rational. Thus we can write, √5 = p/q, where p, q are the integers, and q is not equal to 0. The integers p and q are coprime numbers thus, HCF (p,q) = 1.
√5 = p/q
⇒ p = √5 q ------- (1)
On squaring both sides we get,
⇒ p2 = 5 q2
⇒ p2/5 = q2 ------- (2)
Assuming if p was a prime number and p divides a2, then p divides a, where a is any positive integer.
Hence, 5 is a factor of p2.
This implies that 5 is a factor of p.
Thus we can write p = 5a (where a is a constant)
Substituting p = 5a in (2), we get
(5a)2/5 = q2
⇒ 25a2/5 = q2
⇒ 5a2 = q2
⇒ a2 = q2/5 ------- (3)
Hence 5 is a factor of q (from 3)
(2) indicates that 5 is a factor of p and (3) indicates that 5 is a factor of q. This contradicts our assumption that √5 = p/q.
Therefore, the square root of 5 is irrational.
Prove That Root 5 is Irrational by Long Division Method
The value of the root 5 can be obtained by the long division method using the following steps:
- Step 1: First we write 5 as 5 00 00 00 and pair digits starting from one's place.
- Step 2: Now find a number whose square results in a number less than 5.
- Step 3: The number obtained is 2. The quotient becomes 2 and the remainder obtained is 1.
- Step 4: Now the 00 is brought down and the quotient obtained in step 3 is doubled, i.e; (2×2 = 4).
- Step 5: The unit digit with 4 should be 2 as 42×2 = 84 as it is less than 100. On subtracting 84 from 100 we get 16.
- Step 6: 00 is brought down and the quotient obtained in step 5 is doubled. Thus, 22 is doubled and 44 is obtained as the starting of a new divisor.
- Step 7: The unit digit with 44 should be 3 as 443×3 = 1329 as it is less than 1600. On subtracting 1329 from 1600 we get 271.
- Step 8: Now bring the 00 down and the quotient obtained in step 7 is doubled. Thus, 223 is doubled and 446 is obtained as the starting of a new divisor.
- Step 9: The unit digit with 446 should be 6 as 4466×6 = 26796 as it is less than 27100. On subtracting 26796 from 27100 we get 304.
- Step 10: The long division is continued further in order to obtain the required number of digits after the decimal.
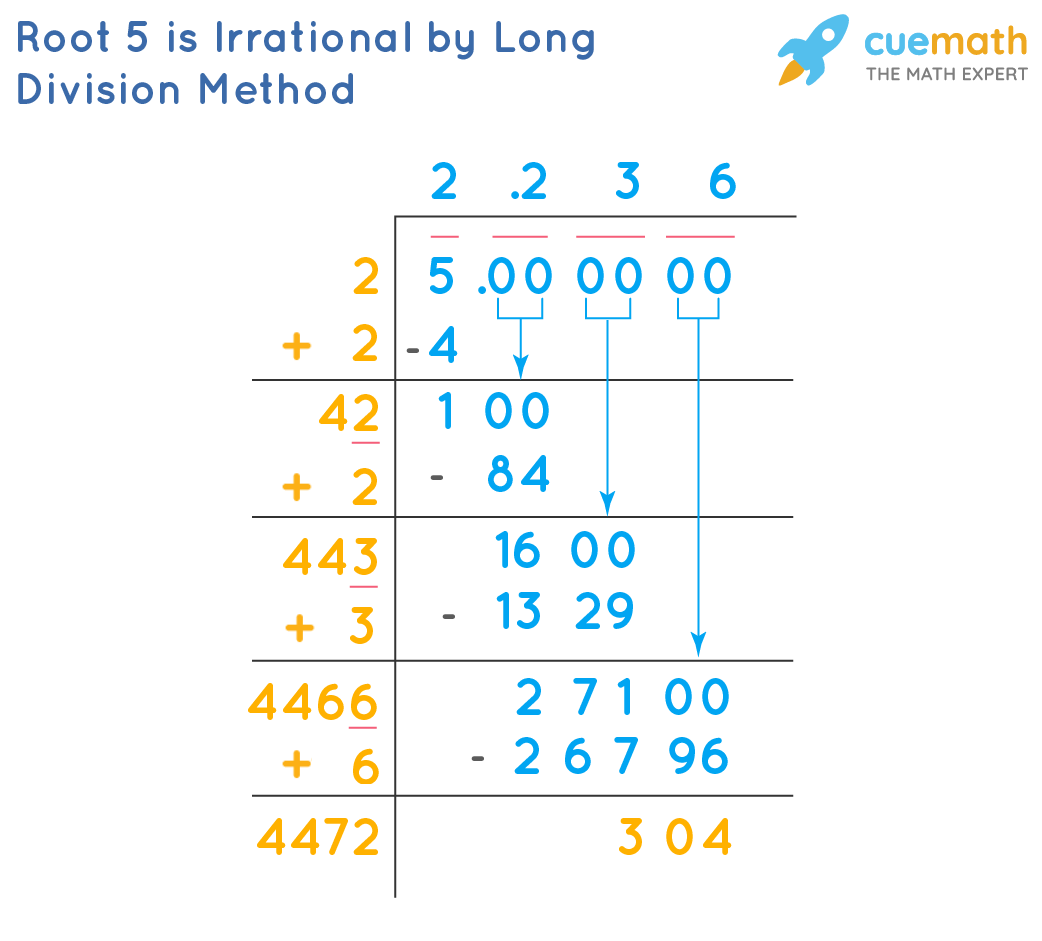
As we can see the value of root 5 does not terminate after 3 decimal places. It can still be extended further. Hence, this proves √5 an irrational number.
☛ Also Check:
Solved Examples
-
Example 1: Ashley wants to prove that √45 is an irrational number. Can you use the fact that the square root of 5 is irrational to prove it?
Solution: As we know, 45 can be expressed as 45 = 5 × 3 × 3.Taking square root on both sides we get,
√75 = √(5 × 3 × 3)
⇒ √75 = 3√5 = 3 × 2.23606797749979 = 6.708203932499369
Since we cannot simplify √5 any further, we can prove that √45 is an irrational number. -
Example 2: Danny said to his friend John that the square root of 5 is an irrational number. She then asked her to find √5 using a number line. Can you help her?
Solution:
Following are the steps to construct the root 5 on the number line.
- Step 1: Draw OA = 2 units on the number line, and by drawing a perpendicular line AB = 1 unit, join OB.
- Step 2: We can conclude that the line segment OB will measure √5 by using the Pythagorean Theorem as √(OA2 + AB2) = √(22 + 12) = √5 units.
- Step 3: Now we construct OP on the number line which measures the same as OB by considering OB as radius.
- Step 4: We can conclude that OP and OB have the same length which is equal to √5 units.
Thus, √5 is represented on the number line.

FAQs on Is Root 5 an Irrational
How do you Prove that Root 5 is Irrational?
Root 5 cannot be expressed in p/q form where p, q are integers and q is not equal to 0. On determining the square root of 5 we can find that it gives a value √5 = 2.23606797749979... which does not terminate after the decimal but only extends further. There are two ways to prove that root 5 is irrational, one by using the method of contradiction and the other by using the method of long division.
Is 2 times the square root of 5 Irrational?
Yes, 2 times the square root of 5 is an irrational number as 2 × √5 = 2 × 2.23606797749979 = 4.472135954999579...A rational number multiplied with an irrational number is irrational. 2 is a rational number which is multiplied with root 5 which is irrational. Thus, 2 times the square root of 5 is irrational.
How to Prove Root 5 is Irrational by Contradiction?
In order to prove root 5 is irrational using contradiction we use the following steps:
- Step 1: Assume that √5 is rational.
- Step 2: Write √5 = p/q
- Step 3: Now both sides are squared, simplified and a constant value is substituted.
- Step 4: It is found that 5 is a factor of the numerator and the denominator which contradicts the property of a rational number.
Is 3 Times the Square Root of 5 Irrational?
Yes, 3 times the square root of 5 is an irrational number as it can be written as 3 × √5 = 3 × 2.23606797749979 = 6.708203932499369... A rational number multiplied with an irrational number is root 5. We can see that any rational number multiplied with root 5 will be irrational. Thus, 3 times the square root of 5 is irrational too.
How to Prove that 1 by Root 5 is irrational?
When we rationalize 1/√5, we get 1/√5 × √5/√5 = √5/5. As we know, that √5 is an irrational number, and dividing an irrational number by a rational number also gives an irrational number, thus we can conclude that 1/√5 is irrational.
visual curriculum
