Properties of Log

The properties of log are used to expand a single logarithm into multiple logarithms (or) compress multiple logarithms into a single logarithm. A logarithm is just another way of writing exponents. Thus, the properties of logarithms are derived from the properties of exponents.

Let us learn the properties of log along with their proofs and let us solve a few examples also using these properties.

The properties of log are nothing but the rules of logarithms and these are derived from the exponent rules. These properties of logarithms are used to solve the logarithmic equations and to simplify logarithmic expressions. There are 4 important logarithmic properties which are listed below:

• logₐ mn = logₐ m + logₐ n (product property)
• logₐ m/n = logₐ m - logₐ n (quotient property)
• logₐ mⁿ = n logₐ m (power property)
• log_b a = (log꜀ a) / (log꜀ b) (change of base property)

Apart from these, we have several other properties of logarithms which are directly derived from the exponent rules and the definition of the logarithm (which is a^x = m ⇔ logₐ m = x).

• a⁰ = 1 ⇒ logₐ 1 = 0
• a¹ = a ⇒ logₐ a = 1
• a^{logₐ x} = x
• \(\log _{b^{n}} a^{m}=\frac{m}{n} \log _{b} a\)
  (follows from the change of base rule and power property)

All the properties of log are mentioned below.

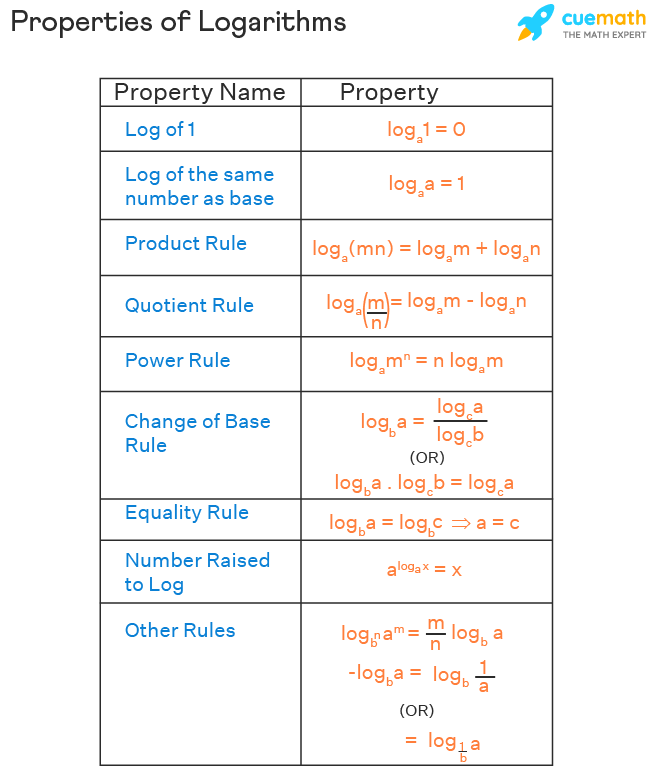
We will study each property one by one in detail along with their derivations in the upcoming sections.

The natural log is nothing but the logarithm with base "e". i.e., logₑ = ln. All the above properties are mentioned in terms of "log" and are applicable for any base and hence all the above properties are applicable for the natural log as well. Here are the natural logarithmic properties.

• ln 1 = 0
• ln e = 1
• ln (mn) = ln m + ln n
• ln (m/n) = ln m - ln n
• ln mⁿ = n ln m
• e^{ln x} = x

The product property of logarithms is used to express the logarithm of a product as the sum of logs. Let us derive the product property: logₐ mn = logₐ m + logₐ n.

Derivation:

Let logₐ m = x and logₐ n = y. By converting each of these into exponential forms, we get

logₐ m = x ⇒ m = a^x ... (1)

logₐ n = y ⇒ n = a^y ... (2)

Multiplying the equations (1) and (2),

mn = a^x · a^y

By using the rule of multiplying exponents,

mn = a^{x + y}

Converting this back to logarithmic form,

logₐ mn = x + y

Substituting the values of x and y back here,

logₐ mn = logₐ m + logₐ n

Hence, the product property of log is derived. Here are a few examples of the application of this property.

• log (2x) = log 2 + log x
• log 6 = log (2×3) = log 2 + log 3
• log₂ (xy) = log₂ x + log₂ y

Note that the product property of log does not give any information for finding log (m + n). In fact, there is no other property available for finding log (m + n).

The quotient property of logarithms is used to express the logarithm of a quotient as the difference of logs. Let us derive the quotient property: logₐ m/n = logₐ m - logₐ n.

Derivation:

Let logₐ m = x and logₐ n = y. Let us convert these into exponential forms.

• logₐ m = x ⇒ m = a^x ... (1)
• logₐ n = y ⇒ n = a^y ... (2)

Dividing the equations (1) and (2),

m/n = a^x / a^y

By using the rule of dividing exponents,

m/n = a^{x - y}

Converting this back to logarithmic form,

logₐ m/n = x - y

Substituting the values of x and y back here,

logₐ m/n = logₐ m - logₐ n

Hence, the product property of log is derived. Here are a few examples of the application of this property.

• log (2/x) = log 2 - log x
• log 10 = log (20/2) = log 20 - log 2
• log₅ (x/y) = log₅ x - log₅ y

Note that the quotient property of log is NOT applicable for finding log (m - n) (because it is applied when it is of the form the log m/n (or) log m - log n).

The power property of logarithm says logₐ mⁿ = n logₐ m. It means the exponent of the argument can be pulled to in front of the log.

Derivation:

Let logₐ m = x. Converting this into exponential form,

a^x = m

Let us raise both sides by n. Then

(a^x) ⁿ = mⁿ

By the power property of exponents,

a^nx = mⁿ

Converting this into logarithmic form,

logₐ mⁿ = nx

Substituting x = logₐ m back here,

logₐ mⁿ = n logₐ m

Hence, the power property of log is derived. Here are a few examples of this property.

• log 2^x = x log 2
• log x³ = 3 log x
• log₅ x^y = y log₅ x

The change of base property says log_b a = (log꜀ a) / (log꜀ b). It means that log_b a can be written as the quotient of two logarithms (log a)/(log b) where both logs should be of the same base (say c). We know that we have two buttons on the calculator to evaluate logarithms. One is log (with base 10) and the other is ln (with base 'e'). But what if we have to calculate a logarithm with some other base, say log₅ 2? This property is very helpful in calculating such logarithms. If we apply the change of base property to log₅ 2, we get

log₅ 2 = (log 2) / (log 5)
≈ (0.3010) / (0.6990)
≈ 0.4306

Let us derive the change of base rule now.

Derivation:

Let log_b a = x, log꜀ a = y, and log꜀ b = z. Let us convert these equations into logarithmic form.

• log_b a = x ⇒ a = b^x ... (1)
• log꜀ a = y ⇒ a = c^y ... (2)
• log꜀ b = z ⇒ b = c^z ... (3)

From (1) and (2),

b^x = c^y

Substituting b = c^z (from (3)),

(c^z)^x = c^y

c^zx = c^y

zx = y (or) x = y/z

Substituting the values of x, y, and z here:

log_b a = (log꜀ a) / (log꜀ b)

Hence, the change of base property of log is derived. By multiplying it on both sides by log꜀ b, we get another form of change of base rule.

log_b a · log꜀ b = log꜀ a

Here are the examples of both forms of the property:

• log₄ 3 = (log 3)/(log 4)
• log₄ 2 · log₅ 4 = log₅ 2

Important Notes on Logarithmic Properties

• The logarithmic properties are applicable for a log with any base. i.e., they are applicable for log, ln, (or) for logₐ.
• The 3 important properties of logarithms are:
  log mn = log m + log n
  log (m/n) = log m - log n
  log mⁿ = n log m
• log 1 = 0 irrespective of the base.
• Logarithmic properties are used to expand or compress logarithms.

☛ Related Topics:

• Log Calculator
• Natural Log Calculator
• Log Table
• Antilog Table

What are 4 Logarithmic Properties?

The logarithmic properties are used to compress/expand logarithms. There are 4 important logarithmic properties:

• log xy = log x + log y
• log x/y = log x - log y
• log a^m = m log a
• log_ba = (log a)/(log b)

What are the Applications of Properties of Log?

We can use the properties of log in simplifying the logarithmic functions and expand/compress the logarithms. For example, using the property log (mn) = log m + log n we can write

• either log 6 as log 2 + log 3
• or log 2 + log 3 as log 6

What is a Number Raised to Log Property?

The result of a number raised to a logarithm of the same base is equal to the argument of the logarithm i.e., a^{logₐ x} = x.

What is Negative Log Property?

We can use the power property of logarithms to convert a negative log into a positive log. For example:

-log_b a = log_b a^-1 = log_b (1/a)

We can apply the change of base rule and power property together to convert a negative log into a positive log.

-log_b a = - (log a)/(log b) = (log a) / (-log b) = (log a) / (log b^-1) = log _1/b a.

Thus, -log_b a = log_b (1/a) (or) log _1/b a.

What are All Properties of Logarithms?

There are 7 important properties of logarithms:

• log 1 = 0
• logₐ a = 1
• log ab = log a + log b
• log a/b = log a - log b
• log a^m = m log a
• log_ba = (log a)/(log b)
• a^{logₐ x} = x.

Which Property of Logarithm is Used to Change the Base?

The change of base rule is used to change the base of a logarithm. Using this rule, log_ba = (log a)/(log b), where the base of each log of right side should be the same number always.

What are Natural Logarithmic Properties?

All properties of log are applicable for natural log as well. Thus, the important properties of the natural log are:

• ln 1 = 0
• ln e = 1
• ln ab = ln a + ln b
• ln a/b = ln a - ln b
• ln a^m = m ln a
• e^{ln x} = x.