Partial Fraction
Partial Fractions are the fractions that are formed when a complex rational expression is split into two or more simpler fractions. Generally, fractions with algebraic expressions are difficult to solve and hence we use the concepts of partial fractions to split the fractions into numerous subfractions. While decomposition, generally, the denominator is an algebraic expression, and this expression is factorized to facilitate the process of generating partial fractions. A partial fraction is a reverse of the process of the addition of rational expressions.
In the normal process, we perform arithmetic operations across algebraic fractions to obtain a single rational expression. This rational expression, on splitting in the reverse direction involved the process of decomposition of partial fractions and results in the two partial fractions. Let us learn more about partial fractions in the following sections.
| 1. | What are Partial Fractions? |
| 2. | Partial Fractions Formulas |
| 3. | Partial Fraction Decomposition |
| 4. | Partial Fractions of Improper Fraction |
| 5. | FAQs on Partial Fractions |
What are Partial Fractions?
When a rational expression is split into the sum of two or more rational expressions, the rational expressions that are a part of the sum are called partial fractions. This is referred to as splitting the given algebraic fraction into partial fractions. The denominator of the given algebraic expression has to be factorized to obtain the set of partial fractions.
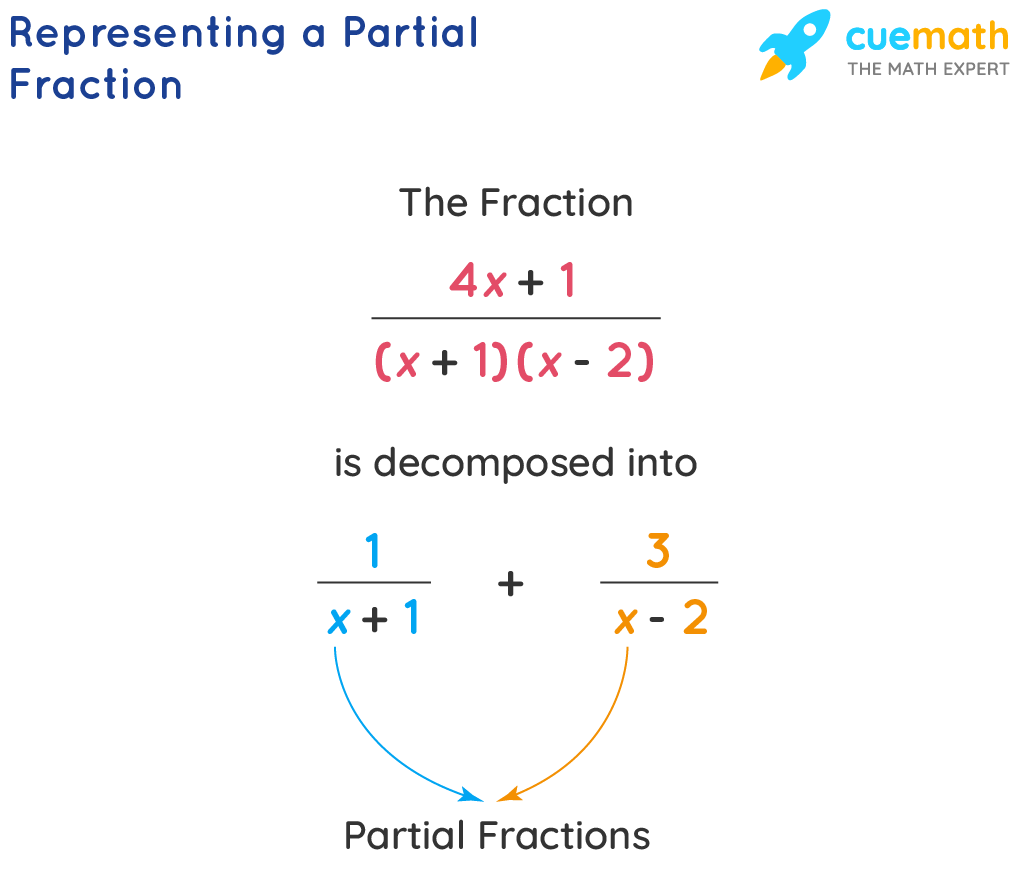
Every factor of the denominator of a rational expression corresponds to a partial fraction. For example, in the above figure, (4x + 1)/[(x + 1)(x - 2)] has two factors in the denominator, and hence there are two partial fractions, one with the denominator (x + 1) and the other with the denominator (x - 2).
Partial Fractions Formulas
In the above example, the numerators of partial fractions are 1 and 3. The numerator of a partial fraction is not always a constant. If the denominator is a linear function, the numerator is constant. And, if the denominator is a quadratic equation, then the numerator is linear. It means, the numerator's degree of a partial fraction is always one less than the denominator's degree. Further, the rational expression needs to be a proper fraction to be decomposed into a partial fraction. Listed below in the table are partial fraction formulas (here, all variables apart from x are constants).
| Type | Form of Rational Fraction | Partial Fraction Decomposition |
|---|---|---|
| Non-repeated Linear Factor | (px + q)/(ax + b) | A/(ax + b) |
| Repeated Linear Factor | (px + q)/(ax + b)n | A1/(ax + b) + A2/(ax + b)2 + .......... An/(ax + b)n |
| Non-repeated Quadratic Factor | (px2 + qx + r)/(ax2 + bx + c) | (Ax + B)/(ax2 + bx + c) |
| Repeated Quadratic Factor | (px2 + qx + r)/(ax2 + bx + c)n | (A1x + B1)/(ax2 + bx + c) + (A2x + B2)/(ax2 + bx + c)2 + ...(Anx + Bn)/(ax2 + bx + c)n |
Let us look at a few examples of partial fractions.
- 4/[(x - 1)(x + 5)] = [A/(x - 1)] + [B/(x + 5)]
- (3x + 1)/[(2x - 1)(x + 2)2] = [A/(2x - 1)] + [B/(x + 2)] + [C/(x + 2)2]
- (2x - 3)/[(x - 2)(x2 + 1)] = [A/(x - 2)] + [(Bx + C)/(x2 + 1)]
In all these examples, A, B, and C are constants to be determined. Let's learn how to find these constants.
Partial Fraction Decomposition
The partial fraction decomposition is writing a rational expression as the sum of two or more partial fractions. The following steps are helpful to understand the process to decompose a fraction into partial fractions.
- Step-1: Factorize the numerator and denominator and simplify the rational expression, before doing partial fraction decomposition.
- Step-2: Split the rational expression as per the formula for partial fractions. P/((ax + b)2 = [A/(ax + b)] + [B/(ax + b)2]. There are different partial fractions formulas based on the numerator and denominator expression.
- Step-3: Take the LCM of the factors of the denominators of the partial fractions, and multiply both sides of the equation with this LCM.
- Step-4: Simplify and obtain the values of A and B by comparing coefficients of like terms on both sides.
- Step-5: Substitute the values of the constants A and B on the right side of the equation to obtain the partial fraction.
Let us learn this process of decomposing a given fraction into partial fractions by an example.
Example: Find the partial fraction expansion of the expression(4x + 12)/(x2 + 4x)
Solution:
Always remember to factor the denominator as much as possible before doing the partial fraction decomposition. (4x + 12)/(x2 + 4x) = (4x + 12)/[x(x + 4)] ; The denominator has non-repeated linear factors. So, every factor corresponds to a constant in the numerator while writing the partial fractions.
Let us assume that: (4x + 12)/[(x)(x + 4)] = [A/x] + [B/(x + 4)] → (1)
The LCD (Least Common Denominator) of the sum (on the right side) is x(x + 4). Multiplying both sides by x(x + 4), 4x + 12 = A(x + 4) + Bx → (2)
Now we have to solve it for A and B. For that, we set each linear factor to zero.
Substitute x + 4 = 0 , or x = -4 in (2): 4(-4) + 12 = A(0) + B(-4); -4 = -4B; B = 1.
Substitute x = 0 in (2): 4(0) + 12 = A(0 + 4) + B(0); 12 = 4A; A = 3.
Substitute the values of A and B in (1), we get the partial fractions decomposition of the given expression: (4x + 12)/[x(x + 4)] = [3/x] + [1/(x + 4)]
Tips & Tricks on Partial Fractions Decomposition
The following tips are helpful to decompose a fraction into its partial fractions.
- If the denominator has non-repeated linear factors:
The constants can be obtained by setting each linear factor to zero. - If the denominator has either repeated linear factors and/or irreducible quadratic factors:
Set the linear factors to zero to find the value of some constants.
Set x = 0 to get at least one another constant.
Compare the coefficients of x3, x2, ..., etc to find the other constants.
Partial Fractions of Improper Fraction
When we have to decompose an improper fraction into partial fractions, we first should do the long division. The long division is helpful to give a whole number and a proper fraction. The whole number is the quotient in the long division, and the remainder forms the numerator of the proper fraction, and the denominator is the divisor. The format of the result of the long division would be Quotient + Remainder/Divisor. Let us understand more of this with the help of the below example.
Example: Find the partial fraction decomposition of the expression (x3 +4x2 - 2x - 5)/(x2 - 4x + 4)
Solution: Here, the degree of the numerator (3) is greater than the degree of the denominator (2). So the given fraction is improper. So we have to do the long division first.
Then write the given fraction as Quotient + Remainder/Divisor. Then we get: (x3 + 4x2 - 2x - 5)/(x2 - 4x + 4) = x + 8 + (26x - 37)/(x2- 4x + 4).
Here, the fraction on the right side is a proper fraction and hence it can be split into partial fractions. (26x - 37)/(x2 - 4x + 4) = (26x - 37)/(x - 2)2 = A/(x - 2)+ B/(x - 2)2
Now let us try to solve for A and B. Hint: Set each of (x - 2) and x one by one to zero to get A and B. You should get A = 26 and B = 15.
Substituting these values in we have: (26x-37)/(x2 - 4x + 4) = [26/(x-2)] + [15/(x-2)2] Further we have: (x3+ 4x2 - 2x - 5)/(x2- 4x + 4) = x + 8 + [26/(x - 2)] + [15/(x - 2)2 ]
Important Notes on Partial Fractions
The following points would help in gaining a more clear understanding of partial fractions.
- The numerator's degree of a partial fraction is always just 1 less than the denominator's degree.
- When a partial fraction has repeated factors of the form (ax + b)n or (ax2 + bx + c)n, they correspond to n different partial fractions where the denominators of the partial fractions have exponents 1, 2, 3, ..., n.
- The above partial fractions formulas do not depend upon the numerator of the given rational expression.
- Before applying the above formulas, factorize the denominator as much as possible. Otherwise, the answer won't be accurate
☛ Related Topics:
Solved Examples on Partial Fraction
-
Example 2: Decompose the following expression into partial fractions.
(x4 + x3 + x2 + 1)/(x2 + x - 2)
Solution:
When we factorize the denominator, we get: x2 + x - 2 = (x + 2)(x - 1). The degree of the numerator (4) is greater than that of the denominator (2). So it is an improper fraction. We need to first do the long division.
So the given fraction can be written as: (x4 + x3 + x2 + 1)/(x2 + x - 2) = x2 + 3 + (-3x + 7)/[(x + 2)(x - 1)];
Now we will decompose (-3x + 7)/[(x + 2)(x - 1)] into partial fractions using:
(-3x + 7)/[(x + 2)(x - 1)] = A/(x + 2) + B/(x - 1) ... (1)
Multiplying both sides by the LCD (x + 2)(x - 1); -3x + 7 = A(x - 1) + B(x + 2).
Substitute x - 1 = 0, or x = 1 we have -3 + 7 = 3B ;B = 4/3.
Substitute x + 2 = 0, or x = -2 we have 6 + 7 = -3A ; A= -13/3.
Substitute the values of A and B in the equation (1) we have:(-3x + 7)/[(x + 2)(x - 1)] = -13/[3(x + 2)]+ 4/[3(x - 1)].
Answer: Therefore the partial fractions decomposition of the given expression is: x2 + 3 - 13/[3(x + 2)]+ 4/[3(x - 1)]
-
Example 3: Decompose the following rational expression into partial fractions.
(4x3 + x + 2)/[x2(x2 + 1)]
Solution:
Look at the denominator. We have x2. It means the linear factor x is repeating. (x2 + 1) is an irreducible (can't be factorized) quadratic factor. So the given fraction can be decomposed as follows:
(4x3 + x + 2)/[x2(x2 + 1)] = [A/x] + [B/x2] + [(Cx + D)/(x2 + 1)] ... (1)
Multiplying both sides by the LCD x2(x2 + 1); 4x3 + x + 2 = Ax3 + Ax + Bx2 + B + Cx3 + Dx2 Setting the linear factor x to 0, i.e., x = 0, we get: 2 = B. Now we do not have any other linear factors to set to zero. So we will expand the right-hand side expression. Then we will compare the coefficients of x3, x2, x, and constant.
By comparing the coefficients of x3, we get 4 = A + C.
By comparing the coefficients of x2, we get 0 = B + D.
By comparing the coefficients of x, we get 1 = A. By comparing the constants, we get 2 = B.
By solving these equations, we get: A = 1, B = 2, C = 3, D = -2 . Further, substitute all these values in (1), the given expression becomes: [1/x] + [2/x2] + [(3x - 2)/(x2 + 1)]
Answer: Therefore we have the resultant partial fractions as (4x3 + x + 2)/[x2(x2 + 1)] = [1/x] + [2/x2] + [(3x - 2)/(x2 + 1)].
FAQs on Partial Fractions
What is the Partial Fraction Method?
The partial fraction is the result of writing a rational expression as the sum of two or more fractions. First simplify the rational expression by breaking it down into the possible factors for the numerator, and the denominator. Further, split the expression into partial fractions based on the formulas. The formulas for partial fractions depend on the number of factors and the degree of the denominator of the rational expression. Further, find the value of the required constants to solve the partial fractions.
What is the Procedure for Partial Fraction Decomposition?
The decomposition of partial fractions is across the following three simple steps.
- Step-1: Split the fractions as per the formula for decomposition of partial fractions, and based on the number of denominator terms.
- Step-2: Find the LCM of the denominators, and multiply both sides with the LCM.
- Step-3: Substitute appropriate values to find the values of the constants in the numerators of the partial fractions.
What Is Meant by A Partial Fraction?
The word "partial" means a "part" and hence a partial fraction is one of the fractions when a given fraction is decomposed into the sum of multiple fractions. The input for the process of the partial fractions is a rational expression, and the result is the sum of two or more proper fractions.
What Are the Different Denominator Types In the Partial Fractions?
The different denominator types in partial fractions are based on the number of factors of the denominator expression, and the degree of the terms in the denominator. The different denominator types of a partial P/(ax + b), P/[(ax + b)(cx + d)], P/(ax + b)2, P/(ax + b)3, P/(ax + b)n.
How Do you Know How To Add Partial Fractions?
While writing an expression as the sum of partial fractions, keep the following points in mind:
- The numerator's degree of a partial fraction is always just 1 less than the denominator's degree.
- When a partial fraction has repeated factors of the form (ax+b)n (or) (ax2 +bx+c)n, they correspond to n different partial fractions where the denominators of the partial fractions have exponents 1, 2, 3, ..., n.
For more information, go to "What are General Formulas of Partial Fractions?" section of this page. To add to partial fractions, we just make their denominators the same and add.
For example: 3/x + 1/(x + 4) = 3/x · (x + 4)/(x + 4) + 1/(x + 4) · x/x = (3x + 12)/(x2 + 4x) + x/(x2 + 4x) = (3x + 12 + x)/(x2 + 4x)= (4x + 12)/(x2 + 4x)
How Do you Solve a Repeated Root Partial Fraction?
When a partial fraction has repeated factors of the form (ax+b)n or (ax2+bx+c)n, they correspond to n different partial fractions where the denominators of the partial fractions have exponents 1, 2, 3, ..., n. For example, if the denominator is of the form (ax+b)n, then the corresponding partial fractions should be of the form A1/(ax + b) + A2/(ax + b)2 + .......... An/(ax + b)n.
How Do you Know When to Use Partial Fraction Decomposition?
The partial fraction decomposition is to be used when the denominator of the fraction is an algebraic expression, and when there is a need to split the fraction. Also, there should be a possibility of getting at least two factors for the algebraic expression in the denominator.
What are Formulas for Solving Different Types of Partial Fractions are there?
The types of partial fractions depend on the number of possible factors of the denominator, and the degree of the factors of the denominator. Broadly there are about three types of partial fractions. The following three types of partial fractions are as follows.
- (px + q)/[(ax + b)(cx + d)] = A/(ax + b) + B/(cx + d)
- (px + q)/[(ax + b)2 = A/(ax + b) + B/(ax + b)2
- (px2 + qx + r)/[(x + a)(x2 + bx + c)] = A/(x + a) + (Bx + c)/(x2 + bx + c)
Is Partial Fraction a Proper Fraction?
For the process of getting partial fractions, the given fraction needs to be a proper fraction. If the given fraction is an improper fraction, the numerator is divided by the denominator to obtain a quotient and a remainder. And the fraction that is used to split into partial fractions in this case would be the remainder/denominator.
How Do you Decompose Partial Fraction with 3 Terms?
Decomposing a partial fraction with 3 terms is the same as the solving of partial fractions with 2 terms. Further, the two formulas for partial fractions with 3 terms are as follows.
- k/[(x + a)(x + b)(x + c)] = A/(x + a) + B/(x + b) + C/(x + c)
- K/[x(x + a)2] = A/x + B/(x + a) + C/(x + a)2