Exponential Equations

Exponential equations, as the name suggests, involve exponents. We know that the exponent of a number (base) indicates the number of times the number (base) is multiplied. But, what happens if the power of a number is a variable? When the power is a variable and if it is a part of an equation, then it is called an exponential equation. We may need to use the connection between the exponents and logarithms to solve the exponential equations.

Let us learn the definition of exponential equations along with the process of solving them when the bases are the same and when the bases are not the same along with a few solved examples and practice questions.

An exponential equation is an equation with exponents where the exponent (or) a part of the exponent is a variable. For example, 3^x = 81, 5^{x - 3} = 625, 6^{2y - 7} = 121, etc are some examples of exponential equations. We may come across the use of exponential equations when we are solving the problems of algebra, compound interest, exponential growth, exponential decay, etc.

Types of Exponential Equations

There are three types of exponential equations. They are as follows:

• Equations with the same bases on both sides. (Example: 4^x = 4²)
• Equations with different bases that can be made the same. (Example: 4^x = 16 which can be written as 4^x = 4²)
• Equations with different bases that cannot be made the same. (Example: 4^x = 15)

The equations in algebra involving variable exponents are called equations with exponents or exponential equations. In other words, we can say that algebraic equations in which variables occur as exponents are known as the equations with exponents. Some of the examples of such an equation are, 3^{x + 4} = 81, -2^3y-7 = -64, etc.  

While solving an exponential equation, the bases on both sides may be the same or may not be the same. Here are the formulas that are used in each of these cases, which we will learn in detail in the upcoming sections.

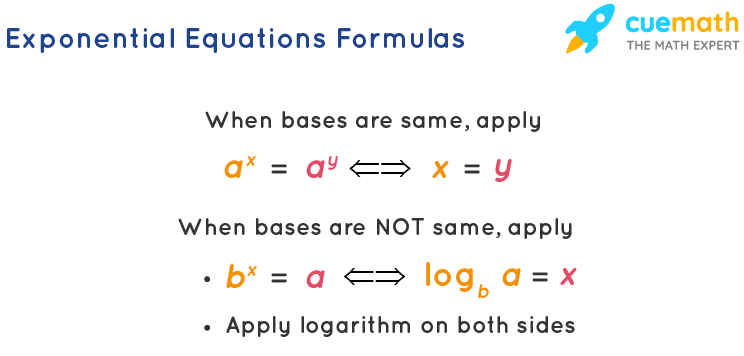
Property of Equality for Exponential Equations

This property is useful to solve an exponential equation with the same bases. It says when the bases on both sides of an exponential equation are equal, then the exponents must also be equal. i.e.,

a^x = a^y ⇔ x = y.

Exponential Equations to Logarithmic Form

We know that logarithms are nothing but exponents and vice versa. Hence an exponential equation can be converted into a logarithmic function. This helps in the process of solving an exponential equation with different bases. Here is the formula to convert exponential equations into logarithmic equations.

b^x = a ⇔ log_ba = x

Sometimes, an exponential equation may have the same bases on both sides of the equation. For example, 5^x = 5³ has the same base 5 on both sides. Sometimes, though the exponents on both sides are not the same, they can be made the same. For example, 5^x = 125. Though it doesn't have the same bases on both sides of the equation, they can be made the same by writing it as 5^x = 5³ (as 125 = 5³). To solve the exponential equations in each of these cases, we just apply the property of equality of exponential equations, using which, we set the exponents to be the same and solve for the variable.

Here is another example where the bases are not the same but can be made the same.

Example: Solve the exponential equation 7^{y + 1} = 343^y.

Solution:

We know that 343 = 7³. Using this, the given equation can be written as,

7^{y + 1} = (7³)^y

7^{y + 1} = 7^3y

Now the bases on both sides are the same. So we can set the exponents to be the same.

y + 1 = 3y

Subtracting y from both sides,

2y = 1

Dividing both sides by 2,

y = 1/2

Sometimes, the bases on both sides of an exponential equation may not be the same (or) cannot be made the same. We solve the exponential equations using logarithms when the bases are not the same on both sides of the equation. For example, 5^x = 3 neither has the same bases on both sides nor the bases can be made the same. In such cases, we can do one of the following things.

• Convert the exponential equation into the logarithmic form using the formula b^x = a ⇔ log_ba = x and solve for the variable.
• Apply logarithm (log) on both sides of the equation and solve for the variable. In this case, we will have to use a property of logarithm, log a^m = m log a.

We will solve the equation 5^x = 3 in each of these methods.

Method 1:

We will convert 5^x = 3 into logarithmic form. Then we get,

log₅3 = x

Using the change of base property,

x = (log 3) / (log 5)

Method 2:

We will apply log on both sides of 5^x = 3. Then we get, log 5^x = log 3. Using the property log a^m = m log a on the left side of the equation, we get, x log 5 = log 3. Dividing both sides by log 5,

x = (log 3) / (log 5)

Important Notes on Exponential Equations:

Here are some important notes with respect to the exponential equations.

• To solve the exponential equations of the same bases, just set the exponents equal.
• To solve the exponential equations of different bases, apply logarithm on both sides.
• The exponential equations with the same bases also can be solved using logarithms.
• If an exponential equation has 1 on any one side, then we can write it as 1 = a⁰, for any 'a'. For example, to solve 5^x = 1, we can write it as 5^x = 5⁰, then we get x = 0.
• To solve an exponential equation using logarithms, we can either apply "log" or apply "ln" on both sides.

Related Articles:

• Exponential Form
• Exponent Rules
• Exponential Functions
• Exponential Equation Calculator

What Are Exponential Equations?

An exponential equation is an equation that has a variable in its exponent(s). For example, 5^{2x - 3} = 125, 3^{7 - 2x} = 91, etc are exponential equations.

What Are Types of Exponential Equations?

There are three types of exponential equations. They are,

• The exponential equations with the same bases on both sides.
• The exponential equations with different bases on both sides that can be made the same.
• The exponential equations with different bases on both sides that cannot be made the same.

How To Solve Exponential Equations?

To solve the exponential equations of equal bases, we set the exponents equal whereas to solve the exponential equations of different bases, we apply logarithms on both sides.

How To Write Exponential Equation in Logarithmic Form?

Writing the exponential equation in the logarithmic form helps us to solve it. This can be done using the formula b^x = a ⇔ log_ba = x.

What Is the Property of Equality of Exponential Equations?

The equality property of exponential equations says to set the exponents equal whenever the bases on both sides of the equation are equal. i.e., a^x = a^y ⇔ x = y.

How To Solve Exponential Equations of Same bases?

When an exponential equation has the same bases on both sides, just set the exponents equal and solve for the variable. Here is an example, 4^{2x - 1} = 4^{1 - x}. Here the bases on both sides are equal. So we can set the exponents equal.
2x - 1 = 1 - x
3x = 2
x = 2/3.

How To Solve Exponential Equations of Different bases?

When an exponential equation has different bases on both sides, apply log on both sides and solve for the variable. Here is an example, 4^{x - 5} = 8. Taking log on both sides,
log 4^{x - 5} = log 8
(x - 5) log 4 = log 8
x - 5 = (log 8) / (log 4)
x = [(log 8) / (log 4)] + 5.

How To Solve Exponential Equations Using Logarithms?

We solve exponential equations using logarithms in two ways.

• Convert the exponential equation into logarithmic equation using b^x = a ⇔ log_ba = x.
• Apply "log" or "ln" on both sides and solve.