Additive Identity Vs Multiplicative Identity

There are many arithmetical operations that we perform in our everyday life. When a specific operation is performed on a number the output of that operation depends on the identity applied to the given numbers. According to algebra, every mathematical operation is associated with some major identities. Additive identity and multiplicative identity are the two basic algebraic identities. For rational numbers, natural numbers, whole numbers, and integers zero is the additive identity and 1 is the multiplicative identity.

In this article, we will learn about what is additive identity, what is multiplicative identity, and the difference between additive identity and multiplicative identity along with interesting solved examples on additive identity Vs multiplicative identity.

The word additive itself gives the impression that this identity is applied while performing the operation of addition. The property of additive identity states that if a number is added to zero it will give the number itself as a resultant. Here, zero is known as the additive identity. This condition is verified and is true for all complex numbers, imaginary numbers, integers, rational numbers, and real numbers as well. Let us look at the practical approach for the same. Below is an example to verify the additive identity. Say p is any real number, then its additive identity property will be given as: p + 0 = p = 0 + p. Here 0 is an identity element.

For example, 1110 + 0 = 1110. This example is an illustration of additive identity. This simply proved when 0 is added to any real number, the output is the same as the real number.

Additive Identity for Integers

Additive identity for integers says that if an integer is added to zero it will give the integer itself as a resultant. Let us look at some examples illustrating the additive identity for integers.

72 + 0= 0+ 72 = 72 (Here, 72 is a positive integer)
-72 + 0 = 0 + (- 72) = -72 (Here, -72 is a negative integer)

The multiplicative identity property is applied while performing the operation of multiplication. It states that if a number is multiplied by 1 the resultant will be the number itself. “1” is the multiplicative identity of a number. It is represented as p × 1 = p = 1 × p. Let us understand the relation with help of a real number example. Here let say p = -3. Putting the value of p in p × 1 = p = 1 × p, we get
−3 × 1 = −3
Here, if we take -1 then, (−1) × (−1) = 1 (Note that −1 is not a multiplicative identity)

Let us look at other examples, 7 × 1 = 7, -7 × 1 = -7, 1/7 × 1 = 1/7. Multiplying number by 1 leaves it unchanged.

The multiplicative identity property states that when any number is multiplied by 1, the product is equal to the original number. This implies when a number is multiplied by 1, we have just one copy of a number so the resultant is equal to the same number. The multiplicative identity property can be mathematically stated as a × 1 = a = 1 × a.

The below table shows the difference between additive identity and multiplicative identity.

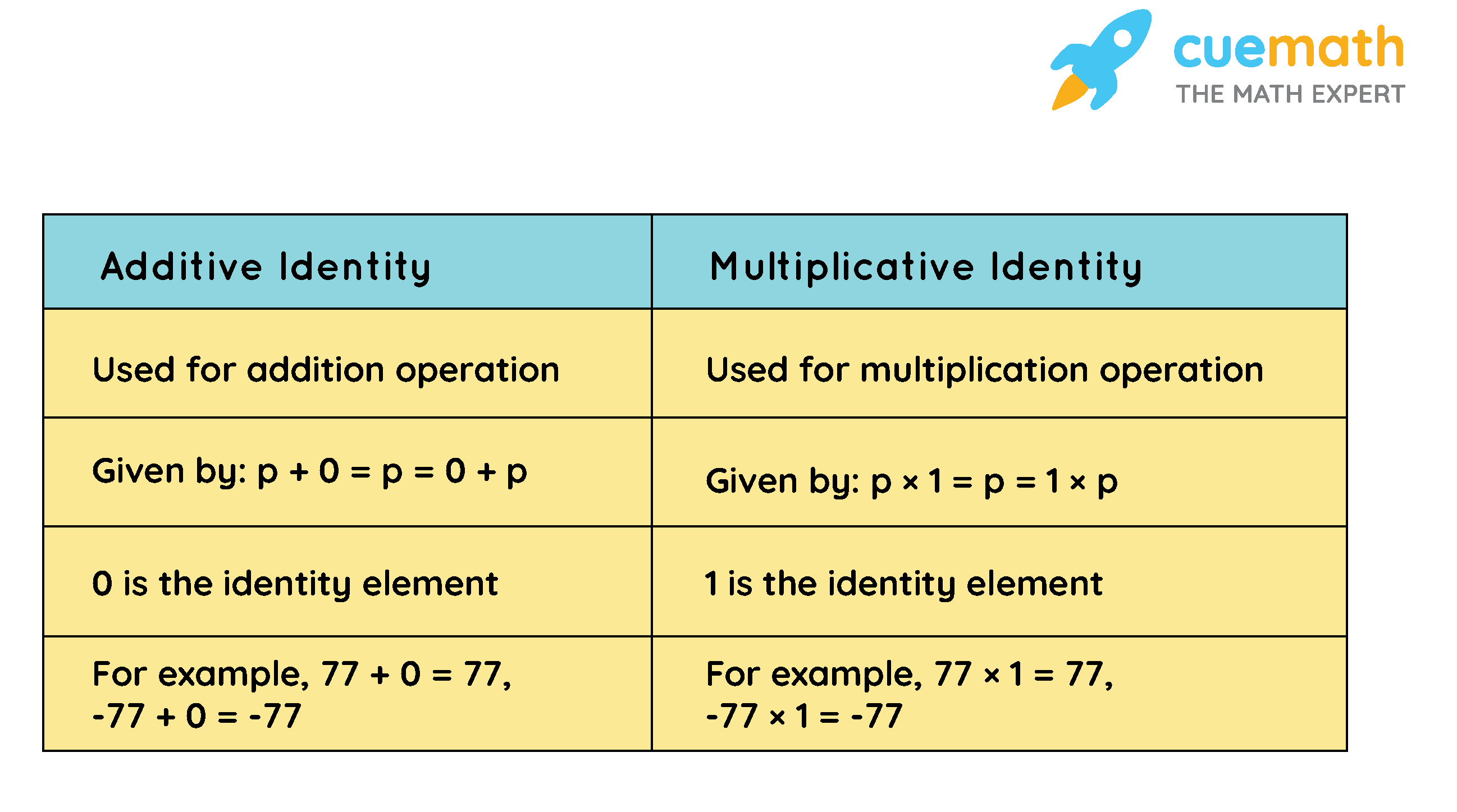
Important Notes on Additive Identity and Multiplicative Identity

• The additive identity is 0 and the multiplicative identity is 1.
• For a real number x, x + 0 = x = 0 + x and x × 1 = x = 1 × x.
• −1 is not a multiplicative identity.

Related Articles

• Multiplicative Inverse
• Multiplicative Inverse Calculator
• The Multiplicative Inverse of 1/4
• Additive Inverse
• Additive Inverse calculator

What is Multiplicative Identity Formula?

The formula for multiplicative identity is written as x × 1 = x = 1 × x, where x is a real number.

What do you Mean by Additive Identity?

Additive identity states that if a number is added to zero it will give the number itself as a resultant. Say x is any real number, then its additive identity will be given as: x + 0 = x = 0 + x. For example, 27 + 0 = 27 = 0 + 27.

What is Multiplicative Identity Property?

Multiplicative identity states that if a number is multiplied to 1 the resultant will be the number itself. “1” is the multiplicative identity of a number and is represented as: p × 1 = p = 1 × p. For example, 27 × 1 = 27 = 1 × 27.

What is the Difference Between Additive Identity and Multiplicative Identity?

Additive identity is used for addition operations whereas multiplicative identity is used for the multiplication operations.
Additive identity is represented as x + 0 = x = 0 + x.
Multiplicative identity is represented as p × 1 = p = 1 × p.

Can you List the Example of Additive Identity for Integers?

Say z is any integer, then its additive identity will be given as: z + 0 = z = 0 + z. For example, 29 + 0 = 29 = 0 + 29. Similarly, -29 + 0 = 0 + (-29).

Can you List the Example of Multiplicative Identity for Real Numbers?

Say z is any real number, then its multiplicative identity will be given as: z × 1 = z = 1 × z. For example, 29 × 1 = 29 = 1 × 29.

What is the Identity Element in Additive Identity and Multiplicative Identity?

• 0 is the identity element in additive identity.
• 1 is the identity element in the multiplicative identity.