Negative Numbers
A negative number is a number whose value is always less than zero and it has a minus (-) sign before it. On a number line, negative numbers are represented on the left side of zero. For example, -6 and -15 are negative numbers. Let us learn more about negative numbers in this lesson.
| 1. | What are Negative Numbers? |
| 2. | Rules for Negative Numbers |
| 3. | Adding and Subtracting Negative Numbers |
| 4. | Multiplication and Division of Negative Numbers |
| 5. | FAQs on Negative Numbers |
What are Negative Numbers?
Negative numbers are numbers that have a minus sign as a prefix. They can be integers, decimals, or fractions. For example, -4, -15, -4/5, -0.5 are termed as negative numbers. Observe the figure given below which shows how negative numbers are placed on a number line.

Negative Integers
Negative integers are numbers that have a value less than zero. They do not include fractions or decimals. For example, -7, -10 are negative integers.
Rules for Negative Numbers
When the basic operations of addition, subtraction, multiplication, and division are performed on negative numbers, they follow a certain set of rules.
- The sum of two negative numbers is a negative number. For example, -5 + (-1) = -6
- The sum of a positive number and a negative number is the difference between two numbers. The sign of the bigger absolute value is placed before the result. For example, -9 + 3 = -6
- The product of a negative number and a positive number is a negative number. For example, -9 × 2 = -18
- The product of two negative numbers is a positive number. For example, -6 × -3 =18
- While dividing negative numbers, if the signs are the same, the result is positive. For example, -56 ÷ -7 = 8
- While dividing negative numbers, if the signs are different, the result is negative. For example, -32 ÷ 4 = -8
Adding and Subtracting Negative Numbers
For adding and subtracting negative numbers, we need to remember the following rules.
Addition of Negative Numbers
Case 1: When a negative number is added to a negative number, we add the numbers and use the negative sign in the answer. For example, -7 + (- 4) = -7 - 4 = -11. In other words, the sum of two negative numbers always results in a negative number.
This can be understood with the help of a number line. The number line rule says, "To add a negative number we move to the left on the number line". Therefore, observe the following number line, and apply the rule on -7 + (- 4). We can see that when we start from -7 and move 4 numbers to the left, it brings us to -11.
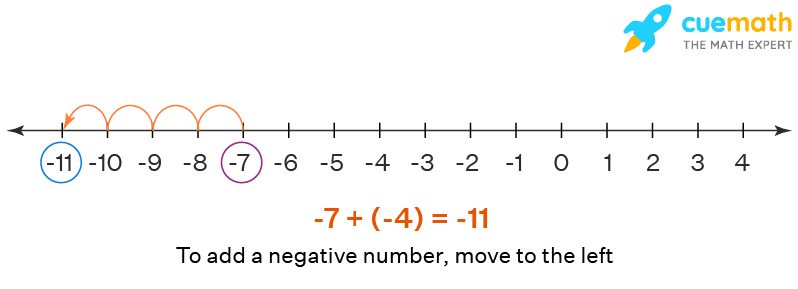
Case 2: When a positive number is added to a negative number, we find their difference and use the sign of the larger absolute value in the answer. For example, -9 + (5) ⇒ - 4. Since we are using the sign of the greater absolute value, the answer is -4.
This can be understood better with the help of a number line. The number line rule says, "To add a positive number we move to the right on the number line". Observe the following number line and apply the rule on -9 + (+5). We start from -9 and move 5 numbers to the right that brings us to -4.

Subtraction of Negative Numbers
The subtraction of negative numbers is similar to addition. We just need to remember a rule which says:
Rule of Subtraction: Change the operation from subtraction to addition, and change the sign of the second number that follows.
Case 1: When we need to subtract a positive number from a positive number, we follow the subtraction rule given above. For example, 5 - (+6) becomes 5 + (-6) = 5 - 6 = -1.
Now, if we apply the rule of the number line on 5 + (-6), to add a negative number, we move to the left. Therefore, we start with 5 and move 6 numbers to the left, which brings us to -1.

Case 2: When we need to subtract a positive number from a negative number, we will follow the same rule of subtraction which says:
Rule of Subtraction: Change the operation from subtraction to addition, and change the sign of the second number that follows.
For example, -3 - (+1), will become -3 + (-1). This can be simplified as -3 -1 = -4.
Now, if we apply the rule of the number line on -3 + (-1), to add a negative number we move to the left. Therefore, we start with -3 and move 1 number to the left, which brings us to -4.

Case 3: When we need to subtract a negative number from a negative number, we will follow the rule of subtraction:
Rule of Subtraction: Change the operation from subtraction to addition, and change the sign of the second number that follows.
For example, -9 - (-12) ⇒ -9 + 12 = 3. Here, 12 becomes positive. We use the sign of the bigger absolute value that is 12 and the answer is 3.
Multiplication and Division of Negative Numbers
There are two basic rules related to the multiplication and division of negative numbers.
Multiplying Positive and Negative Numbers
- Rule 1: When the signs of the numbers are different, the result is negative. (-) × (+) = (-). In other words, when we multiply a negative number with a positive number, the product is always negative. For example, -3 × 6 = -18.
- Rule 2: When the signs of the numbers are the same, the result is positive. (-) × (-) = (+); (+) × (+) = (+). In other words, when we multiply two negative or two positive numbers, the product is always positive. For example, -3 × - 6 = 18.
Dividing Positive and Negative Numbers
- Rule 1: When we divide a negative number by a positive number, the result is always negative. (-) ÷ (+) = (-). For example, (-36) ÷ (4) = -9
- Rule 2: When we divide a negative number by a negative number, the result is always positive. (-) ÷ (-) = (+) For example, (-24) ÷ (-4) = 6
Negative Integers With Exponents
There are two basic rules related to negative integers with exponents:
- If a negative integer has an even number in the exponent, then the final product will always be a positive integer. For example, (-4)6 =(-4) × (-4) × (-4) × (-4) × (-4) × (-4) = +4096
- If a negative integer has an odd number in the exponent, then the final product will always be a negative integer. For example, -93 = -9 × -9 × -9 = -729
☛ Related Topics
Negative Numbers Examples
-
Example 1: Add the given negative numbers.
a.) -45 and -78
b.) -90 and -67
Solution:
a.) Since both -45 and -78 are negative numbers, we will add the negative integers and place a negative sign before the sum.
45 + 78 = 123
Now, we will place a minus sign before the sum. Thus, the answer is -123.
b.) To add -90 and -67, we will add the negative numbers and place a negative sign before the sum.
90 + 67 = 157
Now, we will place a minus sign before the sum. Thus, the answer is -157.
-
Example 2: Subtract the given negative integers: Subtract -5 from -8
Solution:
When we need to subtract a negative number from a negative number, we will follow the rule of subtraction, 'Change the operation from subtraction to addition, and change the sign of the second number that follows.'
In this case, -8 - (-5) ⇒ -8 + 5 = -3.
-
Example 3: Simplify the negative integers:
a.) (-3) × (-2)
b.) -24 ÷ -3
Solution:
a.) To multiply (-3) × (-2), we will multiply the given negative numbers and the sign of the product will be positive. Therefore, in this case, the product of (-3) × (-2) = 6
b.) In order to divide the negative numbers, (-24) ÷ (-3), we will divide them and the sign of the answer will be positive. In this case, (-24) ÷ (-3) = 8

FAQs on Negative Numbers
What are Negative Numbers in Math?
A negative number is a number whose value is always less than zero and it has a minus (-) sign before it. On a number line, negative numbers are shown to the left of zero. For example, - 2, - 3, - 4, - 5 are called negative numbers.
What are the Rules for Negative Numbers?
When the basic operations of addition, subtraction, multiplication, and division are performed on negative numbers, they follow a certain set of rules.
- The sum of two negative numbers is a negative number. For example, -3 + (-1) = -4
- The sum of a positive number and a negative number is the difference between the two numbers. The sign of the bigger absolute value is placed before the result. For example, -6 + 3 = -3
- The product of a negative number and a positive number is always a negative number. For example, -5 × 2 = -10
- The product of two negative numbers is a positive number. For example, -5 × -3 =15
- While dividing negative numbers, if the signs are the same, the result is positive. For example, (-28) ÷ (-7) = 4
- While dividing negative numbers, if the signs are different, the result is negative. For example, (-21) ÷ (3) = -7
What is the Sum of Two Negative Numbers?
The sum of two negative numbers is always a negative number. For example, (-7) + (-2) = -9
What are Negative Numbers used for?
There are situations in real life where we use numbers that are less than zero. Negative numbers are used to measure temperature. For example, the lowest possible temperature is absolute zero which is expressed as -273.15°C on the Celsius scale, and -459.67°F on the Fahrenheit scale. Negative numbers are also used to measure the geographical locations that are below the sea level and which are expressed in negative integers like -100 ft Mean Sea Level.
How to Multiply Negative Numbers?
There are two basic rules related to the multiplication of negative numbers.
- Rule 1: When the signs of the numbers are different, the result is negative. In other words, when we multiply a negative number with a positive number, the product is always negative. For example, -2 × 6 = -12.
- Rule 2: When the signs of the numbers are the same, the result is positive. In other words, when we multiply two negative or two positive numbers, the product is always positive. For example, -4 × - 6 = 24.
How to Divide Negative Numbers?
The rules that are applied for the multiplication of numbers are also used in the division of negative numbers.
- Rule 1: When the signs of the numbers are different, the result is negative. In other words, when we divide a negative number with a positive number, the answer is always negative. For example, -12 ÷ 3 = -4.
- Rule 2: When the signs of the numbers are the same, the result is positive. In other words, when we divide two negative numbers or two positive numbers, the answer is always positive. For example, -14 ÷ - 2 = 7.
What is the Difference Between Negative Integers and Positive Integers?
The main difference between negative integers and positive integers is that negative integers have a value less than zero and positive integers have a value greater than zero. It should be noted that zero is neither a positive integer nor a negative integer.
How do you Add Two Negative Integers?
Adding two negative integers together is easy because we just add the given numbers and then place a negative sign in front of the sum. For example, (-2) + (-5) = -7
What are the Rules For Subtracting Negative Numbers?
There is a basic rule for subtracting negative numbers. "Change the operation from subtraction to addition, and change the sign of the second number that follows". For example, let us subtract -2 - (-5). In this case, we change the operation from subtraction to addition and change the sign of (-5) to (+5). This makes it -2 + (+5) = -2 + 5 = 3.
How to Subtract Negative Numbers?
When we subtract negative numbers, we just need to remember a rule which says: Change the operation from subtraction to addition, and change the sign of the second number that follows. Now, let us apply this rule, for example, subtract 5 from -8. This means -8 - (5). After applying the rule, -8 - (5) becomes -8 + (-5) = -13.
visual curriculum