Integration of Cos 3x
Integration of cos 3x is the process of determining the integral of cos 3x using different methods. The integration of cos 3x is one-third of sine of angle 3x, that is, it is given by (1/3) sin 3x + C, where C is the constant of integration. Integration of cos 3x is the inverse process of differentiation of cos 3x and hence is also called the anti-derivative of cos 3x.
In this article, we will calculate the integral of cos 3x using the substitution method and cos 3x formula. We will also calculate the definite integral of cos 3x using the formula for integration of cos 3x.
What is Integration of Cos 3x?
Cos 3x is an important formula in trigonometry and can be used to determine the integration of cos 3x. We know that the derivative of sin ax is 'a cos ax', therefore, the integral of cos ax is given by (1/a) sin ax + C (as the derivative of C is zero). Using this formula, we have the integration of cos 3x as ∫cos 3x dx = (1/3) sin 3x + C, where dx denotes that the integration of cos 3x is with respect to x, ∫ is the symbol for the integration of cos 3x and C is the constant of integration.
Integration of Cos 3x Formula
Mathematically, we can write the integral of cos 3x using symbols. The formula for the integral of cos 3x is ∫cos 3x dx = (1/3) sin 3x + C, where C is the constant of integration.
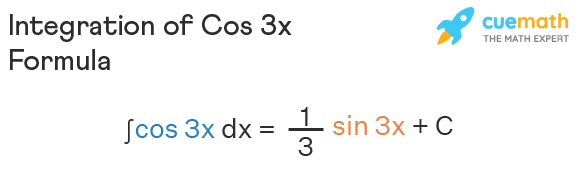
Integration of Cos 3x Proof Using Cos 3x Formula
Cos 3x is an important formula in trigonometry given by cos 3x = 4 cos3x - 3 cos x. Now, we will use this formula to calculate the integration of cos 3x. But before this, we will evaluate the integral of cos cube x, that is, determine the value of ∫cos3x dx. To determine this integral, we will use trigonometric formulas and identities.
∫cos3x dx = ∫cos x . cos2x dx
= ∫cos x (1 - sin2x) dx [Because sin2x + cos2x = 1 ⇒ cos2x = 1 - sin2x]
= ∫cos x dx - ∫cos x sin2x dx
= sin x + C1 - I1, where I1 = ∫cos x sin2x dx
Assume sin x = u ⇒ cos x dx = du. Substitute these values in I1
I1 = ∫cos x sin2x dx
= ∫u2 du
= u3/3 + C2
= (1/3) sin3x + C2
Hence, we have ∫cos3x dx = sin x + C1 - (1/3) sin3x - C2 = sin x - (1/3) sin3x + C, where C = C1 - C2
Now, we will determine the integral of cos 3x using the cos 3x formula.
∫cos 3x dx = ∫(4 cos3x - 3 cos x) dx
= 4 ∫cos3x dx - 3 ∫cos x dx
= 4 [sin x - (1/3) sin3x + C] - 3 sin x + C3
= 4 sin x - (4/3)sin3x + 4C - 3 sin x + C3
= sin x - (4/3)sin3x + 4C + C3
= (1/3)(3 sin x - 4 sin3x) + 4C + C3
= (1/3)sin 3x + K, where K = 4C + C3 is the constant of integration [Because sin 3x = 3 sin x - 4 sin3x]
Hence, we have obtained the integral of cos 3x as ∫cos 3x dx = (1/3) sin 3x + K
Integration of Cos 3x Using Substitution Method
Now, we will use the substitution method to prove that the integration of cos 3x is given by ∫cos 3x dx = (1/3) sin 3x + C. For this, assume 3x = v ⇒ 3dx = dv ⇒ dx = (1/3)dv. We will use different trigonometric formulas and identities to derive the integral of cos 3x.
∫cos 3x dx = ∫cos v (1/3)dv
= (1/3) ∫cos v dv
= (1/3) (sin v + K) [Because Integral of cos x is sin x + C]
= (1/3) sin 3x + (1/3)K
= (1/3) sin 3x + C, where (1/3)K = C is the constant of integration.
Hence we have evaluated the integration of cos 3x using the substitution method.
Definite Integration of Cos 3x From 0 to Pi
Next, we will determine the value of the definite integral of cos 3x with limits from 0 to π. We know that the integral of cos 3x is (1/3) sin 3x + C. So, we have
\(\begin{align}\int_{0}^{\pi}\cos 3x \ dx &= \left [ \dfrac{1}{3}\sin 3x + C\right ]_0^{\pi}\\&=\left ( \dfrac{1}{3}\sin 3\pi + C\right )-\left ( \dfrac{1}{3}\sin 3(0) + C\right )\\&=0\end{align}\)
Therefore, the integration of cos 3x from 0 to π is equal to 0.
Important Points on Integration of Cos 3x
- The antiderivative of cos 3x is ∫cos 3x dx = (1/3) sin 3x + C, where C is the constant of integration.
- The integration of cos 3x can be determined using the substitution method and cos 3x formula.
Related Topics on Integration of Cos 3x
Integration of Cos 3x Examples
-
Example 1: Calculate the integral of x cos 3x
Solution: To evaluate the integration of x cos 3x, we will use integration by parts.
∫x cos 3x dx = x ∫cos 3x dx - ∫[dx/dx × ∫cos 3x dx]dx
= x(1/3) sin 3x - ∫(1/3) sin 3x dx
= (x/3) sin 3x - (1/3)(-1/3)cos 3x + C [Because ∫sin 3x = (-1/3) cos 3x + C]
= (x/3) sin 3x + (1/9)cos 3x + C
Answer: Hence Integral of x cos 3x is (x/3) sin 3x + (1/9)cos 3x + C
-
Example 2: Determine the integration of cos 3x cos x.
Solution: To determine the integration cos 3x cos x, we will use the formula cos a cos b = (1/2)[cos(a + b) + cos(a - b)]. Using this formula, we have
∫cos 3x cos x dx = ∫(1/2)[cos(3x + x) + cos(3x - x)] dx
= ∫(1/2)[cos 4x + cos 2x] dx
= (1/2) ∫(cos 4x + cos 2x) dx
= (1/2) [(sin 4x)/4 + (sin 2x)/2] + C
= (sin 4x)/8 + (sin 2x)/4 + C
Answer: The integration of cos 3x cos x is (sin 4x)/8 + (sin 2x)/4 + C.

FAQs on Integration of Cos 3x
What is Integration of Cos 3x in Trigonometry?
Integration of cos 3x is the process of determining the integral of cos 3x using different methods. The integration of cos 3x is given by ∫cos 3x dx = (1/3) sin 3x + C
What is Formula for Integration of Cos 3x?
The formula for the integral of cos 3x is ∫cos 3x dx = (1/3) sin 3x + C, where C is the constant of integration.
What is the Integral of Cos Cube x?
The integral of cos cube x is sin x - (1/3) sin3x + C.
What is the Integration of cos 3x cos 5x?
The integration of cos 3x cos 5x is (1/16) sin 8x + (1/4) sin 2x + C.
Determine the Integral of cos 3x sin 2x?
The integral of cos 3x sin 2x is (-1/10) cos 5x + (1/2) cos x + C.
How to Calculate the Integral of Cos 3x?
The integral of cos 3x can be calculated using the method of substitution and cos 3x formula.
How to Determine the Definite Integral of Cos 3x from 0 to π?
The integration of cos 3x from 0 to pi is equal to 0.
visual curriculum