Differentiation
The process of finding derivatives of a function is called differentiation in calculus. A derivative is the rate of change of a function with respect to another quantity. The laws of Differential Calculus were laid by Sir Isaac Newton. The principles of limits and derivatives are used in many disciplines of science. Differentiation and integration form the major concepts of calculus.
Let us learn the techniques of differentiation to find the derivatives of algebraic functions, trigonometric functions, and exponential functions.
What is Differentiation?
Differentiation means the rate of change of one quantity with respect to another. The speed is calculated as the rate of change of distance with respect to time. This speed at each instant is not the same as the average calculated. Speed is the same as the slope, which is nothing but the instantaneous rate of change of the distance over a period of time.
The ratio of a small change in one quantity with a small change in another which is dependent on the first quantity is called differentiation. One of the important concepts in calculus is mainly focused on the differentiation of a function. The maximum or minimum value of a function, the velocity and acceleration of moving objects, and the tangent of a curve are determined by differentiation. If y = f(x) that is differentiable, then the differentiation is represented as f'(x) or dy/dx.
Definition of Derivatives
The geometrical meaning of the derivative of y = f(x) is the slope of the tangent to the curve y = f(x) at ( x, f(x)). The first principle of differentiation is to compute the derivative of the function using the limits. Let a function of a curve be y = f(x). Let us take a point P with coordinates(x, f(x)) on a curve. Take another point Q with coordinates (x+h, f(x+h)) on the curve. Now PQ is the secant to the curve. The slope of a curve at a point is the slope of the tangent line at that point. We know, slope of the secant line is \(\dfrac{y_2 -y_1}{x_2- x_1}\).

Thus, in this case, it is \(\dfrac{f(x+h)-f(x)}{(x+h)-x} = \dfrac{f(x+h)-f(x)}{h}\)
We want h to be as small as possible to get the slope of the tangent. We have y = f(x). There is an incremental change in x, denoted as Δx. Then there exists an incremental change in y, denoted as Δy.
Then y + Δy = f(x + Δx)
f(x) + Δy = f(x + Δx)
Δy = f(x + Δx) - f(x)
Dividing by Δx on both the sides,
\(\dfrac{dy}{dx}= \dfrac{f(x + Δx) - f(x)}{Δx}\)
As the change is so small, applying the limits, we get
\(\dfrac{dy}{dx}= \mathop {\lim }\limits_{𝛿x \to 0}\dfrac{𝛿y}{𝛿x}\\\\ =\mathop {\lim }\limits_{𝛿x \to 0}\dfrac{f(x + 𝛿x) - f(x)}{𝛿x}\)
where, d/dx is the differential coefficient, and it is known as the Leibnitz symbol.
\(\mathop {\lim }\limits_{h \to 0} \dfrac{f(x+h)-f(x)}{h}\), if the limit exists, f'(x) is the first derivative of f(x). This derivative of f(x) at a quantifies the change in f(x) with respect to x. This process of computing the derivative of a function is called differentiation.
Thus definition of derivative is as follows: If f is a real-valued function of a real variable defined on an open interval I and if y = f(x) is a differentiable function of x, then dy/dx = f'(x) = \(\mathop {\lim }\limits_{Δx \to 0} \dfrac{f(x+Δx)-f(x)}{Δx}\).
Differentiation Formula
The derivatives of the functions are found using the derivative formula as derived in the previous section. The derivatives of elementary functions are remembered as differentiation formulas.
Consider a function y = xn , n > 0. Then f(x + Δx) = (x + Δx)n and f(x + Δx)-f(x) = (x + Δx)n - xn
\(\dfrac{f(x+\delta x)-f(x)}{\delta x} = \mathop {\lim }\limits_{\delta x \to 0}\dfrac{(x + \delta x)^n - x^n}{(x + \delta x)-x}\\\\ = \mathop {\lim }\limits_{y \to x} \dfrac{y^n - x^n}{y-x} = nx^{n-1}\),
where y = x + Δx and y → x as Δx → 0.
Similarly, we can derive the derivatives of other algebraic, exponential, and trigonometric functions using the fundamental principles of differentiation.
Differentiation of Elementary Functions
- The derivative of a constant function is 0. if y = k, where k is a constant, then y' = 0
- The derivative of a power function: If y = xn , n > 0. Then y' = n x n-1
- The derivative of logarthmic functions: If y = ln x, then y' = 1/x and if y = log\(_a\) x, then y' = 1/[(log a) x]
- The derivative of an exponential function: If y = a x , y = ax log a
Differentiation of Trigonometric Functions
Here are the derivatives of trigonometric functions.
- If y = sin x, y' = cos x
- If y = cos x, y' = -sin x
- If y = tan x, y' = sec2 x
- If y = sec x, y' = sec x tan x
- If y = cosec x, y' = -cosec x cot x
- If y = cot x, y' = -cosec2 x
Differentiation of Inverse Trigonometric Functions
Here are the derivatives of inverse trigonometric functions.
- If y = sin-1 x, y' = \(\dfrac{1}{\sqrt{(1-x^2)}}\)
- If y = cos-1 x, y' = \(-\dfrac{1}{\sqrt{(1-x^2)}}\)
- If y = tan-1 x, y' = \(\dfrac{1}{(1+x^2)}\)
- If y = cot-1 x, y' =\(-\dfrac{1}{(1+x^2)}\)
- If y = sec-1 x, y' = \(\dfrac{1}{|x| \sqrt{(x^2 -1)}}\)
- If y = cosec-1 x, y' = \(-\dfrac{1}{|x| \sqrt{(x^2 -1)}}\)
Rules of Differentiation
If f is differentiable at a point x = \(x_0\), then f is continuous at \(x_0\). A function is differentiable in an interval [a,b] if it is differentiable at every point [a,b]. The sum, difference, product, and composite of differentiable functions, wherever they are defined, are differentiable, and the quotient of two differentiable functions is differentiable, wherever it is defined. The differentiation rules are listed as follows:
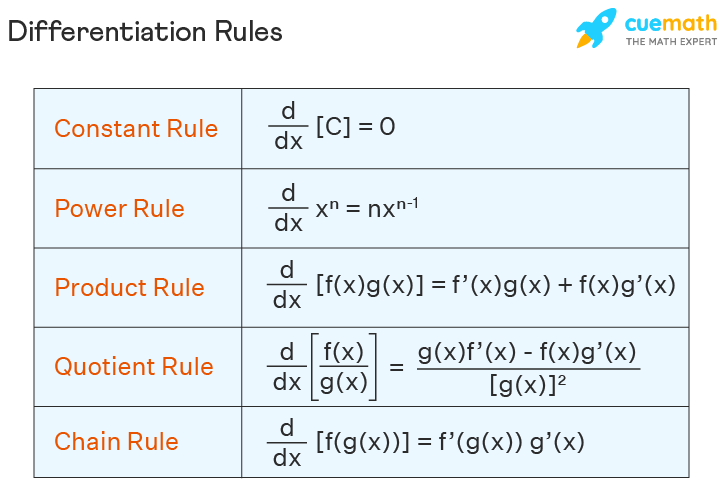
- Sum Rule: If y = u(x) ± v(x), then dy/dx = du/dx ± dv/dx.
- Product Rule: If y = u(x) × v(x), then dy/dx = u.dv/dx + v.du/dx
- Quotient Rule: If y = u(x) ÷ v(x), then dy/dx = (v.du/dx- u.dv/dx)/ v2
- Chain Rule: Let y = f(u) be a function of u and if u=g(x) so that y = f(g(x), then d/dx(f(g(x))= f'(g(x))g'(x)
- Constant Rule: y = k f(x), k ≠ 0, then d/dx(k(f(x)) = k d/dx f(x).
Differentiation of Special Functions
If x= f(t), y = g(t), where t is parameter, then we apply differentiation of parametric functions.
\(\dfrac{dy}{dx} = \dfrac{\dfrac{dy}{dt}}{\dfrac{dx}{dt}} = \dfrac{\dfrac{d .g(t)}{dt}}{\dfrac{d.f(t)}{dt}} = \dfrac{g'(t)}{f'(t)}\)
If y = f(x) and z = g(x). then the differentiation of y with respect to z is given as:
\(\dfrac{dy}{dz} = \dfrac{\dfrac{dy}{dx}}{\dfrac{dz}{dx}} = \dfrac{f'(x)}{g'(x)}\)
Implicit Differentiation
Let f(x,y) be a function in the form of x and y. If we cannot solve for y directly, we use implicit differentiation. Suppose f(x,y) = 0 (which is known as an implicit function), then differentiate this function with respect to x and collect the terms containing dy/dx at one side and then find dy/dx.
For example, let us find dy/dx if x2 +y2 =1.
We differentiate both sides of the equation.
d/dx. x2 + d/dx. y2 = d/dx.1
2x + 2y.dy/dx = 0
dy/ dx = -x/y
Logarithmic Differentiation Functions
If a function is the product and quotient of functions, as in y = \(\dfrac{f_1(x). f_2(x)..... }{g_1(x). g_2(x)....}\) we first take the logarithm and then differentiate it. If a function is in the form of an exponent of a function over another, as in [f(x)] g(x) then we take the logarithm of the function f(x) (to base e) and then differentiate it. This process is known as logarithmic differentiation.
For example, if y = xx , then log y = x log x
1/y. dy/dx = log x + 1
dy/dx = y. (logx + 1)
= xx (logx + 1)
Higher-Order Differentiation
We find higher-order derivatives on successive differentiation. The further differentiation of the first derivative is denoted by f'' or \(\dfrac{d^2y}{dx^2}\) and the third derivative is denoted by f'" or \(\dfrac{d^3y}{dx^3}\). The nth derivative of f(x) is f n(x) is used in the power series. For example, the rate of change of displacement is the velocity. The second derivative of displacement is the acceleration and the third derivative is called the jerk.
Consider a function y = f(x) = x5 - 3x4 + x
f1(x) = 5x4 - 12x3 + 1
f2(x) = 20x3 - 36 x2
f3(x) = 60x2 - 72 x
f4(x) = 120x -72
Partial Differentiation
The partial differential coefficient of f(x,y) with respect to x is the ordinary differential coefficient of f(x,y) when y is regarded as a constant. It is written as 𝛿y/ 𝛿x. For example, if z = f(x,y) = x4 + y4+3xy2 +x2y +x + 2y, then we consider y as constant to find 𝛿f/ 𝛿x and consider x as constant to find 𝛿f/ 𝛿y. Thus we find the partial derivatives of the function.
𝛿f/ 𝛿x = 4x3 +3y2 +2xy +1
𝛿f/ 𝛿y = 4y3 + 6xy + x2 + 2
If f(x,y) is a function of two variables such that 𝛿f/ 𝛿x and 𝛿f/ 𝛿y both exist. Then we have the partial derivatives as follows.
𝛿f/ 𝛿x wrt x = 𝛿2 f/ 𝛿x2 or \(f_{xx}\)
𝛿f/ 𝛿y wrt y = 𝛿2 f/ 𝛿y2 or \(f_{yy}\)
𝛿f/ 𝛿x wrt x = 𝛿2 f/ 𝛿x𝛿y or \(f_{xy}\)
𝛿f/ 𝛿y wrt y= 𝛿2 f/ 𝛿x𝛿y or \(f_{yx}\)
Important Notes
- Differentiation of a function is finding the rate of change of the function with respect to another quantity. \(f'(x) = \mathop {\lim }\limits_{Δx \to 0} \dfrac{f(x+Δx)-f(x)}{Δx}\), where Δx is the incremental change in x.
- The process of finding the derivatives of the function, if the limit exists, is called differentiation. The derivative of a function is given as dy/dx or y' or f'(x).
- Differentiability implies continuity, but its converse is not true.
☛ Also Check:
Examples of Differentiation
-
Example 1: Find the differentiation of y = x3 + 5 x2 + 3x + 7.
Solution:
Given y = x3 + 5 x2 + 3x + 7
We differentiate y with respect to x.Using the differentiation formula of power rule, we get dy/dx = dy/dx( x3 + 5 x2+ 3x + 7)
= d(x3)/dx + d(5 x2 )/dx + d(3x)/dx + d(7)/dx
dy/dx = 3 x2 + 5(2x) + 3 dy/dx + 0
= 3 x2 + 10 x + 3
Answer: dy/dx = 3 x2 + 10 x + 3
-
Example 2: Find the differentiation of y = cos(tan x)
Solution:
Given: y = cos(tan x)
We differentiate y with respect to x.Let u = tan x
y = cos u
Using the chain rule of differentiation,
dy/dx = dy/du . du/dx
dy/dx = d(cos u)/du . d(tan x)/dx
= -sin u . sec2 x
= -sin (tan x) . sec2 x
Answer: dy/dx = -sin (tan x) . sec2 x
-
Example 3.: Compute the dativerive of \(\dfrac{x}{1 + \tan x}\) using the differentiation rule.
Solution:
Given y = \(\dfrac{x}{1 + \tan x}\)
We differentiate y with respect to x.This is of the form y' = u(x)/v(x)
Using the quotient rule of differentiation, we know that
d/dx(u/v) = (v.u'- u.v')/ v2
v = 1 + tan x and u = x
v' = sec2x and u' = 1
\(\dfrac{d}{dx}(\dfrac{x}{1 + \tan x})\\=\dfrac{(1+tan x) -(x sec^2 x)}{(1+tan x)^2}\\=\dfrac{1+ tan x - x sec^2x}{(1+tan x)^2}\)
Answer: The derivative of y is \(\dfrac{1+ tan x - x sec^2x}{(1+tan x)^2}\)

FAQs on Differentiation
What is the Meaning of Differentiation?
The instantaneous rate of change of a function with respect to another quantity is called differentiation. For example, speed is the rate of change of displacement at a certain time. If y = f(x) is a differentiable function of x, then dy/dx = f'(x) = \(\mathop {\lim }\limits_{Δx \to 0} \dfrac{f(x+Δx)-f(x)}{Δx}\).
How Do You Perform Differentiation in Math?
Differentiation is done by applying the techniques of known differentiation formulas and differentiation rules in finding the derivative of a given function.
What Are The Basics of Differentiation?
The process of finding the derivative of a function is called differentiation. The three basic derivatives are differentiating the algebraic functions, the trigonometric functions, and the exponential functions.
Give an Example of Differentiation in Calculus.
The rate of change of displacement with respect to time is the velocity. This is an example of differentiation. Velocity is the first derivative of displacement. Acceleration is the second derivative of displacement.
What Are Differentiation Formulas?
The differentiation formula is used to find the derivative or rate of change of a function. if y = f(x), then the derivative dy/dx = f'(x) = \(\mathop {\lim }\limits_{Δx \to 0} \dfrac{f(x+Δx)-f(x)}{Δx}\).
How Do You Use Differentiation Formula?
The derivative of a function is found by applying limits to the function as per the first principle of differentiation. The derivative f'(x) = \(\mathop {\lim }\limits_{Δx \to 0} \dfrac{f(x+Δx)-f(x)}{Δx}\). For example, let us compute the derivative of sin x. f(x) = sin x.
F(x+Δx) = sin(x +Δx).
f(x+Δx) - f(x) = sin(x +Δx)- sinx = 2 sin(Δx/2) cos (x + Δx/2)
\(\dfrac{f(x+Δx)-f(x)}{Δx}\\ = \dfrac{sin \dfrac{Δx}{2}}{\dfrac{Δx}{2}} cos (x + \dfrac{Δx}{2})\\ ⇒\mathop {\lim }\limits_{Δx \to 0}\dfrac{sin \dfrac{Δx}{2}}{\dfrac{Δx}{2}} cos (x + \dfrac{Δx}{2})\\= 1\times cos x\\= cos x \)[Since cos x is continuous and \(\mathop {\lim }\limits_{Δx \to 0}cos(x+Δx) = cos x\)]
What Are The Differentiation Rules in Calculus?
There are different rules followed in differentiating a function. The differentiation rules are power rule, chain rule, quotient rule, and the constant rule.
- Sum Rule: If y = u(x) ± v(x), then dy/dx = du/dx ± dv/dx.
- Product Rule: If y = u(x) × v(x), then dy/dx = u.dv/dx + v.du/dx
- Quotient Rule: If y = u(x) ÷ v(x), then dy/dx = (v.du/dx- u.dv/dx)/ v2
- Chain Rule: Let y = f(u) be a function of u and if u=g(x) so that y = f(g(x), then d/dx(f(g(x))= f'(g(x))g'(x)
- Constant Rule: y = k f(x), k ≠ 0, then d/dx(k(f(x)) = k d/dx f(x).
What Are The Applications of Differentiation Formulas?
We use the differentiation formulas to find the maximum or minimum values of a function, the velocity and acceleration of moving objects, and the tangent of a curve. To know more applications of differentiation, click here.
What is The Differentiation of a Constant?
The differentiation of a constant is 0 as per the power rule of differentiation.
visual curriculum